User:
Particle in electric field of an infinite plate
Concept 
According to Gauss's Law, the variables the surface where the electric field is constant ($dS$), the charge ($Q$), the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$), the versor normal to the section ($\hat{n}$), and the electric field ($\vec{E}$) satisfy the following equation:
| $\displaystyle\int_S\vec{E}\cdot\hat{n}\,dS=\displaystyle\frac{Q}{\epsilon_0\epsilon}$ |
In the case of a flat Gaussian surface, the field must be constant, so the relationship of the electric eield ($E$) with the surface of the conductor ($S$) is established as:
| $ E S = \displaystyle\frac{ Q }{ \epsilon_0 \epsilon }$ |
what is shown in the graph
Since the charge density by area ($\sigma$) is equally defined by:
| $ \sigma = \displaystyle\frac{ Q }{ S }$ |
For the electric field of an infinite plate ($E_s$), the resulting expression is:
| $ E_s =\displaystyle\frac{ \sigma }{ 2 \epsilon_0 \epsilon }$ |
ID:(11841, 0)
Particle in electric potential of an infinite plate
Concept
The reference electrical, two infinity plates ($\varphi_d$) is with the electric field, two infinite plates ($E_d$) and the position on the z axis ($z$) is equal to:
| $ \varphi_d = -\displaystyle\int_0^z du\,E_d$ |
the electric field of an infinite plate ($E_s$) is with the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$) and the charge density by area ($\sigma$) is equal to:
| $ E_s =\displaystyle\frac{ \sigma }{ 2 \epsilon_0 \epsilon }$ |
the electric potential, infinite plate ($\varphi_s$) is with and the position on the z axis ($z$) turns out
| $ \varphi_s = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z $ |
As illustrated in the following graph:
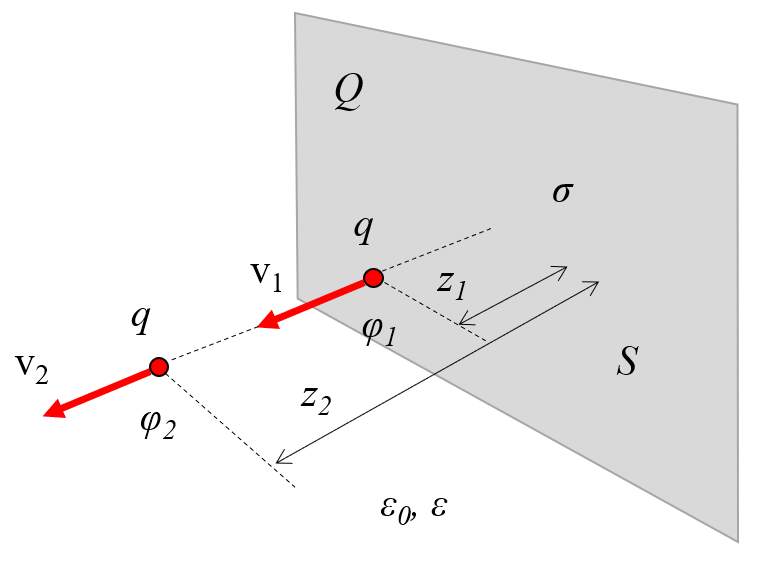
the field at two points must have the same energy. Therefore, the variables the charge ($Q$), the particle mass ($m$), the speed 1 ($v_1$), the speed 2 ($v_2$), and the electric potential 1 ($\varphi_1$) according to the equation:
| $ \varphi_1 = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z_1 $ |
and the electric potential 2 ($\varphi_2$), according to the equation:
| $ \varphi_2 = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z_2 $ |
must satisfy the following relationship:
| $ \displaystyle\frac{1}{2} m v_1 ^2 + q \varphi_1 = \displaystyle\frac{1}{2} m v_2 ^2 + q \varphi_2 $ |
ID:(11852, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ E_s =\displaystyle\frac{ \sigma }{ 2 \epsilon_0 \epsilon }$
E_s = sigma /(2 * epsilon_0 * epsilon )
$ \displaystyle\frac{1}{2} m v_1 ^2 + q \varphi_1 = \displaystyle\frac{1}{2} m v_2 ^2 + q \varphi_2 $
m * v_1 ^2/2 + q * phi_1 = m * v_2 ^2/2 + q * phi_2
$ \varphi_1 = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z_1 $
phi_s = - sigma * z /( epsilon * epsilon_0 )
$ \varphi_2 = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z_2 $
phi_s = - sigma * z /( epsilon * epsilon_0 )
$ \sigma = \displaystyle\frac{ Q }{ S }$
sigma = Q / S
ID:(15808, 0)
Surface charge density
Equation
The surface charge density is calculated by dividing the total charge by the surface area. Therefore, the relationship between the charge density by area ($\sigma$) and the charge ($Q$) with the surface of the conductor ($S$) is established as:
| $ \sigma = \displaystyle\frac{ Q }{ S }$ |
ID:(11460, 0)
Infinite plate
Equation
The electric field of an infinite plate ($E_s$) is with the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$) and the charge density by area ($\sigma$) equal to:
| $ E_s =\displaystyle\frac{ \sigma }{ 2 \epsilon_0 \epsilon }$ |
According to Gauss's Law, the variables the surface where the electric field is constant ($dS$), the charge ($Q$), the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$), the versor normal to the section ($\hat{n}$), and the electric field ($\vec{E}$) satisfy the following equation:
| $\displaystyle\int_S\vec{E}\cdot\hat{n}\,dS=\displaystyle\frac{Q}{\epsilon_0\epsilon}$ |
In the case of a flat Gaussian surface, the field must be constant, so the relationship of the electric eield ($E$) with the surface of the conductor ($S$) is established as:
| $ E S = \displaystyle\frac{ Q }{ \epsilon_0 \epsilon }$ |
Since the charge density by area ($\sigma$) is equally defined by:
| $ \sigma = \displaystyle\frac{ Q }{ S }$ |
For the electric field of an infinite plate ($E_s$), the resulting expression is:
| $ E_s =\displaystyle\frac{ \sigma }{ 2 \epsilon_0 \epsilon }$ |
ID:(11448, 0)
Electrical potential, surfaces (1)
Equation
The electric potential, infinite plate ($\varphi_s$) is with the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$), the charge density by area ($\sigma$) and the position on the z axis ($z$) is equal to:
| $ \varphi_1 = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z_1 $ |
| $ \varphi_s = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z $ |
In the case of an infinite plate, the relationship between the electric potential, infinite plate ($\varphi_s$), the electric field of an infinite plate ($E_s$), and the position on the z axis ($z$) is established by the following equation:
| $ \varphi_s = -\displaystyle\int_0^z du\,E_s$ |
Similarly, the relationship involving the electric field of an infinite plate ($E_s$), the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$), and the charge density by area ($\sigma$) is defined as:
| $ E_s =\displaystyle\frac{ \sigma }{ 2 \epsilon_0 \epsilon }$ |
In spherical coordinates, this is expressed as:
$\varphi_s = -\displaystyle\int_0^z du \displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon }= -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z$
Finally, the relationship that includes the electric potential, infinite plate ($\varphi_s$) and the position on the z axis ($z$) is determined by the following equation:
| $ \varphi_s = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z $ |
ID:(11586, 1)
Electrical potential, surfaces (2)
Equation
The electric potential, infinite plate ($\varphi_s$) is with the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$), the charge density by area ($\sigma$) and the position on the z axis ($z$) is equal to:
| $ \varphi_2 = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z_2 $ |
| $ \varphi_s = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z $ |
In the case of an infinite plate, the relationship between the electric potential, infinite plate ($\varphi_s$), the electric field of an infinite plate ($E_s$), and the position on the z axis ($z$) is established by the following equation:
| $ \varphi_s = -\displaystyle\int_0^z du\,E_s$ |
Similarly, the relationship involving the electric field of an infinite plate ($E_s$), the electric field constant ($\epsilon_0$), the dielectric constant ($\epsilon$), and the charge density by area ($\sigma$) is defined as:
| $ E_s =\displaystyle\frac{ \sigma }{ 2 \epsilon_0 \epsilon }$ |
In spherical coordinates, this is expressed as:
$\varphi_s = -\displaystyle\int_0^z du \displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon }= -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z$
Finally, the relationship that includes the electric potential, infinite plate ($\varphi_s$) and the position on the z axis ($z$) is determined by the following equation:
| $ \varphi_s = -\displaystyle\frac{ \sigma }{2 \epsilon_0 \epsilon } z $ |
ID:(11586, 2)
Energy of a particle
Equation
Electric potentials, which represent potential energy per unit of charge, influence how the velocity of a particle varies. Consequently, due to the conservation of energy between two points, it follows that in the presence of variables the charge ($q$), the particle mass ($m$), the speed 1 ($v_1$), the speed 2 ($v_2$), the electric potential 1 ($\varphi_1$), and the electric potential 2 ($\varphi_2$), the following relationship must be satisfied:
| $ \displaystyle\frac{1}{2} m v_1 ^2 + q \varphi_1 = \displaystyle\frac{1}{2} m v_2 ^2 + q \varphi_2 $ |
ID:(11596, 0)