Ferromagnet or solenoid
Image 
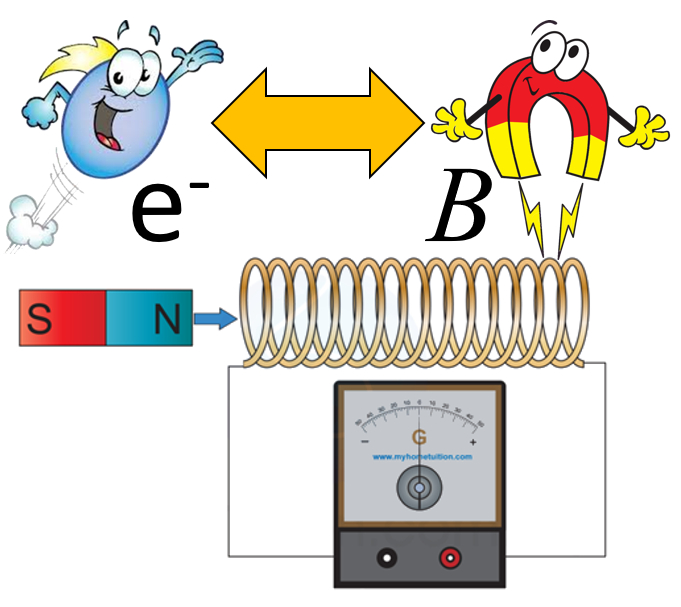
When current is allowed to flow through a solenoid, we observe that it creates a magnetic field similar to that of a magnetized bar. This indicates that the flow of electrons is capable of generating magnetic fields, and these fields are equivalent to permanent magnetic fields.

ID:(12116, 0)
Magnetic component of the Lorentz force
Equation 
The magnetic component of the Lorentz force is described by the following equation:
| $ \vec{F} = q ( \vec{E} + \vec{v} \times \vec{B} )$ |
This can be expressed as:
kyon
ID:(10056, 0)
Relationship between field and magnetic flux density
Equation 
El campo y flujo magnético son proporcionales, por lo que se tiene con que
| $ \vec{B} = \mu_0 \mu_r \vec{H}$ |
ID:(12171, 0)
Magnetic flux
Equation 
Similar to the electric field flux, we can introduce magnetic flux $\Phi$. If we introduce a magnetic flux density $B$, we can define the flux as:
ID:(12168, 0)
Faraday's law
Equation 
Faraday observo que la variación temporal del flujo magnético inducia una diferencia de potencial que, de conectarse a un alambre, puede generar una corriente. Esta relación se puede escribir con como
| $ \Delta\varphi = -\displaystyle\frac{d \Phi }{d t }$ |
El potencial creado se denomina también 'fuerza electromotriz' (fem) pues es el mecanismo con el que se genera la fuerza que mueve un motor.
ID:(12175, 0)
Faraday's law, average values
Equation 
La ley de Faraday que establece con magnetic flux $kg/C s$, potential difference $V$ and time $s$ que
| $ \Delta\varphi = -\displaystyle\frac{d \Phi }{d t }$ |
Para el caso que se trabaja con valores medios se puede estimar que la variación del flujo magnético es con magnetic flux $kg/C s$, potential difference $V$ and time $s$
| $ \Delta\varphi = -\displaystyle\frac{ \Delta\Phi }{ \Delta t }$ |
ID:(12256, 0)
Lenz's law
Image 
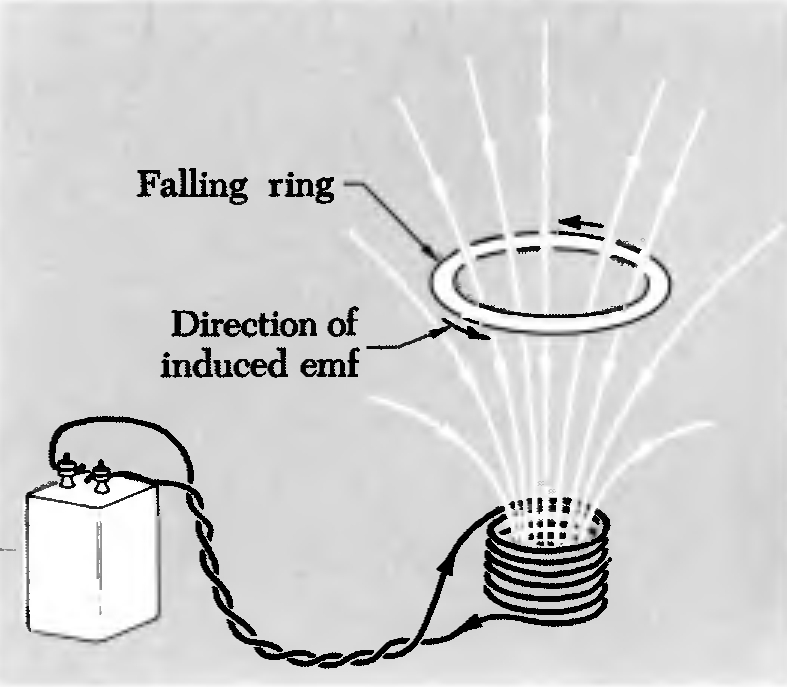
Al igual que en la mecánica en que la inercia trata de resistirse al cambio de estado en la electrodinámica existe un fenómeno análogo que fue descrito inicialmente por Lenz. Este se observa en particular en la inducción magnética en que las corrientes generadas son siempre de modo de oponerse al imán que las esta generando. En otras palabras las corrientes generadas crean un campo magnético que esta en oposición al que las origina inicialmente. Esto es lo que se denomina la ley de Lenz.
Ejemplo de la aplicación de la ley de Lenz: si se deja caer un anillo sobre un campo magnético se genera en el una corriente tal que genera un campo de polaridad opuesta que se resiste a caer sobre el campo existente.
ID:(12174, 0)
Potential difference due to flow change
Equation 
A simple example of flux variation can be estimated using the formula:
| $ \Delta\varphi = -\displaystyle\frac{ \Delta\Phi }{ \Delta t }$ |
Assuming that the magnetic flux density is constant across the section, represented by:
| $ \Phi =\displaystyle\int_S\vec{B}\cdot d\vec{S} $ |
In such a case, when a conductor is withdrawn from a magnetic field, the section of the conductor reduces as it moves. If we simplify the calculations by considering a rectangular shape, we can express it as:
$\displaystyle\frac{\Delta\Phi}{\Delta t} = B L \displaystyle\frac{\Delta s}{\Delta t}=B L v$
Here, the change in path over time is defined as velocity. Therefore, we can estimate that:
ID:(12177, 0)
Calculation of a magnetic field
Image 
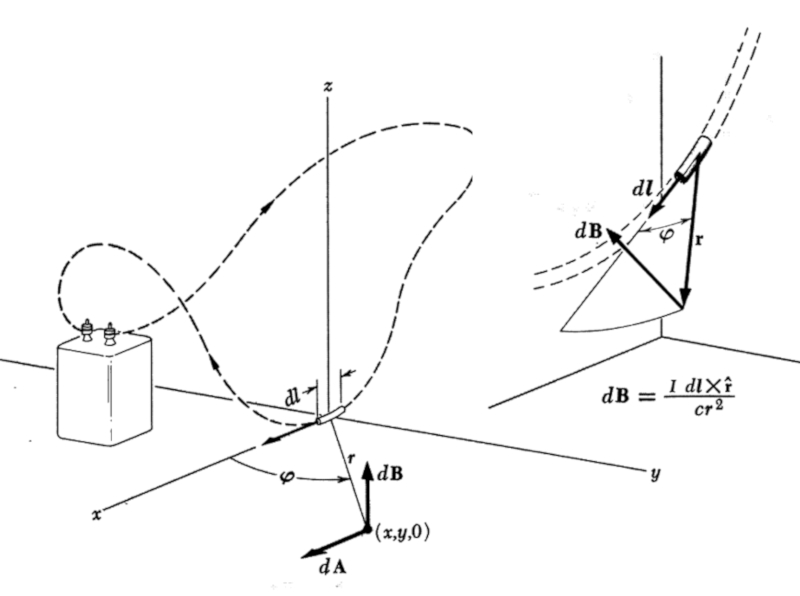
A magnetic field is constructed based on the collective contributions of all elements conducting electricity. When we consider one of these particular elements, it can be observed how it participates in the magnetic field, as depicted in the image:
ID:(12179, 0)
Biot-Savart law
Equation 
En general para el calculo de campos magnéticos se puede usar la llamada ley de Biot Savat que para una corriente dada en un segmento de alambre calcula su contribución a un campo magnético en un punto del espacio. Con esta es
| $ d\vec{H}= \displaystyle\frac{ I }{4\pi}\displaystyle\frac{ d\vec{l}\times \hat{r}}{r^2}$ |
ID:(12178, 0)