Storyboard

If the object (airplane / bird) maintains a slightly negative angle of attack, it can make part of the lifting force contribute to boost by countering the resistance. On the average that the remaining lift is not much less than the force of gravity, the object is long maintained in the air. You can talk about an extremely slow controlled descent or glide.

>Model

ID:(466, 0)

Iframe

>Top

ID:(15177, 0)

Description

>Top

One method of flying is known as gliding. In this technique, the wings are used both for propulsion and to remain airborne. To achieve this, it is necessary to adjust the wing's angle of attack in a way that the lift force counteracts gravity. As a result, gliding becomes a controlled descent where the descent is used to generate lift and thereby reduce speed in a controlled manner.

ID:(1171, 0)

Description

>Top

The key to gliding is to tilt the aircraft or bird forward, in other words, to have a negative angle represented by angle of attack of a wing ($\alpha$). With this negative angle, the the lift force ($F_L$) vector points upward and forward instead of backward. This results in a pulling force rather than the resistance force ($F_W$), propelling the aircraft or bird and generating speed, which, in turn, creates the necessary lift.

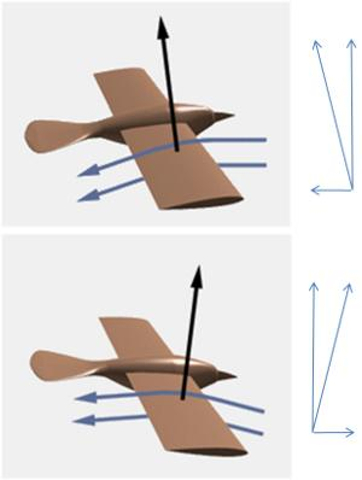
This mechanism allows for flight, but it's essential to understand that it's essentially a slow and controlled descent since it doesn't achieve a completely vertical a lift force ($F_L$) to counteract its own weight. Therefore, it's necessary to take the glider to high altitudes or allow the bird to gain initial height through its own propulsion. Afterward, both seek ascending air currents that enable them to glide within an updraft stronger than the glider's descent rate. This way, they can remain in flight for extended periods without the need to land.

ID:(7044, 0)

Description

>Top

Similarly to how the angle of attack of a wing ($\alpha$) is defined as the angle between the wing's centerline and the horizon, its negative counterpart can be defined as the glide angle ($\phi$).

Jonker JS3 Rapture sailplane glider (Air Cargo Week)

ID:(7047, 0)

Description

>Top

When it comes to forces, we have the following actions:

• the lift force ($F_L$) acts perpendicularly to the aircraft or bird's axis.
• the resistance force ($F_W$) acts along the aircraft or bird's axis.
• the gravitational force ($F_g$) ($mg$) acts vertically.

These three forces are depicted at the center of the diagram:

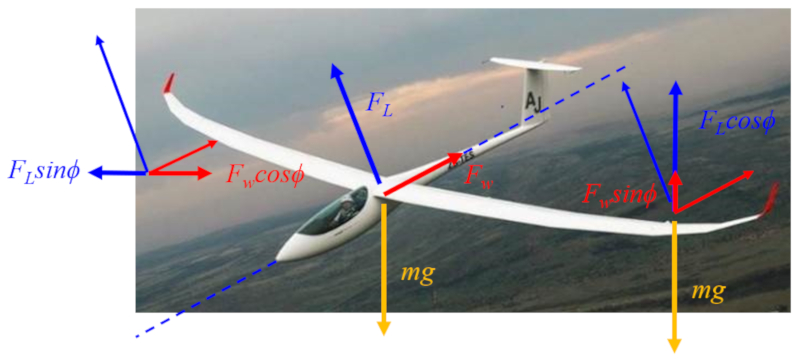
Jonker JS3 Rapture sailplane glider (Air Cargo Week)

On the left side, you can see the horizontal component, where lift counters the drag, acting as thrust.

On the right side, the vertical components are visible, where both aerodynamic forces (lift and drag) oppose the weight acting on the center of mass.

Although the forces balance each other out, the glider descends because its flight direction is determined by the glide angle.

ID:(7046, 0)

Description

>Top

The angle of planning is that angle of inclination in which the horizontal component of the traction force counteracts the horizontal friction while the sum of the support and friction in the vertical direction counteracts the force of gravity. This situation allows a descent with an angle equal to the gliding angle that can be small allowing a very slow descent.

ID:(1586, 0)

Concept

>Top

If we consider the lift force ($F_L$), the resistance force ($F_W$), the body mass ($m$), the gravitational Acceleration ($g$), and the glide angle ($\phi$), the force during gliding in the vertical direction is:

and in the horizontal direction is:

This allows us to eliminate the lift force ($F_L$), resulting in:

$F_L=F_W\displaystyle\frac{\cos\phi}{\sin\phi} \rightarrow F_W(\sin^2\phi+\cos^2\phi)=mg\sin\phi$

the resistance force ($F_W$) should be:

ID:(15769, 0)

Concept

>Top

If we consider the lift force ($F_L$), the resistance force ($F_W$), the body mass ($m$), the gravitational Acceleration ($g$), and the glide angle ($\phi$), the force during gliding in the vertical direction is:

and in the horizontal direction:

This allows us to eliminate the resistance force ($F_W$), resulting in:

$F_L=F_W\displaystyle\frac{\cos\phi}{\sin\phi} \rightarrow F_W(\sin^2\phi+\cos^2\phi)=mg\sin\phi$

Therefore, the lift force ($F_L$) is:

ID:(15770, 0)

Concept

>Top

Let's consider the lift force ($F_L$), the resistance force ($F_W$), the body mass ($m$), the gravitational Acceleration ($g$), and the glide angle ($\phi$). With these forces, the lift force is calculated as:

and the drag force as:

We can determine the glide angle ($\phi$) by dividing the lift force ($F_L$) by the resistance force ($F_W$), resulting in:

$\tan\phi=\displaystyle\frac{F_W}{F_L}$

Where the resistance force ($F_W$) is calculated using the equation:

with the total object profile ($S_p$) and the coefficient of resistance ($C_W$). Similarly, the lift force ($F_L$) is calculated as:

with the surface that generates lift ($S_w$) and the coefficient of lift ($C_L$).

With both forces, we can determine the necessary angle of attack for gliding as:

ID:(15771, 0)

Top

>Top

Parameters

$m$

m

Body mass

kg

$C_W$

C_W

Coefficient of resistance

-

$\rho$

rho

Density

kg/m^3

$\phi$

phi

Glide angle

rad

$g$

g

Gravitational Acceleration

m/s^2

$S_w$

S_w

Surface that generates lift

m^2

$S_p$

S_p

Total object profile

m^2

Variables

$F_L$

F_L

Lift force

N

$F_W$

F_W

Resistance force

N

$C_L$

C_L

Simple Model for Sustainability Coefficient

-

$v$

v

Speed with respect to the medium

m/s

Calculations

• Alternative method
• Traditional method
• Strategy
• 2D
• 3D

Equation

Number

First, select the equation: to , then, select the variable: to

---

Calculations

Symbol

Equation

Solved

Translated

Calculations

Symbol

Equation

Solved

Translated

Variable Given Calculate Target : Equation To be used

Equations

ID:(15190, 0)

Equation

>Top, >Model

In the case of gliding, the goal is to maintain a constant velocity, so the lift force ($F_L$) must generate sufficient propulsion to counteract the resistance force ($F_W$).

To achieve this lift force ($F_L$), the bird or aircraft generates a negative a angle of attack of a wing ($\alpha$), which means that part of the lift force ($F_L$) is converted into thrust. This component of force is equal to the sine of the angle.

The inclination also leads to a reduction in the resistance force ($F_W$), as a portion of it contributes to lift. In this case, the component that still contributes to resistance is this force multiplied by the cosine of the angle.

Therefore, the equation of force in the horizontal plane can be expressed as:

$ F_L \sin \phi = F_w \cos \phi $

Conditions

Variables

$\phi$

Glide angle

$rad$

6128

$F_L$

Lift force

$N$

6120

$F_W$

Resistance force

$N$

6124

Relation

Instead of using the angle of attack of a wing ($\alpha$), we will work with the glide angle ($\phi$).

ID:(4420, 0)

Equation

>Top, >Model

In the vertical plane, the glide angle ($\phi$) results in a reduction of the lift force ($F_L$) by a factor equal to the cosine of the angle. On the other hand, it causes the resistance force ($F_W$) to contribute to lift with a factor equal to the sine of the angle. Both forces must counteract the weight generated by the body mass ($m$) and the gravitational Acceleration ($g$), so we have:

$ F_L \cos \phi + F_w \sin \phi = m g $

Conditions

Variables

$m$

Body mass

$kg$

6150

$\phi$

Glide angle

$rad$

6128

$g$

Gravitational Acceleration

9.8

$m/s^2$

5310

$F_L$

Lift force

$N$

6120

$F_W$

Resistance force

$N$

6124

Relation

ID:(4419, 0)

Equation

>Top, >Model

The lift force ($F_L$) is with the body mass ($m$), the gravitational Acceleration ($g$) and the glide angle ($\phi$) equal to:

$ F_L = m g \cos\phi $

Conditions

Variables

$m$

Body mass

$kg$

6150

$\phi$

Glide angle

$rad$

6128

$g$

Gravitational Acceleration

9.8

$m/s^2$

5310

$F_L$

Lift force

$N$

6120

Relation

Development

If we consider the lift force ($F_L$), the resistance force ($F_W$), the body mass ($m$), the gravitational Acceleration ($g$), and the glide angle ($\phi$), the force during gliding in the vertical direction is:

$ F_L \cos \phi + F_w \sin \phi = m g $

and in the horizontal direction:

$ F_L \sin \phi = F_w \cos \phi $

This allows us to eliminate the resistance force ($F_W$), resulting in:

$F_L=F_W\displaystyle\frac{\cos\phi}{\sin\phi} \rightarrow F_W(\sin^2\phi+\cos^2\phi)=mg\sin\phi$

Therefore, the lift force ($F_L$) is:

$ F_L = m g \cos\phi $

ID:(4421, 0)

Equation

>Top, >Model

The resistance force ($F_W$) is with the body mass ($m$), the gravitational Acceleration ($g$) and the glide angle ($\phi$) equal to:

$ F_W = m g \sin \phi $

Conditions

Variables

$m$

Body mass

$kg$

6150

$\phi$

Glide angle

$rad$

6128

$g$

Gravitational Acceleration

9.8

$m/s^2$

5310

$F_W$

Resistance force

$N$

6124

Relation

Development

If we consider the lift force ($F_L$), the resistance force ($F_W$), the body mass ($m$), the gravitational Acceleration ($g$), and the glide angle ($\phi$), the force during gliding in the vertical direction is:

$ F_L \cos \phi + F_w \sin \phi = m g $

and in the horizontal direction is:

$ F_L \sin \phi = F_w \cos \phi $

This allows us to eliminate the lift force ($F_L$), resulting in:

$F_L=F_W\displaystyle\frac{\cos\phi}{\sin\phi} \rightarrow F_W(\sin^2\phi+\cos^2\phi)=mg\sin\phi$

the resistance force ($F_W$) should be:

$ F_W = m g \sin \phi $

ID:(4422, 0)

Equation

>Top, >Model

The lift force ($F_L$) and the resistance force ($F_W$) depend on the body mass ($m$), the gravitational Acceleration ($g$), and the glide angle ($\phi$). Both equations allow us to calculate the glide angle ($\phi$) in terms of the lift force ($F_L$) and the resistance force ($F_W$).

Since the lift force ($F_L$) and the resistance force ($F_W$) are functions of the body mass ($m$), the speed with respect to the medium ($v$), the surface that generates lift ($S_w$), the total object profile ($S_p$), the coefficient of lift ($C_L$), and the coefficient of resistance ($C_W$), we can demonstrate that the glide angle ($\phi$) is:

$ \tan \phi =\displaystyle\frac{ S_p C_w }{ S_w C_L }$

Conditions

Variables

$C_W$

Coefficient of resistance

$-$

6122

$\phi$

Glide angle

$rad$

6128

$C_L$

Simple Model for Sustainability Coefficient

$-$

6164

$S_w$

Surface that generates lift

$m^2$

6117

$S_p$

Total object profile

$m^2$

6123

Relation

Development

Let's consider the lift force ($F_L$), the resistance force ($F_W$), the body mass ($m$), the gravitational Acceleration ($g$), and the glide angle ($\phi$). With these forces, the lift force is calculated as:

$ F_L = m g \cos\phi $

and the drag force as:

$ F_W = m g \sin \phi $

We can determine the glide angle ($\phi$) by dividing the lift force ($F_L$) by the resistance force ($F_W$), resulting in:

$\tan\phi=\displaystyle\frac{F_W}{F_L}$

Where the resistance force ($F_W$) is calculated using the equation:

$ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$

with the total object profile ($S_p$) and the coefficient of resistance ($C_W$). Similarly, the lift force ($F_L$) is calculated as:

$ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$

with the surface that generates lift ($S_w$) and the coefficient of lift ($C_L$).

With both forces, we can determine the necessary angle of attack for gliding as:

$ \tan \phi =\displaystyle\frac{ S_p C_w }{ S_w C_L }$

ID:(4423, 0)

Equation

>Top, >Model

To generate higher pressure below than above the wing and generate lift, Bernoulli's principle is employed, correcting for the lack of energy density conservation using ($$). The pressure over the wing, the lift force ($F_L$), can be estimated using the density ($\rho$), the surface that generates lift ($S_w$), the coefficient of lift ($C_L$), and the speed with respect to the medium ($v$) through the following formula:

$ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$

Conditions

Variables

$\rho$

Density

$kg/m^3$

5342

$F_L$

Lift force

$N$

6120

$C_L$

Simple Model for Sustainability Coefficient

$-$

6164

$v$

Speed with respect to the medium

$m/s$

6110

$S_w$

Surface that generates lift

$m^2$

6117

Relation

Development

The lift force ($F_L$), along with the wing span ($L$), the density ($\rho$), the wing top speed factor ($c_t$), the wing bottom speed factor ($c_b$), the upper wing length ($l_t$), the bottom wing length ($l_b$), and the speed with respect to the medium ($v$), is found in

$ F_L = \rho L ( c_b l_b - c_t l_t ) v ^2$

If we consider the surface that generates lift ($S_w$), given by the wing span ($L$), the upper wing length ($l_t$), and the bottom wing length ($l_b$),

$ S_w = \displaystyle\frac{1}{2} L ( l_t + l_b )$

and for the coefficient of lift ($C_L$), defined as

$ C_L = 4\displaystyle\frac{ c_t l_t - c_b l_b }{ l_t + l_b }$

we obtain

$ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$

ID:(4417, 0)

Equation

>Top, >Model

The resistance force ($F_W$) kann mit the density ($\rho$), the coefficient of resistance ($C_W$), the total object profile ($S_p$) und the speed with respect to the medium ($v$) entsprechend berechnet werden folgende Formel:

$ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$

Conditions

Variables

$C_W$

Coefficient of resistance

$-$

6122

$\rho$

Density

$kg/m^3$

5342

$F_W$

Resistance force

$N$

6124

$v$

Speed with respect to the medium

$m/s$

6110

$S_p$

Total object profile

$m^2$

6123

Relation

Development

Similarly to how the equation for the lift force ($F_L$) was derived using the density ($\rho$), the coefficient of lift ($C_L$), the surface that generates lift ($S_w$), and the speed with respect to the medium ($v$)

$ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$

in this analogy, what corresponds to the surface that generates lift ($S_w$) will be equivalent to the total object profile ($S_p$) and the coefficient of lift ($C_L$) to the coefficient of resistance ($C_W$), thus the resistance force ($F_W$) is calculated:

$ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$

The drag coefficient is measured and, in turbulent flows over aerodynamic bodies, values are generally found around 0.4.

ID:(4418, 0)