User:
Mechanisms
Iframe 
Mechanisms
ID:(15169, 0)
Wing in the flow
Concept
If we assume that the flow around a wing is laminar, we can observe multiple layers surrounding the wing. Those on the upper side tend to be slightly longer due to the upward curvature, while the lower layers tend to be shorter and, therefore, closer to the wing.
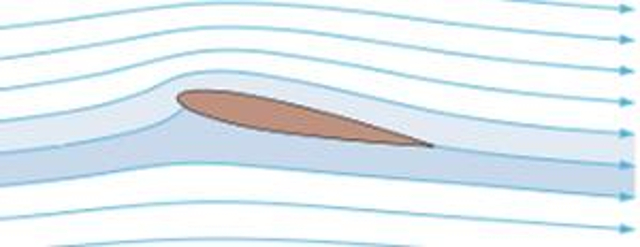
Supposing that the flow is such that these layers converge in a way that points close together on either side of the wing return to the same relative position once the flow separates, the speed of the upper layers will necessarily be higher than that of the lower layers. It's important to keep in mind that this is just an assumption, and there is no real necessity for them to converge; in fact, they could end up being out of phase without any issues.
ID:(7016, 0)
Force on the wing
Concept
Since the velocity in the upper layers of the wing is greater than in the lower layers, this implies that the pressure on the upper surface of the wing is lower than on the lower surface.
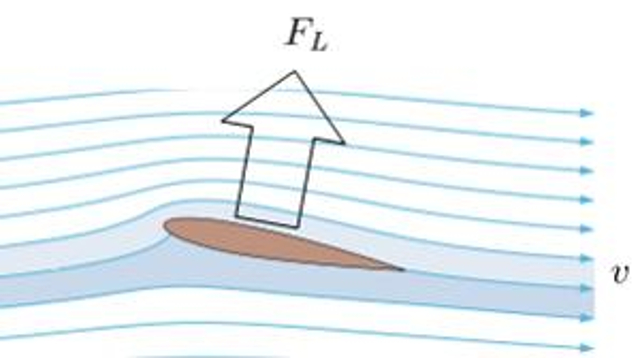
This effectively means that there is a greater force from below the wing compared to above the wing, which leads to the generation of lift force.
ID:(7018, 0)
Flight, balance of forces
Concept
The forces that influence an aircraft or bird can be categorized into two fundamental groups:
Forces that impact the control of the center of mass's movement:
• the lift force ($F_L$), which counteracts the gravitational force ($F_g$).
• the propulsion force ($F_p$), which opposes the resistance force ($F_W$).
Forces aimed at achieving the rotation of the aircraft or bird around the center of mass, achieved through the ailerons on the wings and the rudder:
• Ailerons allow the generation of a turning moment by asymmetrically altering lift on each wing.
• The rudder controls the direction of the aircraft or bird by redirecting the airflow.
Boeing Images - 777-300ER Illustration in Boeing Livery
Key parameters for controlling the center of mass's movement are:
• the surface that generates lift ($S_w$) and the total object profile ($S_p$).
• the coefficient of lift ($C_L$) and the coefficient of resistance ($C_W$), the latter being dependent on the angle of attack of a wing ($\alpha$).
ID:(11080, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ F_g = m g $
F_g = m_g * g
$ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$
F_L = rho * S_w * C_L * v ^2/2
$ F_R = F_W \cos \alpha + F_L \sin \alpha $
F_R = F_W *cos( alpha )+ F_L *sin( alpha )
$ F_R = \displaystyle\frac{1}{2} \rho S_p C_w v ^2 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v ^2}$
F_R = rho * S_p * C_w * v ^2/2+2* m ^2* g ^2/( c ^2* S_w * rho * v ^2)
$ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$
F_W = rho * S_p * C_W * v ^2/2
$ P = F_R v $
P = F_R * v
$ P =\displaystyle\frac{1}{2} \rho S_p C_W v ^3 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v }$
P = rho * S_p * C_W * v ^3/2+2* m ^2* g ^2/( c ^2* S_w * rho * v )
ID:(15170, 0)
Gravitational Force
Equation
The gravitational Force ($F_g$) is based on the gravitational mass ($m_g$) of the object and on a constant reflecting the intensity of gravity at the planet's surface. The latter is identified by the gravitational Acceleration ($g$), which is equal to $9.8 m/s^2$.
Consequently, it is concluded that:
| $ F_g = m g $ |
| $ F_g = m_g g $ |
ID:(3241, 0)
Lift force
Equation
To generate higher pressure below than above the wing and generate lift, Bernoulli's principle is employed, correcting for the lack of energy density conservation using ($$). The pressure over the wing, the lift force ($F_L$), can be estimated using the density ($\rho$), the surface that generates lift ($S_w$), the coefficient of lift ($C_L$), and the speed with respect to the medium ($v$) through the following formula:
| $ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$ |
The lift force ($F_L$), along with the wing span ($L$), the density ($\rho$), the wing top speed factor ($c_t$), the wing bottom speed factor ($c_b$), the upper wing length ($l_t$), the bottom wing length ($l_b$), and the speed with respect to the medium ($v$), is found in
| $ F_L = \rho L ( c_b l_b - c_t l_t ) v ^2$ |
If we consider the surface that generates lift ($S_w$), given by the wing span ($L$), the upper wing length ($l_t$), and the bottom wing length ($l_b$),
| $ S_w = \displaystyle\frac{1}{2} L ( l_t + l_b )$ |
and for the coefficient of lift ($C_L$), defined as
| $ C_L = 4\displaystyle\frac{ c_t l_t - c_b l_b }{ l_t + l_b }$ |
we obtain
| $ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$ |
ID:(4417, 0)
Total force of resistance
Equation
To calculate the total resistance force ($F_R$), we assume small angles and consider a situation where the angle is such that it maintains the body mass ($m$). Using this approximation and the variables the coefficient of lift ($C_L$), the coefficient of resistance ($C_W$), the surface that generates lift ($S_w$), the total object profile ($S_p$), the gravitational Acceleration ($g$), the proportionality constant coefficient sustainability ($c$), the density ($\rho$), and the speed with respect to the medium ($v$), we obtain the following expression:
| $ F_R = \displaystyle\frac{1}{2} \rho S_p C_w v ^2 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v ^2}$ |
Using the relationships of the total resistance force ($F_R$) with the lift force ($F_L$), the resistance force ($F_W$), and the angle of attack of a wing ($\alpha$):
| $ F_R = F_W \cos \alpha + F_L \sin \alpha $ |
we can calculate using the resistance force with the density ($\rho$), the coefficient of resistance ($C_W$), the total object profile ($S_p$), and the speed with respect to the medium ($v$):
| $ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
and the lift force with the surface that generates lift ($S_w$) and the coefficient of lift ($C_L$):
| $ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$ |
using the relationship for the coefficient of lift ($C_L$) with the proportionality constant coefficient sustainability ($c$):
| $ C_L = c \alpha $ |
using the relationship for the sine of the small angle of attack $\alpha$:
| $\sin\alpha\sim\alpha$ |
and the cosine:
| $\cos\alpha\sim 1$ |
with the condition to balance the weight of the bird or aircraft for the body mass ($m$) and the gravitational Acceleration ($g$):
| $ \alpha =\displaystyle\frac{2 m g }{ c \rho S_w }\displaystyle\frac{1}{ v ^2}$ |
we obtain:
| $ F_R = \displaystyle\frac{1}{2} \rho S_p C_w v ^2 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v ^2}$ |
ID:(4546, 0)
Total resistance force calculation
Equation
The total force of resistance is composed of the horizontal components of the wing profile's resistance force $F_W$ and the lift force $F_L$, which can be calculated from the angle of attack $\alpha$:
| $ F_R = F_W \cos \alpha + F_L \sin \alpha $ |
The horizontal component of the lift force corresponds to the force $F_L$ multiplied by the sine of the angle of attack $\alpha$:
$F_L \sin\alpha $
And the horizontal component of the drag force corresponds to the force $F_W$ multiplied by the cosine of the angle of attack $\alpha$:
$F_W \cos\alpha $
Therefore, the total resistance force can be calculated as:
| $ F_R = F_W \cos \alpha + F_L \sin \alpha $ |
ID:(9579, 0)
Resistance force
Equation
The resistance force ($F_W$) kann mit the density ($\rho$), the coefficient of resistance ($C_W$), the total object profile ($S_p$) und the speed with respect to the medium ($v$) entsprechend berechnet werden folgende Formel:
| $ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
Similarly to how the equation for the lift force ($F_L$) was derived using the density ($\rho$), the coefficient of lift ($C_L$), the surface that generates lift ($S_w$), and the speed with respect to the medium ($v$)
| $ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$ |
in this analogy, what corresponds to the surface that generates lift ($S_w$) will be equivalent to the total object profile ($S_p$) and the coefficient of lift ($C_L$) to the coefficient of resistance ($C_W$), thus the resistance force ($F_W$) is calculated:
| $ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
The drag coefficient is measured and, in turbulent flows over aerodynamic bodies, values are generally found around 0.4.
ID:(4418, 0)
Flight power
Equation
Power $P$ is the energy per unit of time that needs to be supplied to sustain a given force $F_R$. Therefore, it can be calculated based on the force by multiplying it by the velocity $v$:
| $ P = F_R v $ |
The power is defined as the energy $\Delta W$ per time $\Delta t$ according to the equation:
| $ P =\displaystyle\frac{ \Delta W }{ \Delta t }$ |
Since energy is equal to force $F$ multiplied by the distance traveled $\Delta s$, we have:
| $ \Delta W = F \Delta s $ |
Thus, we obtain:
$P=\displaystyle\frac{\Delta W}{\Delta t}= F_R \displaystyle\frac{\Delta s}{\Delta t}$
However, since the distance traveled in a time interval is the velocity $v$:
| $ \bar{v} \equiv\displaystyle\frac{ \Delta s }{ \Delta t }$ |
Finally, we can write the expression for power as:
| $ P = F_R v $ |
ID:(4547, 0)
General flight power
Equation
To obtain the power of flight ($P$), you need to multiply the total resistance force ($F_R$) by the speed with respect to the medium ($v$). Since the total resistance force ($F_R$) is a function of the density ($\rho$), the surface that generates lift ($S_w$), the total object profile ($S_p$), the coefficient of resistance ($C_W$), the proportionality constant coefficient sustainability ($c$), the body mass ($m$), and the gravitational Acceleration ($g$), which is equal to
| $ F_R = \displaystyle\frac{1}{2} \rho S_p C_w v ^2 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v ^2}$ |
,
the potential is
| $ P =\displaystyle\frac{1}{2} \rho S_p C_W v ^3 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v }$ |
The total resistance force ($F_R$) is a function of the density ($\rho$), the surface that generates lift ($S_w$), the total object profile ($S_p$), the coefficient of resistance ($C_W$), the proportionality constant coefficient sustainability ($c$), the body mass ($m$), and the gravitational Acceleration ($g$), which is equal to
| $ F_R = \displaystyle\frac{1}{2} \rho S_p C_w v ^2 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v ^2}$ |
,
therefore, using the equation for the power of flight ($P$)
| $ P = F_R v $ |
,
we obtain:
| $ P =\displaystyle\frac{1}{2} \rho S_p C_W v ^3 + \displaystyle\frac{2 m ^2 g ^2}{ c ^2 S_w \rho }\displaystyle\frac{1}{ v }$ |
.
ID:(4548, 0)