User:
Interior flow and erosion
Storyboard 
Internal flow occurs through the capillaries formed between the soil particles. Whenever these capillaries have dimensions greater than those of the small clay plates, there is a risk that these clay particles may be carried away by this flow. If this happens, the soil could lose some of its clay content, which would impact its mechanical properties, stability, and support for organic life.
ID:(379, 0)
Energy density
Equation
Since a fluid or gas is a continuum, the concept of energy can no longer be associated with a specific mass. However, it is possible to consider the energy contained in a volume of the continuum, and by dividing it by the volume itself, we obtain the energy density ($e$). Therefore, with the density ($\rho$), the speed on a cylinder radio ($v$), the column height ($h$), the gravitational Acceleration ($g$), and the water column pressure ($p$), we have:
 $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
Another useful equation is the one corresponding to the conservation of energy, which is applicable in cases where viscosity, a process that leads to energy loss, can be neglected. If we consider the classic energy equation $E$, which takes into account kinetic energy, gravitational potential energy, and an external force displacing the liquid over a distance $\Delta z$, it can be expressed as:
$E=\displaystyle\frac{m}{2}v^2+mgh+F\Delta x$
If we consider the energy within a volume $\Delta x\Delta y\Delta z$, we can replace the mass with:
$m=\rho \Delta x\Delta y\Delta z$
And since pressure is given by:
$F=p \Delta S =p \Delta y\Delta z$
We obtain the equation for energy density:
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
which corresponds to the Bernoulli equation.
ID:(3159, 0)
General Bernoulli equation
Equation
With the mean Speed of Fluid in Point 1 ($v_1$), the height or depth 1 ($h_1$), and the pressure in column 1 ($p_1$) representing the velocity, height, and pressure at point 1, respectively, and the mean Speed of Fluid in Point 2 ($v_2$), the height or depth 2 ($h_2$), and the pressure in column 2 ($p_2$) representing the velocity, height, and pressure at point 2, respectively, we have:
| $\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $ |
If we assume that the energy density ($e$) is conserved, we can state that for a cell where the average velocity is the speed on a cylinder radio ($v$), the density is the density ($\rho$), the pressure is the water column pressure ($p$), the height is the column height ($h$), and the gravitational acceleration is the gravitational Acceleration ($g$), the following holds:
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
At point 1, this equation will be equal to the same equation at point 2:
$e(v_1,p_1,h_1)=e(v_2,p_2,h_2)$
where the mean Speed of Fluid in Point 1 ($v_1$), the height or depth 1 ($h_1$), and the pressure in column 1 ($p_1$) represent the velocity, height, and pressure at point 1, respectively, and the mean Speed of Fluid in Point 2 ($v_2$), the height or depth 2 ($h_2$), and the pressure in column 2 ($p_2$) represent the velocity, height, and pressure at point 2, respectively. Therefore, we have:
| $\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $ |
ID:(4504, 0)
Bernoulli equation, variations
Equation
The variación de la Presión ($\Delta p$) can be calculated from the average speed ($\bar{v}$) and the speed difference between surfaces ($\Delta v$) with the density ($\rho$) using
| $ \Delta p = - \rho \bar{v} \Delta v $ |
In the case where there is no hystrostatic pressure, Bernoulli's law for the density ($\rho$), the pressure in column 1 ($p_1$), the pressure in column 2 ($p_2$), the mean Speed of Fluid in Point 1 ($v_1$) and the mean Speed of Fluid in Point 2 ($v_2$)
| $\displaystyle\frac{1}{2} \rho v_1 ^2 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2 + p_2 $ |
can be rewritten with the variación de la Presión ($\Delta p$)
| $ \Delta p = p_2 - p_1 $ |
and keeping in mind that
$v_2^2 - v_1^2 = \displaystyle\frac{1}{2}(v_2-v_1)(v_1+v_2)$
with
| $ \bar{v} = \displaystyle\frac{ v_1 + v_2 }{2}$ |
and
| $ \Delta v = v_2 - v_1 $ |
you have to
| $ \Delta p = - \rho \bar{v} \Delta v $ |
which allows us to see the effect of the average speed of a body and the difference between its surfaces, as observed in an airplane or bird wing.
ID:(4835, 0)
Flow after Hagen-Poiseuille equation
Concept
The profile of the speed on a cylinder radio ($v$) in the radius of position in a tube ($r$) allows us to calculate the volume flow ($J_V$) in a tube by integrating over the entire surface, which leads us to the well-known Hagen-Poiseuille law.
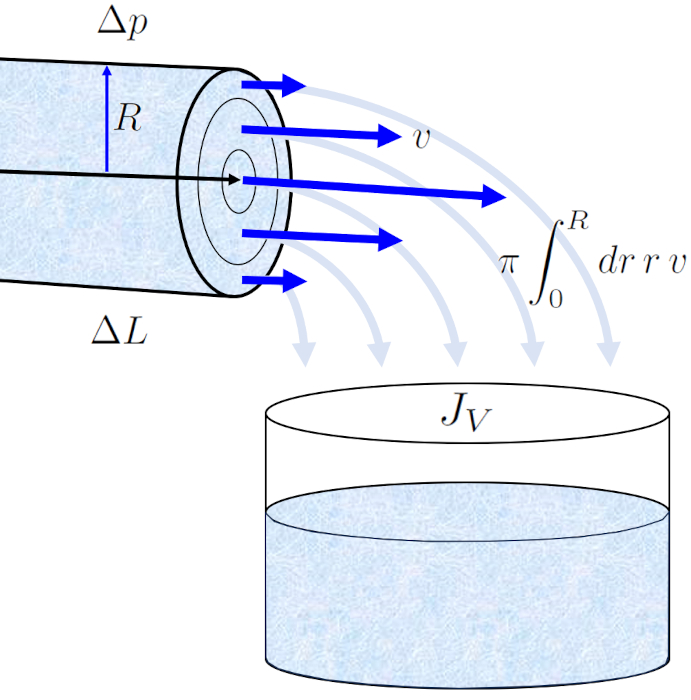
The result is an equation that depends on tube radius ($R$) raised to the fourth power. However, it is crucial to note that this flow profile only holds true in the case of laminar flow.
Thus, from the viscosity ($\eta$), it follows that the volume flow ($J_V$) before ($$) and ($$), the expression:
| $ J_V =-\displaystyle\frac{ \pi R ^4}{8 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
The original papers that gave rise to this law with a combined name were:
"Ueber die Gesetze, welche des der Strom des Wassers in röhrenförmigen Gefässen bestimmen" (On the laws governing the flow of water in cylindrical vessels), Gotthilf Hagen, Annalen der Physik und Chemie 46:423442 (1839).
"Recherches expérimentales sur le mouvement des liquides dans les tubes de très-petits diamètres" (Experimental research on the movement of liquids in tubes of very small diameters), Jean-Louis-Marie Poiseuille, Comptes Rendus de l'Académie des Sciences 9:433544 (1840).
ID:(2216, 0)
Speed profile of flow in a cylinder
Equation
When solving the flow equation with the boundary condition, we obtain the speed on a cylinder radio ($v$) as a function of the curvature radio ($r$), represented by a parabola centered at the maximum flow rate ($v_{max}$) and equal to zero at the tube radius ($R$):
| $ v = v_{max} \left(1-\displaystyle\frac{ r ^2}{ R ^2}\right)$ |
When a the pressure difference ($\Delta p_s$) acts on a section with an area of $\pi R^2$, with the tube radius ($R$) as the curvature radio ($r$), it generates a force represented by:
$\pi r^2 \Delta p$
This force drives the liquid against viscous resistance, given by:
| $ F_v =-2 \pi r \Delta L \eta \displaystyle\frac{ dv }{ dr }$ |
By equating these two forces, we obtain:
$\pi r^2 \Delta p = \eta 2\pi r \Delta L \displaystyle\frac{dv}{dr}$
Which leads to the equation:
$\displaystyle\frac{dv}{dr} = \displaystyle\frac{1}{2\eta}\displaystyle\frac{\Delta p}{\Delta L} r$
If we integrate this equation from a position defined by the curvature radio ($r$) to the edge where the tube radius ($R$) (taking into account that the velocity at the edge is zero), we can obtain the speed on a cylinder radio ($v$) as a function of the curvature radio ($r$):
| $ v = v_{max} \left(1-\displaystyle\frac{ r ^2}{ R ^2}\right)$ |
Where:
| $ v_{max} =-\displaystyle\frac{ R ^2}{4 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
is the maximum flow rate ($v_{max}$) at the center of the flow.
.
ID:(3627, 0)
Maximal speed of flow in a cylinder
Equation
The value of the maximum flow rate ($v_{max}$) at the center of a cylinder depends on the viscosity ($\eta$), the tube radius ($R$), and the gradient created by the pressure difference ($\Delta p_s$) and the tube length ($\Delta L$), as represented by:
| $ v_{max} =-\displaystyle\frac{ R ^2}{4 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
The negative sign indicates that the flow always occurs in the direction opposite to the gradient, meaning from the area of higher pressure to the area of lower pressure.
ID:(3628, 0)
Condición de erosión generalizada
Equation
La plaquita de arcilla sera arrastrada por la corriente en la medida que la fuerza hidrostática
Por ello la condición de ser arrastrada es:
| $ dp S > m g $ |
ID:(4506, 0)
Masa de Plaquita de Arcilla
Equation
La masa de la plaquita se puede calcular de la densidad solida del material y del volumen mediante\\n\\n
$m=\rho_sV$
\\n\\nEl volumen se calcula del cuadrado del lado
$V=w_cl_c^2$
Con ello la masa del la plaquita es:
| $ m = \rho_s w_c l_c ^2$ |
ID:(4508, 0)
Sección de Plaquita de Arcilla
Equation
La sección
| $ S = l_c ^ 2$ |
ID:(4507, 0)
Condición de erosión en función de geometría
Equation
La condición de estabilidad general
| $ dp S > m g $ |
se puede reescribir con la masa
| $ m = \rho_s w_c l_c ^2$ |
y la sección
| $ S = l_c ^ 2$ |
como
| $dp > \rho_s w_c g $ |
ID:(10630, 0)