User: 
Thermal conduction
Storyboard 
Heat transport consists of two alternating processes. On one hand, there is conduction through a medium, and on the other, the transfer of heat from one medium to another.
Transport through a medium involves the transfer of heat into the medium, followed by conduction through it, and finally, the transmission of heat out of the medium. In more complex media, multiple stages of conduction and transfer between different media must be considered. If heat is generated or absorbed in one of the media, conduction from or to that heat source or sink is accounted for.
ID:(313, 0)
Heat conduction mechanism
Concept
The heat conducted by a
and the conductivity
ID:(7718, 0)
Geometry and material dependence
Top
One of the key factors determining how much heat can be conducted through a solid or liquid is its cross-sectional area, which refers to the quantity of atom chains available. The more of these chains we have, the greater our heat transport capacity.
However, the length of these chains can be counterproductive. As the spring chain becomes longer, our ability to transmit heat decreases, as more atoms must adjust their oscillation amplitudes.
If we represent this with the section ($S$) and the conductor length ($L$), the diagram takes the following form:
Finally, the ability of the medium and material to transport heat, described by coefficients the internal transmission coefficient ($\alpha_i$) and the external transmission coefficient ($\alpha_e$), and the thermal conductivity ($\lambda$), explains how heat moves in response to the temperature difference ($\Delta T$) created by the difference between the indoor temperature ($T_i$) and the outdoor temperature ($T_e$):
This is calculated as:
| $ \Delta T = T_i - T_e $ |
ID:(15235, 0)
Heat conduction
Top
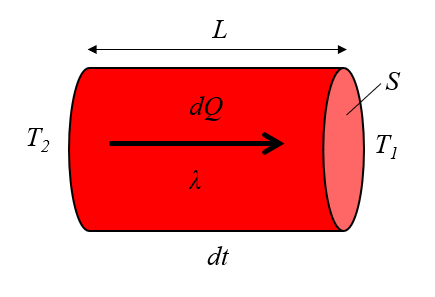
Heat conduction was first modeled by Jean Baptiste Joseph Fourier [1], who established that the heat flow rate ($q$), defined using the heat transported ($dQ$), the time variation ($dt$), and the section ($S$), is expressed by:
| $ q \equiv \displaystyle\frac{1}{ S }\displaystyle\frac{ dQ }{ dt }$ |
This theory is also related to the section ($S$), the conductor length ($L$), the temperature difference in the conductor ($\Delta T_0$), and the thermal conductivity ($\lambda$), as shown in:
| $ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $ |
and is illustrated with the following diagram:
[1] "Théorie Analytique de la Chaleur" (The Analytical Theory of Heat), Jean Baptiste Joseph Fourier, 1822.
ID:(15236, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ \Delta T_0 = T_{is} - T_{es} $
DT_0 = T_is - T_es
$ q \equiv \displaystyle\frac{1}{ S }\displaystyle\frac{ dQ }{ dt }$
q = dQ /( S * dt )
$ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $
q = lambda * DT_0 / L
$ q =\displaystyle\frac{ \lambda }{ r_e \ln\left(\displaystyle\frac{ r_e }{ r_i }\right)} \Delta T_0 $
q = lambda *ln( r_e / r_i )* DT_0 / r_e
$ S = 2 \pi r_e L $
S = 2* pi * r_e * L
$ T_z = T_{is} + ( T_{es} - T_{is} )\displaystyle\frac{ z }{ L }$
T_z = T_is + ( T_es - T_is )* z / L
$T_r=\displaystyle\frac{\ln\left(\displaystyle\frac{r}{r_i}\right)}{\ln\left(\displaystyle\frac{r_e}{r_i}\right)}(T_{es}-T_{is})+T_{is}$
T(r)=(ln(r/r_i)/ln(r_e/r_i))(T_es-T_is)+T_is
ID:(15334, 0)
Surface temperature difference
Equation
In the case of a solid, and similarly for a liquid, we can describe the system as a structure of atoms held together by something that behaves like a spring. When both ends have temperatures of ($$), with the inner surface temperature ($T_{is}$) and the outer surface temperature ($T_{es}$):
| $ \Delta T_0 = T_{is} - T_{es} $ |
ID:(15120, 0)
Heat flux
Equation
The the heat flow rate ($q$) is defined based on the heat transported ($dQ$) passing through the section ($S$) in the time variation ($dt$):
| $ q \equiv \displaystyle\frac{1}{ S }\displaystyle\frac{ dQ }{ dt }$ |
ID:(3482, 0)
Temperature distribution
Equation
The temperature in radius r ($T_r$) is a function of the radio ($r$) which with the inside radius ($r_i$), the inner surface temperature ($T_{is}$), the outdoor radio ($r_e$) and < var>5214 is:
| $ T_z = T_{is} + ( T_{es} - T_{is} )\displaystyle\frac{ z }{ L }$ |
ID:(15747, 0)
Calculation of heat conduction
Equation
The heat flux ($q$) is a function of the thermal conductivity ($\lambda$), the conductor length ($L$) and the temperature difference in the conductor ($\Delta T_0$):
| $ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $ |
ID:(7712, 0)
Radial temperature distribution in tube
Equation
The temperature in radius r ($T_r$) is a function of the radio ($r$) which with the inside radius ($r_i$), the inner surface temperature ($T_{is}$), the outdoor radio ($r_e$) and < var>5214 is:
| $T_r=\displaystyle\frac{\ln\left(\displaystyle\frac{r}{r_i}\right)}{\ln\left(\displaystyle\frac{r_e}{r_i}\right)}(T_{es}-T_{is})+T_{is}$ |
The temporal variation of the heat flux $\dot{Q}$ in polar coordinates, where $r$ represents the radius and the thermal conductivity ($\lambda$) is:
$\dot{Q}=-\lambda\displaystyle\frac{1}{r}\displaystyle\frac{\partial}{\partial r}\left(r\displaystyle\frac{\partial T}{\partial r}\right)$
When the flux is stationary, its time derivative is zero and the equation reduces to zero. The solution to this equation, given the boundary conditions where the temperatures at the inside radius ($r_i$) are the inner surface temperature ($T_{is}$) and at the outdoor radio ($r_e$) are the outer surface temperature ($T_{es}$), is described as:
| $T_r=\displaystyle\frac{\ln\left(\displaystyle\frac{r}{r_i}\right)}{\ln\left(\displaystyle\frac{r_e}{r_i}\right)}(T_{es}-T_{is})+T_{is}$ |
ID:(7741, 0)
Heat Flow in tube
Equation
The heat flow rate ($q$) is with the temperature difference in the conductor ($\Delta T_0$), the thermal conductivity ($\lambda$), the inside radius ($r_i$) and the outdoor radio ($r_e$) is:
| $ q =\displaystyle\frac{ \lambda }{ r_e \ln\left(\displaystyle\frac{ r_e }{ r_i }\right)} \Delta T_0 $ |
ID:(7742, 0)
Tube surface
Equation
The section ($S$) is a function of the conductor length ($L$) and the outdoor radio ($r_e$) is:
| $ S = 2 \pi r_e L $ |
ID:(15748, 0)