User:
Heat Transportation
Storyboard 
Heat transport through a system composed of multiple media can be estimated by analyzing how heat is conducted within each medium and transferred at each interface. The calculation is performed using the specific parameters of each medium and interface, as well as the temperatures at both ends of the system, thereby providing the temperatures at each interface.
ID:(1483, 0)
Heat transport
Concept
The basic system includes a transfer generated by the temperature difference ($\Delta T$), which consists of the temperature difference at internal interface ($\Delta T_i$), the temperature difference in the conductor ($\Delta T_0$), and the temperature difference at external interface ($\Delta T_e$). Therefore:
| $ \Delta T = \Delta T_i + \Delta T_0 + \Delta T_e $ |
With the heat flow rate ($q$) being responsible for the transfer between the interior and the conductor, using the internal transmission coefficient ($\alpha_i$):
| $ q = \alpha_i \Delta T_i $ |
Conduction involves the thermal conductivity ($\lambda$) and the conductor length ($L$):
| $ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $ |
And the transfer from the conductor to the exterior, with the external transmission coefficient ($\alpha_e$), is represented by:
| $ q = \alpha_e \Delta T_e $ |
All this is graphically represented by:
ID:(7723, 0)
Heat transport between two systems via a third medium
Concept
The heat flow rate ($q$) is calculated from the total transport coefficient (multiple medium, two interfaces) ($k$) and the temperature difference ($\Delta T$) using the following equation:
| $ q = k \Delta T $ |
where the total transport coefficient (multiple medium, two interfaces) ($k$) is derived from the external transmission coefficient ($\alpha_e$), the internal transmission coefficient ($\alpha_i$), the thermal conductivity ($\lambda$), and the conductor length ($L$) through this equation:
| $\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_i }+\displaystyle\frac{1}{ \alpha_e }+\displaystyle\frac{ L }{ \lambda }$ |
This is represented in the image below:
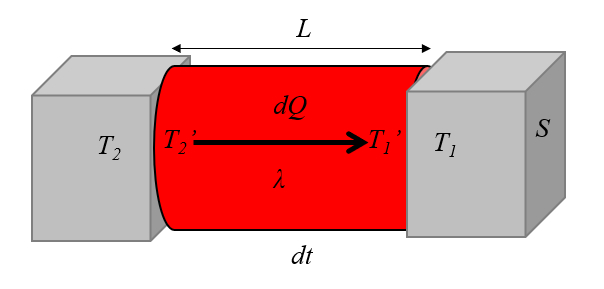
ID:(1675, 0)
Temperature Profile
Concept
Typically, the temperature variation within a conductor follows a linear pattern. However, in the case of gaseous and/or liquid media in contact with the conductor, there is a gradual temperature variation from the center of the medium to the surface, as depicted in the following image:
the outer surface temperature ($T_{es}$) depends on the outdoor Temperature ($T_e$), the coefficient of total transportation ($k$), the external transmission coefficient ($\alpha_e$), and the temperature difference ($\Delta T$):
| $ T_{es} = T_e + \displaystyle\frac{ k }{ \alpha_e } \Delta T $ |
the inner surface temperature ($T_{is}$) is a function of the indoor temperature ($T_i$) and the internal transmission coefficient ($\alpha_i$):
| $ T_{is} = T_i - \displaystyle\frac{ k }{ \alpha_i } \Delta T $ |
and the temperature difference ($\Delta T$):
| $ \Delta T = T_i - T_e $ |
ID:(7722, 0)
Total heat flow transportation
Concept
When the material includes multiple conductors connected in series, the total transport coefficient (multiple medium, two interfaces) ($k$) is calculated from the external transmission coefficient ($\alpha_e$), the internal transmission coefficient ($\alpha_i$), the thermal conductivity element i ($\lambda_i$), and the element length i ($L_i$) using the equation:
| $\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_i }+\displaystyle\frac{1}{ \alpha_e }+\sum_i\displaystyle\frac{ L_i }{ \lambda_i }$ |
This process is illustrated in the following diagram:
ID:(7721, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_i }+\displaystyle\frac{1}{ \alpha_e }+\displaystyle\frac{ L }{ \lambda }$
1/ k =1/ alpha_i + 1/ alpha_e + L / lambda
$ \Delta T = \Delta T_i + \Delta T_0 + \Delta T_e $
DT = DT_i + DT_0 + DT_e
$ \Delta T = T_i - T_e $
DT = T_i - T_e
$ \Delta T_0 = T_{is} - T_{es} $
DT_0 = T_is - T_es
$ \Delta T_e = T_{es} - T_e $
DT_e = T_es - T_e
$ \Delta T_i = T_i - T_{is} $
DT_i = T_i - T_is
$ q = \alpha_e \Delta T_e $
q = alpha_e * DT_e
$ q = \alpha_i \Delta T_i $
q = alpha_i * DT_i
$ q \equiv \displaystyle\frac{1}{ S }\displaystyle\frac{ dQ }{ dt }$
q = dQ /( S * dt )
$ q = k \Delta T $
q = k * DT
$ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $
q = lambda * DT_0 / L
$ T_{es} = T_e + \displaystyle\frac{ k }{ \alpha_e } \Delta T $
T_es = T_e + k * DT / alpha_e
$ T_{is} = T_i - \displaystyle\frac{ k }{ \alpha_i } \Delta T $
T_is = T_i - k * DT / alpha_i
ID:(15336, 0)
Temperature difference
Equation
The temperature difference ($\Delta T$) is calculated by subtracting the outdoor temperature ($T_e$) and the indoor temperature ($T_i$), which is expressed as:
| $ \Delta T = T_i - T_e $ |
ID:(15116, 0)
Temperature difference conductor to medium
Equation
The temperature difference at external interface ($\Delta T_e$) is calculated by subtracting the outer surface temperature ($T_{es}$) from the outdoor temperature ($T_e$):
| $ \Delta T_e = T_{es} - T_e $ |
ID:(15118, 0)
Medium to conductor temperature difference
Equation
The temperature difference at internal interface ($\Delta T_i$) is calculated by subtracting the inner surface temperature ($T_{is}$) from the indoor temperature ($T_i$):
| $ \Delta T_i = T_i - T_{is} $ |
ID:(15117, 0)
Surface temperature difference
Equation
In the case of a solid, and similarly for a liquid, we can describe the system as a structure of atoms held together by something that behaves like a spring. When both ends have temperatures of ($$), with the inner surface temperature ($T_{is}$) and the outer surface temperature ($T_{es}$):
| $ \Delta T_0 = T_{is} - T_{es} $ |
ID:(15120, 0)
Total temperature variation
Equation
In the process of heat transfer, the temperature gradually decreases from the system with the highest temperature (internal) to the one with the lowest temperature (external). In this process, it first decreases from the internal average temperature to the temperature difference at internal interface ($\Delta T_i$), then to the temperature difference in the conductor ($\Delta T_0$), and finally to the temperature difference at external interface ($\Delta T_e$). The sum of these three variations equals the total drop, that is, the temperature difference ($\Delta T$), as shown below:
| $ \Delta T = \Delta T_i + \Delta T_0 + \Delta T_e $ |
ID:(15115, 0)
Calculation of heat conduction
Equation
The heat flux ($q$) is a function of the thermal conductivity ($\lambda$), the conductor length ($L$) and the temperature difference in the conductor ($\Delta T_0$):
| $ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $ |
ID:(7712, 0)
Calculation of the total heat transport by a conductor
Equation
In this way, we establish a relationship that allows us to calculate the heat flow rate ($q$) as a function of the total transport coefficient (multiple medium, two interfaces) ($k$), and the temperature difference ($\Delta T$):
| $ q = k \Delta T $ |
With the temperature difference at internal interface ($\Delta T_i$), the temperature difference in the conductor ($\Delta T_0$), the temperature difference at external interface ($\Delta T_e$), and the temperature difference ($\Delta T$), we obtain
| $ \Delta T = \Delta T_i + \Delta T_0 + \Delta T_e $ |
which can be rewritten with the heat transported ($dQ$), the time variation ($dt$), the section ($S$)
| $ q = \alpha_i \Delta T_i $ |
| $ q = \alpha_e \Delta T_e $ |
and with the thermal conductivity ($\lambda$) and the conductor length ($L$)
| $ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $ |
and
| $\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_i }+\displaystyle\frac{1}{ \alpha_e }+\displaystyle\frac{ L }{ \lambda }$ |
as
$\Delta T = \Delta T_i + \Delta T_0 + \Delta T_e = \displaystyle\frac{1}{S} \frac{dQ}{dt} \left(\displaystyle\frac{1}{\alpha_i} + \displaystyle\frac{1}{\alpha_e} + \displaystyle\frac{L}{\lambda}\right) = \displaystyle\frac{1}{Sk} \displaystyle\frac{dQ}{dt}$
resulting in
| $ q = k \Delta T $ |
ID:(7716, 0)
Calculation of heat transmission to the conductor
Equation
In this way, we establish a relationship that allows us to calculate the heat flow rate ($q$) based on the temperature difference at internal interface ($\Delta T_i$), and the internal transmission coefficient ($\alpha_i$):
| $ q = \alpha_i \Delta T_i $ |
ID:(15113, 0)
Calculation of heat transfer from the conductor
Equation
In this manner, we establish a relationship that enables us to calculate the heat flow rate ($q$) based on the temperature difference at external interface ($\Delta T_e$), and the external transmission coefficient ($\alpha_e$):
| $ q = \alpha_e \Delta T_e $ |
ID:(15114, 0)
Temperature on the external surface of the conductor
Equation
The outer surface temperature ($T_{es}$) is not equal to the temperature of the medium, which is the outdoor temperature ($T_e$). This temperature can be calculated from the temperature difference ($\Delta T$), the total transport coefficient (multiple medium, two interfaces) ($k$), and the external transmission coefficient ($\alpha_e$) using the following formula:
| $ T_{es} = T_e + \displaystyle\frac{ k }{ \alpha_e } \Delta T $ |
With the heat transported ($dQ$), the time variation ($dt$), the section ($S$), the temperature difference ($\Delta T$), and the total transport coefficient (multiple medium, two interfaces) ($k$), we obtain
| $ q = k \Delta T $ |
which, with the external transmission coefficient ($\alpha_e$) and the temperature difference at external interface ($\Delta T_e$)
| $ q = \alpha_e \Delta T_e $ |
results in
$k\Delta T = \alpha_e \Delta T_e$
and with the outdoor temperature ($T_e$) and the outer surface temperature ($T_{es}$) and
| $ \Delta T_e = T_{es} - T_e $ |
results in
| $ T_{es} = T_e + \displaystyle\frac{ k }{ \alpha_e } \Delta T $ |
ID:(15122, 0)
Temperature on the inner surface of the conductor
Equation
The inner surface temperature ($T_{is}$) is not equal to the temperature of the medium itself, which is the indoor temperature ($T_i$). This temperature can be calculated from the temperature difference ($\Delta T$), the total transport coefficient (multiple medium, two interfaces) ($k$), and the internal transmission coefficient ($\alpha_i$) using the following formula:
| $ T_{is} = T_i - \displaystyle\frac{ k }{ \alpha_i } \Delta T $ |
With the heat transported ($dQ$), the time variation ($dt$), the section ($S$), the temperature difference ($\Delta T$), and the total transport coefficient (multiple medium, two interfaces) ($k$), we have
| $ q = k \Delta T $ |
which, with the internal transmission coefficient ($\alpha_i$) and the temperature difference at internal interface ($\Delta T_i$)
| $ q = \alpha_i \Delta T_i $ |
results in
$k\Delta T = \alpha_i \Delta T_i$
and with the indoor temperature ($T_i$) and the inner surface temperature ($T_{is}$) and
| $ \Delta T_i = T_i - T_{is} $ |
results in
| $ T_{is} = T_i - \displaystyle\frac{ k }{ \alpha_i } \Delta T $ |
ID:(15121, 0)
Total transportation coefficient (one medium, two interfaces)
Equation
The value of the coefficient of total transportation ($k$) in the transport equation is determined using the external transmission coefficient ($\alpha_e$), the internal transmission coefficient ($\alpha_i$), the thermal conductivity ($\lambda$), and the conductor length ($L$) as follows:
| $\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_i }+\displaystyle\frac{1}{ \alpha_e }+\displaystyle\frac{ L }{ \lambda }$ |
With the temperature difference at internal interface ($\Delta T_i$), the temperature difference in the conductor ($\Delta T_0$), the temperature difference at external interface ($\Delta T_e$), and the temperature difference ($\Delta T$), we obtain
| $ \Delta T = \Delta T_i + \Delta T_0 + \Delta T_e $ |
which can be rewritten with the heat transported ($dQ$), the time variation ($dt$), the section ($S$)
| $ q = \alpha_i \Delta T_i $ |
| $ q = \alpha_e \Delta T_e $ |
and with the thermal conductivity ($\lambda$) and the conductor length ($L$)
| $ q = \displaystyle\frac{ \lambda }{ L } \Delta T_0 $ |
as
$\Delta T_i + \Delta T_0 + \Delta T_e = \displaystyle\frac{1}{S} \displaystyle\frac{dQ}{dt} \left(\displaystyle\frac{1}{\alpha_i} + \displaystyle\frac{1}{\alpha_e} + \displaystyle\frac{L}{\lambda}\right)$
so we can define a combined coefficient as
| $\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_i }+\displaystyle\frac{1}{ \alpha_e }+\displaystyle\frac{ L }{ \lambda }$ |
ID:(3486, 0)
Heat flux density
Equation
The heat flow rate ($q$) is defined in terms of the heat transported ($dQ$), the time variation ($dt$), and the section ($S$) as follows:
| $ q \equiv \displaystyle\frac{1}{ S }\displaystyle\frac{ dQ }{ dt }$ |
ID:(15133, 0)