User:
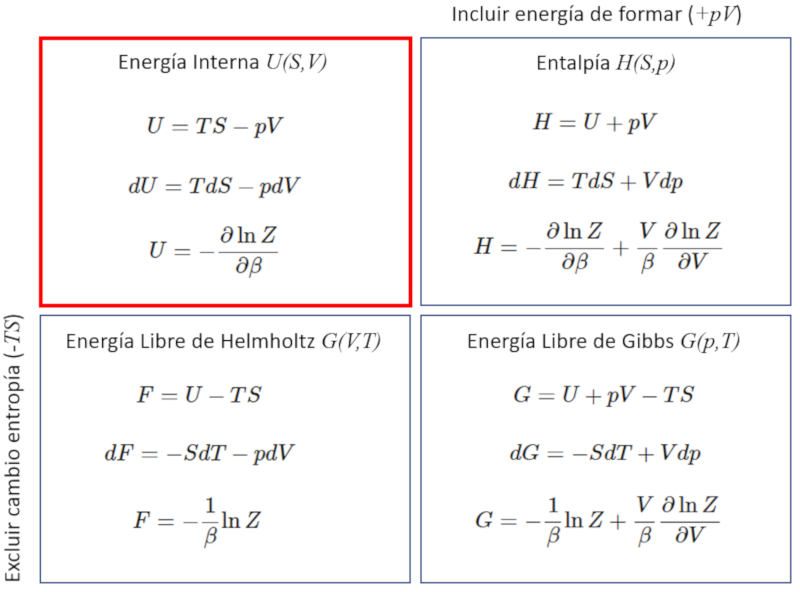
Internal energy and partition function
Image
The internal energy is allowed to calculate from the partition function as the derivative with respect to
ID:(11723, 0)
Internal Energy
Equation
If the absolute temperature ($T$) and the pressure ($p$) are held constant, the the variation of the Internal Energy ($dU$), which depends on the entropy variation ($dS$) and the volume Variation ($dV$),
| $ dU = T dS - p dV $ |
can be integrated, resulting in the expression for the internal energy ($U$) in terms of the entropy ($S$) and the volume ($V$):
 $ U = T S - p V $ |
ID:(3472, 0)
Internal Energy: Differential ratio
Equation
As the internal energy differential ($dU$) depends on the differential inexact Heat ($\delta Q$), the pressure ($p$), and the volume Variation ($dV$) according to the equation:
| $ dU = \delta Q - p dV $ |
we can replace the differential inexact Heat ($\delta Q$) with the expression from the second law of thermodynamics in terms of the absolute temperature ($T$) and the entropy variation ($dS$), resulting in the expression for the internal energy differential ($dU$):
| $ dU = T dS - p dV $ |
As the internal energy differential ($dU$) depends on the differential inexact Heat ($\delta Q$), the pressure ($p$), and the volume Variation ($dV$) according to the equation:
| $ dU = \delta Q - p dV $ |
and the expression for the second law of thermodynamics with the absolute temperature ($T$) and the entropy variation ($dS$) as:
| $ \delta Q = T dS $ |
we can conclude that:
| $ dU = T dS - p dV $ |
ID:(3471, 0)
Differential of internal energy
Equation
Since the internal energy is a function of volume and entropy, we can calculate its differential in terms of the differentials of volume and entropy. Therefore, we have:
| $ dU = DU_{S,V} dS + DU_{V,S} dV $ |
where we have defined:
| $ DU_{S,V} =\left(\displaystyle\frac{\partial U }{\partial S }\right)_ V $ |
and
| $ DU_{V,S} =\left(\displaystyle\frac{\partial U }{\partial V }\right)_ S $ |
ID:(8185, 0)
Calculo de la derivada parcial de la energía interna en el volumen a entropía constante
Equation
La derivada de la energía interna en el volumen a entropia constante es
| $ DU_{V,S} =\left(\displaystyle\frac{\partial U }{\partial V }\right)_ S $ |
ID:(12023, 0)
Calculo de la derivada parcial de la energía interna en la entropia a volumen constante
Equation
La derivada de la energía interna en la entropia a volumen constante es
| $ DU_{S,V} =\left(\displaystyle\frac{\partial U }{\partial S }\right)_ V $ |
ID:(12024, 0)
Internal energy and equation of state at constant entropy
Equation
The internal energy differential ($dU$) is a function of the variations in the entropy ($S$) and the volume ($V$), as well as the slopes the partial derivative of internal energy with respect to volume at constant entropy ($DU_{V,S}$) and the partial derivative of internal energy with respect to entropy at constant volume ($DU_{S,V}$), which is expressed as:
| $ dU = DU_{S,V} dS + DU_{V,S} dV $ |
Comparing this with the first law of thermodynamics, it turns out that the partial derivative of internal energy with respect to volume at constant entropy ($DU_{V,S}$) is equal to minus the pressure ($p$):
| $ DU_{V,S} =- p $ |
ID:(3535, 0)
Internal energy and equation of state at constant volume
Equation
The internal energy differential ($dU$) is a function of the variations in the entropy ($S$) and the volume ($V$), as well as the slopes the partial derivative of internal energy with respect to volume at constant entropy ($DU_{V,S}$) and the partial derivative of internal energy with respect to entropy at constant volume ($DU_{S,V}$), which is expressed as:
| $ dU = DU_{S,V} dS + DU_{V,S} dV $ |
Comparing this with the first law of thermodynamics, it turns out that the partial derivative of internal energy with respect to entropy at constant volume ($DU_{S,V}$) is equal to the absolute temperature ($T$):
| $ DU_{S,V} = T $ |
ID:(3546, 0)
Internal energy and partition function
Equation
La energía interna es igual a la energía media calculada con
| $\bar{E}=-\displaystyle\frac{\partial\ln Z}{\partial\beta}$ |
se tiene que con la energía interna es
| $U=-\displaystyle\frac{\partial\ln Z}{\partial\beta}$ |
ID:(3534, 0)
Internal energy and its relation of Maxwell
Equation
Since the internal energy ($U$) is an exact differential, it means that you can vary the entropy ($S$) first and then the volume ($V$), or in the reverse order, and the result will be the same. This can be expressed by taking derivatives of the slopes in different orders, and there will be no difference:
$D(DU_{S,V})_{V,S}=D(DU_{V,S})_{S,V}$
If you replace the differential with the corresponding variable, you obtain the relationship involving the absolute temperature ($T$) and the pressure ($p$):
| $ DT_{V,S} =- Dp_{S,V} $ |
Since the internal energy differential ($dU$) is an exact differential, we should note that the internal energy ($U$) with respect to the entropy ($S$) and the volume ($V$) must be independent of the order in which the function is derived:
$D(DU_{S,V}){V,S}=D(DU{V,S})_{S,V}$
Using the relationship between the slope the partial derivative of internal energy with respect to entropy at constant volume ($DU_{S,V}$) and the absolute temperature ($T$)
| $ DU_{S,V} = T $ |
,
and the relationship between the slope the partial derivative of internal energy with respect to volume at constant entropy ($DU_{V,S}$) and the pressure ($p$)
| $ DU_{V,S} =- p $ |
,
we can conclude that:
| $ DT_{V,S} =- Dp_{S,V} $ |
ID:(3556, 0)
Calculo de la derivada parcial de la temperatura en el volumen a entropía constante
Equation
La derivada de la temperatura en el volumen a entropia constante es
| $ DT_{V,S} =\left(\displaystyle\frac{\partial T }{\partial V }\right)_ S $ |
ID:(12025, 0)
Calculo de la derivada parcial de la presión en la entropia a volumen constante
Equation
La derivada de la presión en la entropia a volumen constante es
| $ Dp_{S,V} =\left(\displaystyle\frac{\partial p }{\partial S }\right)_ V $ |
ID:(12026, 0)
0
Video
Video: Internal energy