User:
Energía Libre de Gibbs
Storyboard
Se obtienen mediante la función partición las distintas funciones y relaciones termodinámicas.
ID:(443, 0)
Gibbs free energy with partition function
Image
To calculate the Gibbs function of the partition function, it is enough to see how the enthalpy and the entropy of it are constructed. How do you have to
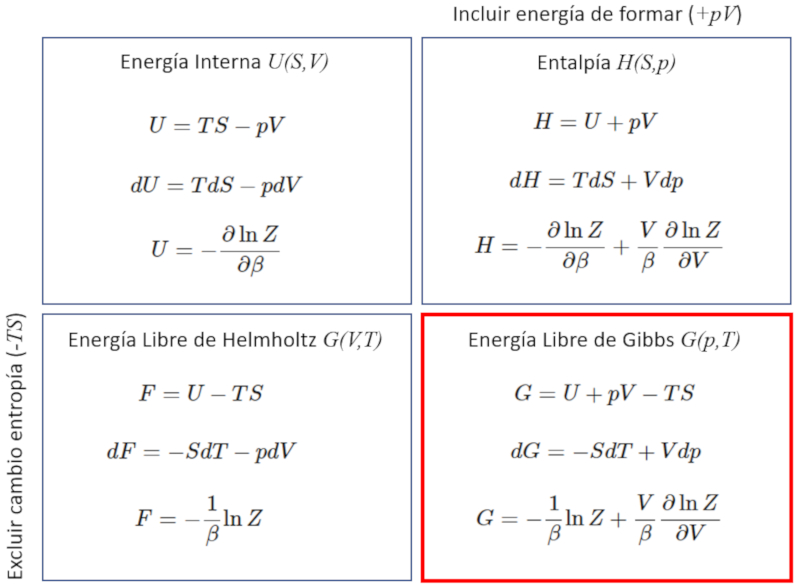
ID:(11726, 0)
Gibbs and Helmholtz free energy
Equation
The gibbs free energy ($G$) [1,2] represents the total energy, encompassing both the internal energy and the formation energy of the system. It is defined as the enthalpy ($H$), excluding the portion that cannot be used to perform work, which is represented by $TS$ with the absolute temperature ($T$) and the entropy ($S$). This relationship is expressed as follows:
 $ G = H - T S $ |
Where the absolute temperature ($T$) and the entropy ($S$) play a significant role.
[1] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 108-248 (October 1875 May 1876)
[2] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 343-524 (May 1877 July 1878)
ID:(3542, 0)
Gibbs free energy as differential
Equation
As the gibbs free energy ($G$) [1,2] depends on the enthalpy ($H$), the entropy ($S$), and the absolute temperature ($T$):
| $ G = H - T S $ |
The dependence on the gibbs free energy ($G$) with respect to the pressure ($p$) is obtained, and from the absolute temperature ($T$), we obtain the differential:
| $ dG =- S dT + V dp $ |
The gibbs free energy ($G$) as a function of the enthalpy ($H$), the entropy ($S$), and the absolute temperature ($T$) is expressed as:
| $ G = H - T S $ |
The value of the differential of the Gibbs free energy ($dG$) is determined using the differential enthalpy ($dH$), the temperature variation ($dT$), and the entropy variation ($dS$) through the equation:
$dG=dH-SdT-TdS$
Since the differential enthalpy ($dH$) is related to the volume ($V$) and the pressure Variation ($dp$) as follows:
| $ dH = T dS + V dp $ |
It follows that the differential enthalpy ($dH$), the entropy variation ($dS$), and the pressure Variation ($dp$) are interconnected in the following manner:
| $ dG =- S dT + V dp $ |
[1] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 108-248 (October 1875 May 1876)
[2] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 343-524 (May 1877 July 1878)
ID:(3541, 0)
Gibbs Free Energy Differential
Equation
Given that the gibbs free energy ($G$) is a function of the absolute temperature ($T$) and the pressure ($p$), we can express the differential of the Gibbs free energy ($dG$) as follows:
$dG=\left(\displaystyle\frac{\partial G}{\partial T}\right)_pdT+\left(\displaystyle\frac{\partial G}{\partial p}\right)_Tdp$
This allows us to define the differential of the Gibbs free energy ($dG$) in terms of the slopes the partial derivative of the Gibbs free energy with respect to pressure at constant temperature ($DG_{p,T}$) and the partial derivative of the Gibbs free energy with respect to temperature at constant pressure ($DG_{T,p}$):
| $ dG = DG_{T,p} dT + DG_{p,T} dp $ |
ID:(8188, 0)
Calculo de la derivada parcial de la energía libre de Gibbs en la temperatura a presión constante
Equation
La derivada de la energía interna en el volumen a entropia constante es
| $ DG_{T,p} =\left(\displaystyle\frac{\partial G }{\partial T }\right)_ p $ |
ID:(12418, 0)
Calculo de la derivada parcial de la energía libre de Gibbs en la presión a temperatura constante
Equation
La derivada de la energía interna en el volumen a entropia constante es
| $ DG_{p,T} =\left(\displaystyle\frac{\partial G }{\partial p }\right)_ T $ |
ID:(12419, 0)
Gibbs free energy and equation of state at constant pressure
Equation
The differential of the Gibbs free energy ($dG$) is a function of the variations in the absolute temperature ($T$) and the pressure ($p$), as well as the slopes the partial derivative of the Gibbs free energy with respect to temperature at constant pressure ($DG_{T,p}$) and the partial derivative of the Gibbs free energy with respect to pressure at constant temperature ($DG_{p,T}$), which is expressed as:
| $ dG = DG_{T,p} dT + DG_{p,T} dp $ |
Comparing this with the first law of thermodynamics, it turns out that the partial derivative of the Gibbs free energy with respect to temperature at constant pressure ($DG_{T,p}$) is equal to minus the entropy ($S$):
| $ DG_{T,p} =- S $ |
ID:(3552, 0)
Gibbs Free Energy and Equation of State by Constant Temperature
Equation
The differential of the Gibbs free energy ($dG$) is a function of the variations in the absolute temperature ($T$) and the pressure ($p$), as well as the slopes the partial derivative of the Gibbs free energy with respect to temperature at constant pressure ($DG_{T,p}$) and the partial derivative of the Gibbs free energy with respect to pressure at constant temperature ($DG_{p,T}$), which is expressed as:
| $ dG = DG_{T,p} dT + DG_{p,T} dp $ |
Comparing this with the first law of thermodynamics, it turns out that the partial derivative of the Gibbs free energy with respect to pressure at constant temperature ($DG_{p,T}$) is equal to the volume ($V$):
| $ DG_{p,T} = V $ |
ID:(3553, 0)
Gibbs free energy with partition function
Equation
Para calcular la función de Gibbs de la función partición basta ver como se construye la entalpía y la entropía de esta misma. Como se tiene que con absolute temperature $K$, enthalpy $J$, entropy $J/K$ and gibbs free energy $J$
| $ G = H - T S $ |
con
| $ H =-\displaystyle\frac{\partial \ln Z }{\partial \beta }+\displaystyle\frac{ V }{ \beta }\displaystyle\frac{\partial \ln Z }{\partial V }$ |
con
| $ S = k_B ( \ln Z + \beta U )$ |
y con
| $ k_B T \equiv\displaystyle\frac{1}{ \beta }$ |
se tiene que con
| $ G =-\displaystyle\frac{1}{ \beta }\ln Z +\displaystyle\frac{ V }{ \beta }\displaystyle\frac{\partial\ln Z }{\partial V }$ |
ID:(3543, 0)
Gibbs free energy and its Maxwell relation
Equation
Since the gibbs free energy ($G$) is an exact differential, it means that you can first vary the absolute temperature ($T$) and then the pressure ($p$), or in the reverse order, and the result will be the same. This can be expressed by taking derivatives of slopes in different orders, and there will be no difference:
$D(DG_{T,p})_{p,T}=D(DG_{p,T})_{T,p}$
If you replace the differential with the corresponding variable, you obtain the relationship involving the entropy ($S$) and the volume ($V$):
| $ DS_{p,T} = -DV_{T,p} $ |
Since the differential of the Gibbs free energy ($dG$) is an exact differential, it implies that the gibbs free energy ($G$) with respect to the absolute temperature ($T$) and the pressure ($p$) must be independent of the order in which the function is derived:
$D(DG_{T,p}){p,T}=D(DG{p,T})_{T,p}$
Using the relationship for the slope the partial derivative of the Gibbs free energy with respect to pressure at constant temperature ($DG_{p,T}$) with respect to the volume ($V$)
| $ DG_{p,T} = V $ |
,
and the relationship for the slope the partial derivative of the Gibbs free energy with respect to temperature at constant pressure ($DG_{T,p}$) with respect to the entropy ($S$)
| $ DG_{T,p} =- S $ |
,
we can conclude that:
| $ DS_{p,T} = -DV_{T,p} $ |
ID:(3557, 0)
Calculo de la derivada parcial de la entropía en la presión a temperatura constante
Equation
La derivada de la entropía en la presión a temperatura constante es
| $ DS_{p,T} =\left(\displaystyle\frac{\partial S }{\partial p }\right)_ T $ |
ID:(12423, 0)
Calculo de la derivada parcial del volumen en la temperatura a presión constante
Equation
La derivada el volumen en la temperatura a presión constante es
| $ DV_{T,p} =\left(\displaystyle\frac{\partial V }{\partial T }\right)_ p $ |
ID:(12421, 0)
0
Video
Video: Gibbs Free Energy