User:
Application in Microscopes
Storyboard 
The principle of the microscope is to capture the small image, which above as parallel beams and enlarge it with a biconvex lens to form a larger inverted real image.
ID:(299, 0)
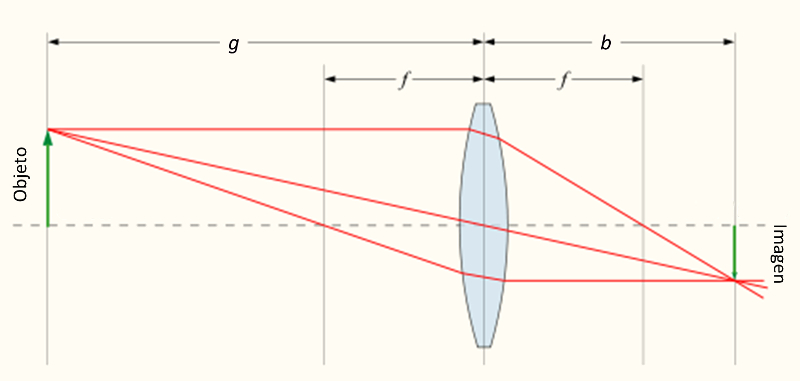
Beam geometry in a lens, near object
Image
In the event that the object is closer to the lens than the focal point, the diagram to determine image size and position is somewhat more complex. In this case the beams must be
projected from where they would have reached the object that radiates them
within the projection the same rules as in a real beam must be followed
In this case it is enough to diagram the same three beams again:
- parallel to the optical axis is refracted by the focus
- via the focus is refracted parallel to the optical axis
- via the origin of the continuous optical axis in a straight line
and the image is obtained in the same way:
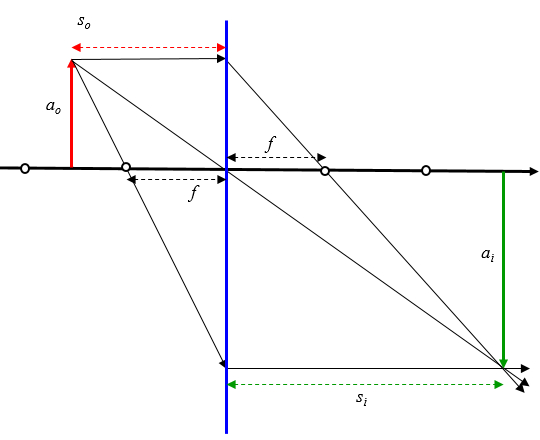
ID:(9783, 0)
Geometry of the Beams on a Lens
Image
In the case of a biconvex lens a beam that reaches the lens
- parallel to the optical axis is refracted by the focus
- via the focus is refracted parallel to the optical axis
- via the origin of the continuous optical axis in a straight line
what in the case of an object at a distance greater than the photo corresponds to:
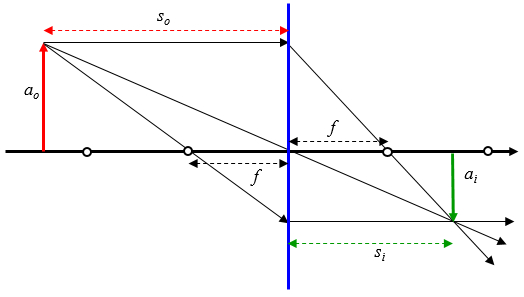
ID:(1856, 0)
Convex Lens
Image
A convex lens is a lens that refracts the parallel beam of light that strikes parallel through its focus:
ID:(1855, 0)
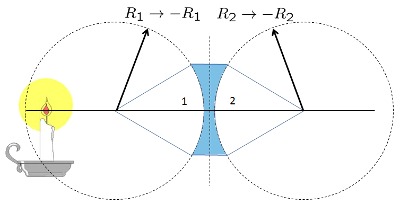
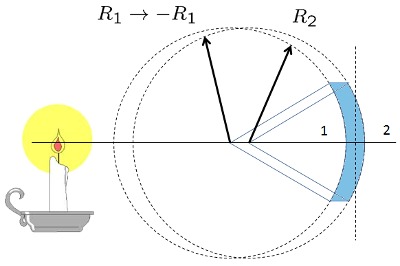
Position and focus of concave lens
Equation
Por similitud de los triángulos de los tamaños del objeto y la imagen y las posiciones del objeto y foco permite por similitud de triángulos mostrar que:
 $\displaystyle\frac{1}{ f_{lc} }=\displaystyle\frac{1}{ s_o }+\displaystyle\frac{1}{ s_{lc} }$ |
Una relación se puede armar con los triángulos del lado del objeto. En este caso la similitud nos permite escribir que el tamaño del objeto
$\displaystyle\frac{a_o}{s_o-f}=\displaystyle\frac{a_i}{f}$
Con la relación de similitud de los triángulos
| $\displaystyle\frac{ a_o }{ a_{lc} }=\displaystyle\frac{ s_o }{ s_{lc} }$ |
se puede mostrar que se cumple:
| $\displaystyle\frac{1}{ f_{lc} }=\displaystyle\frac{1}{ s_o }+\displaystyle\frac{1}{ s_{lc} }$ |
ID:(3347, 0)
Proportions size and position of concave lens
Equation
For any lens you can draw characteristic beams with which you can similarly show that the sizes of the object and the image are in the same proportion as their distances to the optical element (lens or mirror).
If the object has a size
| $\displaystyle\frac{ a_o }{ a_{lc} }=\displaystyle\frac{ s_o }{ s_{lc} }$ |
ID:(3346, 0)
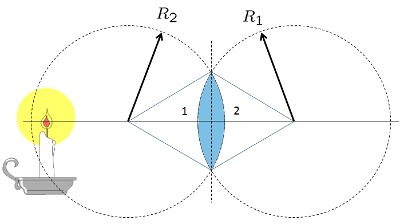
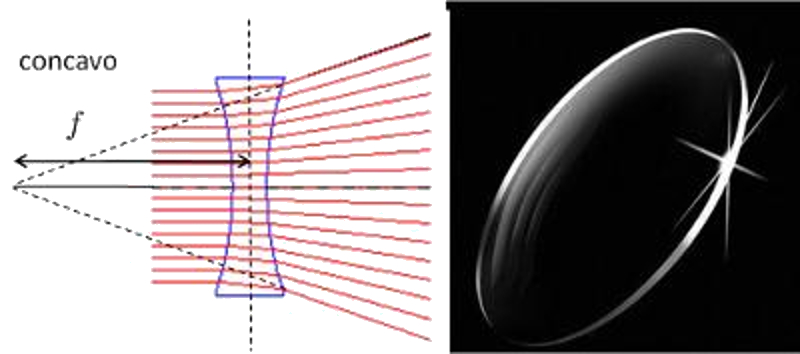
Concave Lens
Image
Convex lenses are thinner in their center widening towards the edges.
The light beams that have a parallel impact are scattered as if the light were emitted in the lens focus.
ID:(1854, 0)
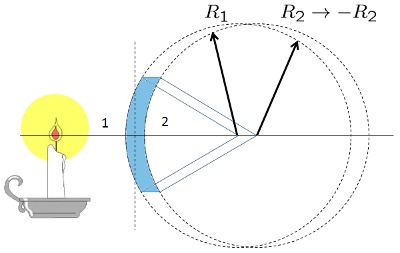
Calculating the focus of a Bi-Convex Simple Lens
Equation
Una caso especial es aquel en que los radios son iguales, o sea
| $\displaystyle\frac{1}{ f_{vsd} }=( n -1)\left(\displaystyle\frac{2}{ R }-\displaystyle\frac{( n -1) d }{ n R ^2}\right)$ |
ID:(3432, 0)
Calculating the Focus of a bi-convex thickness Lens
Equation
Los lentes reales tienen un grosor que se debe considerar. Si el lente tiene un indice de refracción
| $\displaystyle\frac{1}{ f_{vvd} }=( n -1)\left(\displaystyle\frac{1}{ R_1 }+\displaystyle\frac{1}{ R_2 }-\displaystyle\frac{( n -1)d}{ n R_1 R_2 }\right)$ |
ID:(3348, 0)
Cálculo del foco de un lente convexo-concavo grueso simétrico
Equation
Una caso especial es aquel en que los radios son iguales, o sea
| $\displaystyle\frac{1}{ f_{vcs} }=\displaystyle\frac{( n -1)^2 d }{ n R ^2}$ |
ID:(3430, 0)
Cálculo del foco de un lente convexo-cóncavo grueso
Equation
Los lentes reales tienen un grosor que se debe considerar. Si el lente tiene vidrio con indice de refracción
| $\displaystyle\frac{1}{ f_{vcs} }=( n -1)\left(\displaystyle\frac{1}{ R_1 }-\displaystyle\frac{1}{ R_2 }+\displaystyle\frac{( n -1) d }{ n R_1 R_2 }\right)$ |
ID:(3350, 0)
Calculating the Focus of a Simple Bi-Concave Lens
Equation
Una caso especial es aquel en que los radios son iguales, o sea
| $\displaystyle\frac{1}{ f_{csd} }=-( n -1)\left(\displaystyle\frac{2}{ R } +\displaystyle\frac{( n -1) d }{ n R ^2}\right)$ |
ID:(3431, 0)
Cálculo del foco de un lente concavo-convexo grueso simétrico
Equation
Una caso especial es aquel en que los radios son iguales, o sea
| $\displaystyle\frac{1}{ f_{cvs} }=\displaystyle\frac{( n -1)^2 d }{ n R ^2}$ |
ID:(3429, 0)
Calculating the Focus of a bi-concave thickness Lens
Equation
Los lentes reales tienen un grosor que se debe considerar. Si el lente tiene vidrio con indice de refracción
| $\displaystyle\frac{1}{ f_{ccd} }=-( n -1)\left(\displaystyle\frac{1}{ R_1 }+\displaystyle\frac{1}{ R_2 }+\displaystyle\frac{( n -1)d}{ n R_1 R_2 }\right)$ |
ID:(3349, 0)
Multiples lentes
Image
Cuando se acoplan dos lentes con sus respectivos focos, el primer lente genera una imagen que funciona como objeto para el segundo lente que a su vez genera una imagen de una imagen:
ID:(9465, 0)
Refraction depending on the color of light
Image
The refractive index of glass can depend on the wavelength or frequency of light. In such cases, the glass is referred to as 'chromatic.' If it does not exhibit this property, it is called 'achromatic.'
The main issue with this property is that the focal point of a lens depends on the color of light. Therefore, an optical lens has the problem that if the eye can focus on one color, it will not be able to simultaneously focus on objects of other colors.
ID:(1626, 0)