User:
Bernoulli with hydrostatic pressure
Storyboard 
If we consider a fluid without viscosity and turbulence (laminar flow), we can assume that energy is conserved and flows with the liquid (or gas). In these cases, we obtain an equation that states that the sum of the density of kinetic energy and the density of potential energy are constant.
This allows us to calculate how velocity evolves as a function of position as long as the existing pressure or any force field is known.
The only problem is that most media have significant viscosity and therefore tend not to have turbulence or it is negligible, and the flow is intrinsically turbulent. Therefore, the application of Bernoulli's law in this sense is restricted, or rather, it is a first approximation.
ID:(684, 0)
Mechanisms
Iframe
Mechanisms
ID:(15486, 0)
Movement of a liquid/gas element with the flow
Concept
If we envision the flow as a series of volumes with sides $\Delta x$, $\Delta y$, and $\Delta z$ moving within the stream, we can assume that the energy it contains remains constant. This means that if we calculate the energy density at any point, it will always be the same.
ID:(11097, 0)
Kinetic energy of the element in the flow
Concept
If the medium has a density of $\rho$, the mass of the volume $\Delta x\Delta y\Delta z$ can be calculated as:
$m=\rho\Delta x\Delta y\Delta z$
From this, we can estimate the kinetic energy of the element using the velocity $v$:
$\displaystyle\frac{1}{2}m v^2=\displaystyle\frac{1}{2}\rho\Delta x\Delta y\Delta z v^2$
This can be visualized in the following image:
Therefore the density of the kinetic energy is
$\displaystyle\frac{m v^2}{2 \Delta x\Delta y\Delta z}=\displaystyle\frac{1}{2}\rho v^2$
ID:(11101, 0)
Gravitational potential energy of the element in the flow
Concept
If the medium has a density of $\rho$ the mass of the volume $\Delta x\Delta y\Delta z$ can be calculated as
$m=\rho\Delta x\Delta y\Delta z$
with what you can estimate with the height $h$ the gravitational potential energy of the element
$mgh=\rho\Delta x\Delta y\Delta z gh$
what is displayed in
Therefore the density of the gravitational potential energy is
$\displaystyle\frac{mgh}{\Delta x\Delta y\Delta z}=\rho g h$
ID:(11102, 0)
General potential energy of the element in the flow
Concept
Suppose there is a force acting on the element, and if we orient the coordinate system such that this force acts in the x-direction, the force will be doing work given by:
$F\Delta x$
If the force is generated by pressure, then the force would act on the surface perpendicular to the force, that is, $\Delta y \Delta z$. Thus, the energy would be:
$F = p \Delta S = p \Delta y\Delta z$
This can be visualized in the following image:
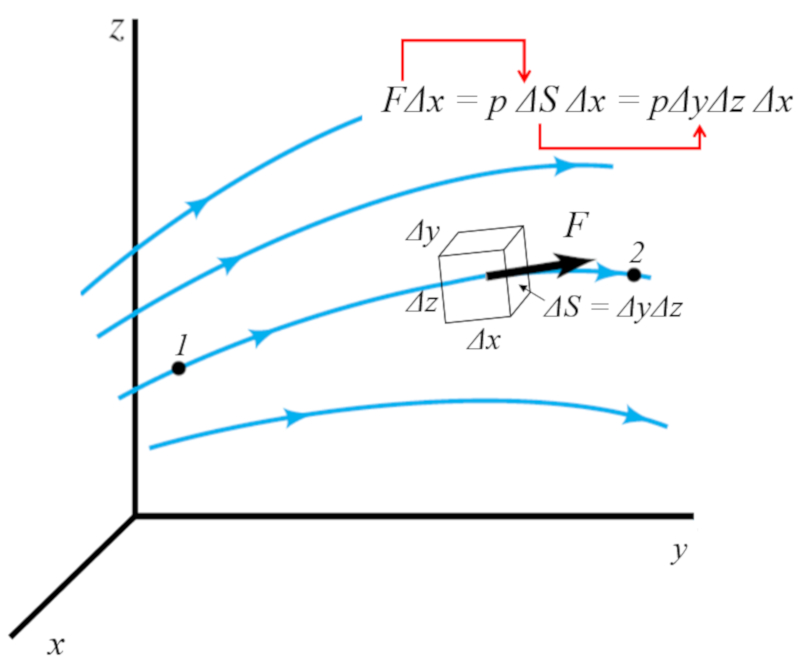
Therefore the density of the general energy is
$\displaystyle\frac{F \Delta x}{\Delta x\Delta y\Delta z}=\displaystyle\frac{p \Delta x\Delta y\Delta z}{\Delta x\Delta y\Delta z}=p$
ID:(11103, 0)
Energy density
Concept
The Bernoulli's hypothesis states that energy is conserved locally, meaning there are no mechanisms allowing a volume of the medium to exchange energy with its surroundings. If we consider the energy equation $E$, which includes:
• The kinetic energy as a function of mass $m$ and velocity the speed on a cylinder radio ($v$),
• The gravitational potential energy as a function of the gravitational Acceleration ($g$) and the column height ($h$), and
• An external force $F$ displacing the liquid by a distance $\Delta z$,
we can express it as follows:
$E=\displaystyle\frac{m}{2}v^2+mgh+F\Delta x$
If we consider the energy in a volume $\Delta x\Delta y\Delta z$, we can replace the mass with the density ($\rho$):
$m=\rho \Delta x\Delta y\Delta z$
And since the water column pressure ($p$) is expressed as:
$F=p \Delta S =p \Delta y\Delta z$
We obtain the equation for the energy density ($e$):
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
In the absence of viscosity, energy conservation implies that the energy density ($e$) is constant at any point in the fluid. Therefore, knowing the velocity and/or pressure at any location in the fluid is sufficient to establish a relationship between velocity and pressure at any point in the fluid.
ID:(15708, 0)
Bernoulli's law and its limits
Top
The hypothesis of Bernoulli's law is that energy, and thus the energy density ($e$), remains constant. In this case, the energy density is the sum of:
• Kinetic energy, which depends on the liquid density ($\rho_w$) and the speed on a cylinder radio ($v$)
• Gravitational potential energy, which depends on the gravitational Acceleration ($g$) and the column height ($h$)
• General potential energy, which depends on the pressure ($p$)
resulting in:
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
However, this limits the applicability of the law because:
• Viscosity is a process in which energy diffuses through the medium, and in this sense, energy is not locally conserved as it redistributes within the medium.
• Vortices cannot exist because they inherently present zones of different energy densities, thus contradicting the hypothesis. This means that it would not describe turbulent flow.
The problem is that in most cases, the flow can be dominated by viscosity, termed "laminar" flow, or dominated by inertia, resulting in "turbulent" flow. Therefore, Bernoulli's law is a model only applicable in situations where the inhomogeneity of energy density is lower.
ID:(15500, 0)
General Bernoulli equation
Concept
If we assume that the energy density ($e$) is conserved, we can state that for a cell where the average velocity is the speed on a cylinder radio ($v$), the density is the density ($\rho$), the pressure is the water column pressure ($p$), the height is the column height ($h$), and the gravitational acceleration is the gravitational Acceleration ($g$), the following holds:
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
At point 1, this equation will be equal to the same equation at point 2:
$e(v_1,p_1,h_1)=e(v_2,p_2,h_2)$
where the mean Speed of Fluid in Point 1 ($v_1$), the height or depth 1 ($h_1$), and the pressure in column 1 ($p_1$) represent the velocity, height, and pressure at point 1, respectively, and the mean Speed of Fluid in Point 2 ($v_2$), the height or depth 2 ($h_2$), and the pressure in column 2 ($p_2$) represent the velocity, height, and pressure at point 2, respectively.
Therefore, we have the Bernoulli equation [1]:
| $\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $ |
[1] "Hydrodynamica" (Hidrodinamica), Daniel Bernoulli, Typis Joh. Henr. Deckeri (1738)
It is important to bear in mind the following assumptions:
Energy is conserved, particularly assuming the absence of viscosity.
There is no deformation in the medium, hence the density remains constant.
There is no vorticity, meaning no swirling motion leading to circulation in the medium. The fluid must exhibit laminar behavior.
ID:(15707, 0)
Perfume dispenser
Description
Perfume dispensers operate by creating an airflow over a tube immersed in the perfume. This airflow causes a decrease in pressure, resulting in the pressure in the perfume column being lower than the column generated by the liquid inside the bottle. As a result, the liquid is propelled up through the column. Eventually, the liquid reaching the top is atomized and carried away by the air stream.
To model the system, one can use the Bernoulli's principle with the liquid density the liquid density ($\rho_w$) and the height the gravitational Acceleration ($g$). If point 1 is at the base of the liquid transport tube, then the mean Speed of Fluid in Point 1 ($v_1$) is null, the height or depth 1 ($h_1$) represents the depth of the liquid ($h$), and the pressure in column 1 ($p_1$) denotes the atmospheric pressure. If point 2 is at the upper outlet of the liquid transport tube, then the mean Speed of Fluid in Point 2 ($v_2$) represents the velocity at which the liquid emerges ($v$), the height or depth 2 ($h_2$) is null, and the pressure in column 2 ($p_2$) is the atmospheric pressure. Therefore, the expression
| $\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $ |
reduces to
$\rho g h=\displaystyle\frac{1}{2}\rho v^2 $
since atmospheric pressure simplifies. Thus, the velocity at which the liquid emerges is:
$v = \sqrt{ 2 g h }$
ID:(11096, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ \Delta h = h_2 - h_1 $
Dh = h_2 - h_1
$ \Delta p = p_2 - p_1 $
Dp = p_2 - p_1
$ \Delta p = \rho g \Delta h $
Dp = rho_w * g * Dh
$ \Delta v = v_2 - v_1 $
Dv = v_2 - v_1
$ e_1 =\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 $
e = rho * v ^ 2 / 2 + rho * g * h + p
$ e_2 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $
e = rho * v ^ 2 / 2 + rho * g * h + p
$ e_1 = e_2 $
e_1 = e_2
$\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $
rho * v_1 ^2/2+ rho * g * h_1 + p_1 = rho * v_2 ^2/2+ rho * g * h_2 + p_2
ID:(15489, 0)
Conservation of energy density
Equation
If energy is conserved within the flowing volumes, then the energy density in 1 ($e_1$) and the energy density in 2 ($e_2$) must be equal:
| $ e_1 = e_2 $ |
This is only possible if viscosity is negligible, as it is associated with energy diffusion, and there are no vortices present, which themselves exhibit energy differences due to varying tangential velocities along the vortex radius.
ID:(15499, 0)
Energy density (1)
Equation
Since a fluid or gas is a continuum, the concept of energy can no longer be associated with a specific mass. However, it is possible to consider the energy contained in a volume of the continuum, and by dividing it by the volume itself, we obtain the energy density ($e$). Therefore, with the density ($\rho$), the speed on a cylinder radio ($v$), the column height ($h$), the gravitational Acceleration ($g$), and the water column pressure ($p$), we have:
| $ e_1 =\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 $ |
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
Another useful equation is the one corresponding to the conservation of energy, which is applicable in cases where viscosity, a process that leads to energy loss, can be neglected. If we consider the classic energy equation $E$, which takes into account kinetic energy, gravitational potential energy, and an external force displacing the liquid over a distance $\Delta z$, it can be expressed as:
$E=\displaystyle\frac{m}{2}v^2+mgh+F\Delta x$
If we consider the energy within a volume $\Delta x\Delta y\Delta z$, we can replace the mass with:
$m=\rho \Delta x\Delta y\Delta z$
And since pressure is given by:
$F=p \Delta S =p \Delta y\Delta z$
We obtain the equation for energy density:
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
which corresponds to the Bernoulli equation.
ID:(3159, 1)
Energy density (2)
Equation
Since a fluid or gas is a continuum, the concept of energy can no longer be associated with a specific mass. However, it is possible to consider the energy contained in a volume of the continuum, and by dividing it by the volume itself, we obtain the energy density ($e$). Therefore, with the density ($\rho$), the speed on a cylinder radio ($v$), the column height ($h$), the gravitational Acceleration ($g$), and the water column pressure ($p$), we have:
| $ e_2 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $ |
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
Another useful equation is the one corresponding to the conservation of energy, which is applicable in cases where viscosity, a process that leads to energy loss, can be neglected. If we consider the classic energy equation $E$, which takes into account kinetic energy, gravitational potential energy, and an external force displacing the liquid over a distance $\Delta z$, it can be expressed as:
$E=\displaystyle\frac{m}{2}v^2+mgh+F\Delta x$
If we consider the energy within a volume $\Delta x\Delta y\Delta z$, we can replace the mass with:
$m=\rho \Delta x\Delta y\Delta z$
And since pressure is given by:
$F=p \Delta S =p \Delta y\Delta z$
We obtain the equation for energy density:
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
which corresponds to the Bernoulli equation.
ID:(3159, 2)
General Bernoulli equation
Equation
With the mean Speed of Fluid in Point 1 ($v_1$), the height or depth 1 ($h_1$), and the pressure in column 1 ($p_1$) representing the velocity, height, and pressure at point 1, respectively, and the mean Speed of Fluid in Point 2 ($v_2$), the height or depth 2 ($h_2$), and the pressure in column 2 ($p_2$) representing the velocity, height, and pressure at point 2, respectively, we have:
| $\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $ |
If we assume that the energy density ($e$) is conserved, we can state that for a cell where the average velocity is the speed on a cylinder radio ($v$), the density is the density ($\rho$), the pressure is the water column pressure ($p$), the height is the column height ($h$), and the gravitational acceleration is the gravitational Acceleration ($g$), the following holds:
| $ e =\displaystyle\frac{1}{2} \rho v ^2+ \rho g h + p $ |
At point 1, this equation will be equal to the same equation at point 2:
$e(v_1,p_1,h_1)=e(v_2,p_2,h_2)$
where the mean Speed of Fluid in Point 1 ($v_1$), the height or depth 1 ($h_1$), and the pressure in column 1 ($p_1$) represent the velocity, height, and pressure at point 1, respectively, and the mean Speed of Fluid in Point 2 ($v_2$), the height or depth 2 ($h_2$), and the pressure in column 2 ($p_2$) represent the velocity, height, and pressure at point 2, respectively. Therefore, we have:
| $\displaystyle\frac{1}{2} \rho v_1 ^2+ \rho g h_1 + p_1 =\displaystyle\frac{1}{2} \rho v_2 ^2+ \rho g h_2 + p_2 $ |
ID:(4504, 0)
Pressure difference between columns
Equation
The height difference, denoted by the height difference ($\Delta h$), implies that the pressure in both columns is distinct. In particular, the pressure difference ($\Delta p$) is a function of the liquid density ($\rho_w$), the gravitational Acceleration ($g$), and the height difference ($\Delta h$), as follows:
| $ \Delta p = \rho g \Delta h $ |
| $ \Delta p = \rho_w g \Delta h $ |
If there is the pressure difference ($\Delta p$) between two points, as determined by the equation:
| $ \Delta p = p_2 - p_1 $ |
we can utilize the water column pressure ($p$), which is defined as:
| $ p_t = p_0 + \rho_w g h $ |
This results in:
$\Delta p=p_2-p_1=p_0+\rho_wh_2g-p_0-\rho_wh_1g=\rho_w(h_2-h_1)g$
As the height difference ($\Delta h$) is:
| $ \Delta h = h_2 - h_1 $ |
the pressure difference ($\Delta p$) can be expressed as:
| $ \Delta p = \rho_w g \Delta h $ |
ID:(4345, 0)
Height difference
Equation
When two liquid columns are connected with the height of liquid column 1 ($h_1$) and the height of liquid column 2 ($h_2$), a the height difference ($\Delta h$) is formed, which is calculated as follows:
| $ \Delta h = h_2 - h_1 $ |
the height difference ($\Delta h$) will generate the pressure difference that will cause the liquid to flow from the higher column to the lower one.
ID:(4251, 0)
Pressure difference
Equation
When two liquid columns are connected with the pressure in column 1 ($p_1$) and the pressure in column 2 ($p_2$), a the pressure difference ($\Delta p$) is formed, which is calculated according to the following formula:
| $ \Delta p = p_2 - p_1 $ |
the pressure difference ($\Delta p$) represents the pressure difference that will cause the liquid to flow from the taller column to the shorter one.
ID:(4252, 0)
Speed difference
Equation
The speed difference between surfaces ($\Delta v$) is with the mean Speed of Fluid in Point 1 ($v_1$) and the mean Speed of Fluid in Point 2 ($v_2$) is
| $ \Delta v = v_2 - v_1 $ |
ID:(15502, 0)