User:
Ohm's Law
Storyboard 
When a field is applied to a charge, it generates a force. This force, when acting along a path, gives rise to potential energy. If this potential energy is expressed in terms of an electric field, it becomes the potential energy per unit charge, known as electric potential.
The electric potential induces the movement of charges, creating a flow called electric current. The magnitude of this current depends on both the applied electric potential and the resistance of the material through which the charges move, commonly referred to as the conductor.
The resulting relationship between electric potential, current, and resistance is described by the well-known Ohm's law.
ID:(815, 0)
Current by a conductor
Image
In summary, the application of a potential difference between the two ends of the
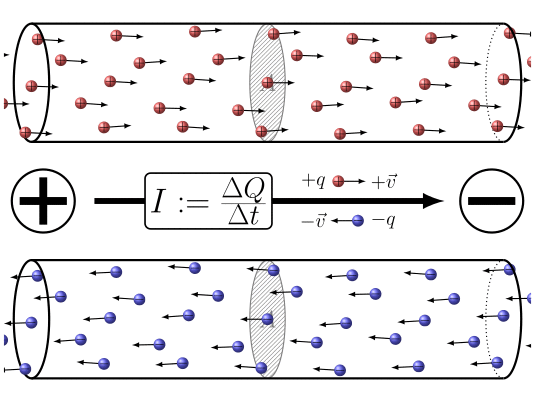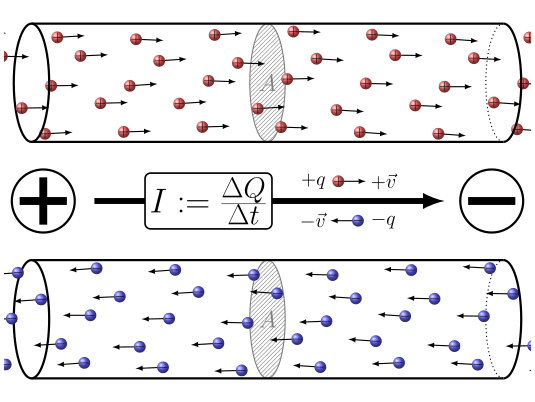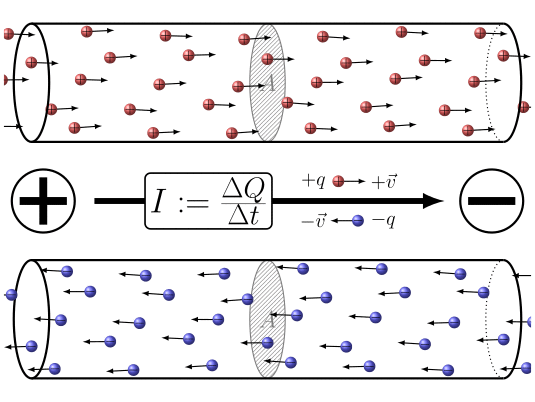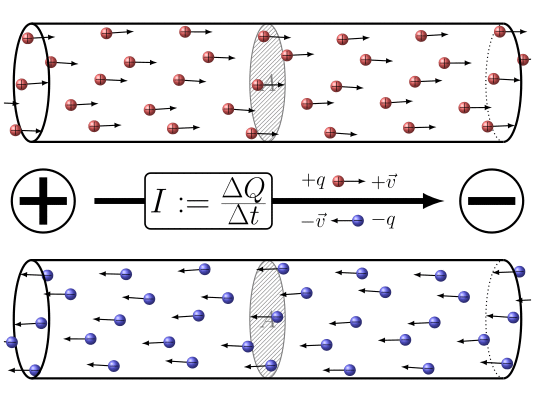
ID:(7860, 0)
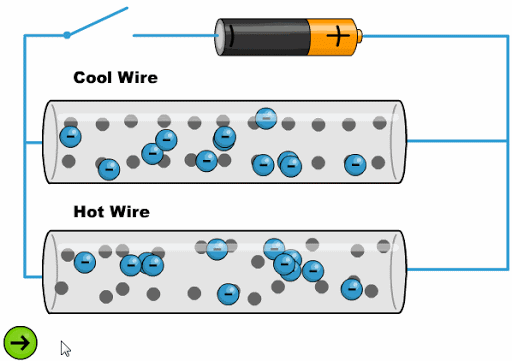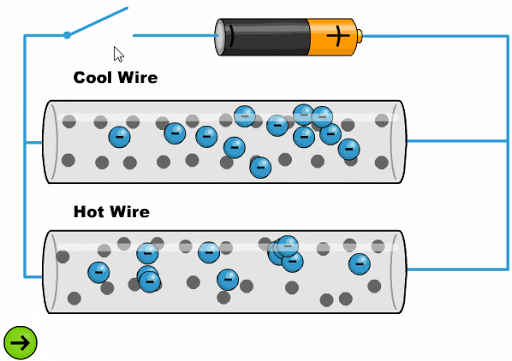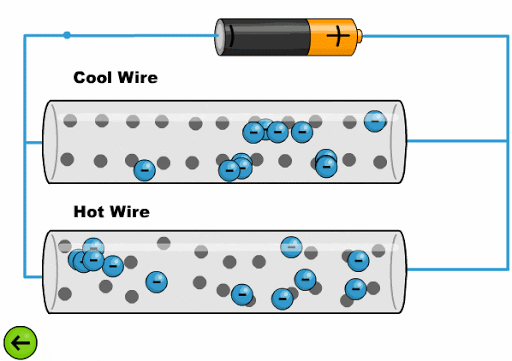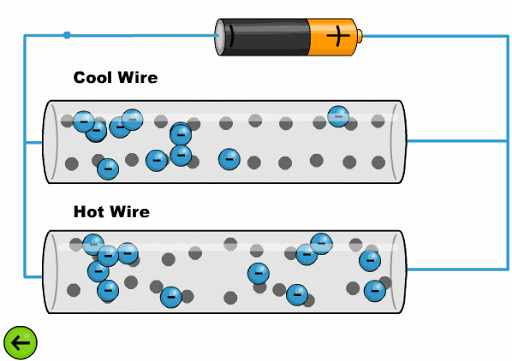
Resistance and heat
Image
The heat makes the atoms oscillate with a greater amplitude, making it difficult for the electrons to advance:
ID:(11761, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ a =\displaystyle\frac{ e E }{ m_e }$
a = e * E / m_e
$ \Delta\varphi = R I $
Dphi = R * I
$ \Delta\varphi =\displaystyle\frac{2 m_e }{ e ^2 \tau c }\displaystyle\frac{ L }{ S } I $
Dphi =(2 * m_e /( e ^2* tau * c ))*( L / S )* I
$ E =\displaystyle\frac{ \Delta\varphi }{ L }$
E = Dphi / L
$ I =\displaystyle\frac{ \Delta Q }{ \Delta t }$
I = DQ / Dt
$ I = e S c \bar{v} $
I = e * S * c * v_m
$ I =\displaystyle\frac{ e ^2 E }{2 m_e } \tau c S $
I = e ^2* E * tau * c * S /(2* m_e )
$ R = \rho_e \displaystyle\frac{ L }{ S }$
R = rho_e * L / S
$ \rho_e =\displaystyle\frac{2 m_e }{ e ^2 \tau c }$
rho_e =2* m_e /( e ^2* tau * c )
$ v_{max} =\displaystyle\frac{ e E }{ m_e } \tau $
v_max =( e * E/ m_e )* tau
ID:(16002, 0)
Charge flow
Equation
When electric charges move, it is possible to define a quantity the load element ($\Delta Q$), representing the amount of charge passing through a section over a time interval the time elapsed ($\Delta t$). This quantity is related to a current ($I$) and is defined by the following expression:
| $ I =\displaystyle\frac{ \Delta Q }{ \Delta t }$ |
None
ID:(10401, 0)
Field in the conductor
Equation
The electric eield ($E$) is generated by the potential difference ($\Delta\varphi$) between two electrodes, separated by a distance of a conductor length ($L$). This value can be calculated using the following expression:
| $ E =\displaystyle\frac{ \Delta\varphi }{ L }$ |
None
ID:(3838, 0)
Acceleration of electrons
Equation
The electric eield ($E$), together with the electron Charge ($e$), generates a force that, through the mass of the electron ($m_e$), results in the acceleration of charge in the conductor ($a$). This relationship can be expressed as:
| $ a =\displaystyle\frac{ e E }{ m_e }$ |
None
ID:(3843, 0)
Maximum speed of the electron
Equation
In a time between collisions ($\tau$), the electron is accelerated by the electric eield ($E$), in combination with the electron Charge ($e$) and the mass of the electron ($m_e$), until it reaches the maximum Speed ($v_{max}$). This process is described by the following relationship:
| $ v_{max} =\displaystyle\frac{ e E }{ m_e } \tau $ |
ID:(3836, 0)
Microscopic current
Equation
The current ($I$) can be calculated by considering electrons with a charge concentration ($c$) and the electron Charge ($e$), moving at a average speed of charges ($\bar{v}$) through a section of Conductors ($S$). This relationship is expressed as:
| $ I = e S c \bar{v} $ |
ID:(10400, 0)
Current as a function of electric field
Equation
The current ($I$) can be calculated from the electric eield ($E$), in combination with the electron Charge ($e$), the charge concentration ($c$), the mass of the electron ($m_e$), the time between collisions ($\tau$), and the section of Conductors ($S$), using the following relationship:
| $ I =\displaystyle\frac{ e ^2 E }{2 m_e } \tau c S $ |
ID:(3837, 0)
Microscopic Ohm's law
Equation
If the current ($I$) is expressed using the potential difference ($\Delta\varphi$) instead of the electric eield ($E$), the microscopic form of Ohm's law is obtained. This equation involves the electron Charge ($e$), the charge concentration ($c$), the mass of the electron ($m_e$), the time between collisions ($\tau$), the section of Conductors ($S$), and the conductor length ($L$), through the following relationship:
| $ \Delta\varphi =\displaystyle\frac{2 m_e }{ e ^2 \tau c }\displaystyle\frac{ L }{ S } I $ |
ID:(3839, 0)
Specific resistivity
Equation
From the microscopic form of Ohm's law, a factor specific to the material of the conductor can be identified. This allows the resistivity ($\rho_e$) to be defined in terms of the electron Charge ($e$), the charge concentration ($c$), the mass of the electron ($m_e$), and the time between collisions ($\tau$), using the following relationship:
| $ \rho_e =\displaystyle\frac{2 m_e }{ e ^2 \tau c }$ |
ID:(3840, 0)
Resistance
Equation
Using the resistivity ($\rho_e$) along with the geometric parameters the conductor length ($L$) and the section of Conductors ($S$), the resistance ($R$) can be defined through the following relationship:
| $ R = \rho_e \displaystyle\frac{ L }{ S }$ |
ID:(3841, 0)
Ohm's law
Equation
Traditional Ohm's law establishes a relationship between the potential difference ($\Delta\varphi$) and the current ($I$) through the resistance ($R$), using the following expression:
| $ \Delta\varphi = R I $ |
None
ID:(3214, 0)