Ohm's Law
Storyboard 
If a field is applied to a load, a force is obtained. Such force applied along a path leads to potential energy. If it is expressed with an electric field, the potential energy per charge that we call electrical potential is obtained. The electric potential generates displacement of charges which implies that there is a flow that we call electric current. Its magnitude depends on the electrical potential and the resistance that the material in which the electrons that we will call the conductor are. The resulting law is the so-called Ohm's law.
ID:(815, 0)
Current by a conductor
Image 
In summary, the application of a potential difference between the two ends of the
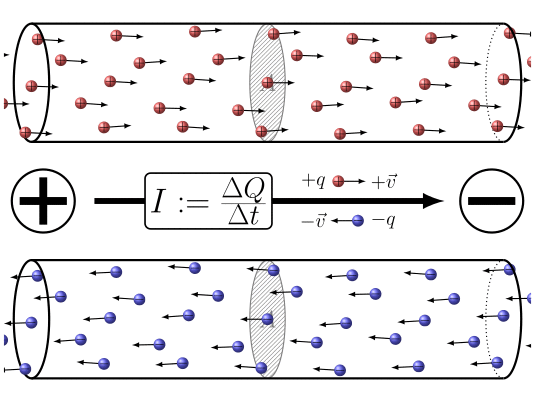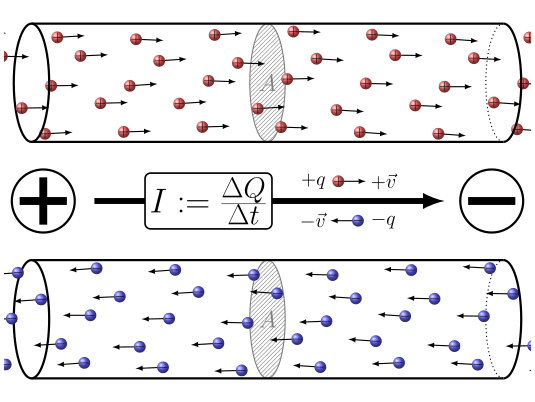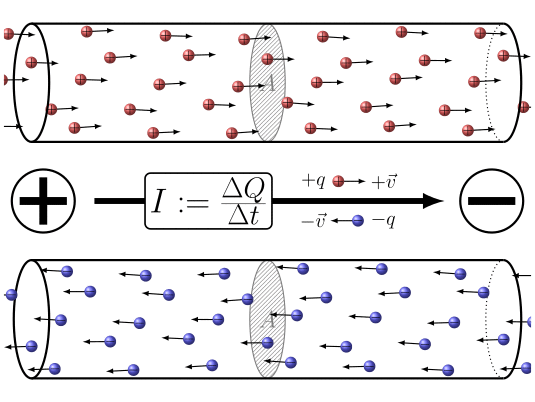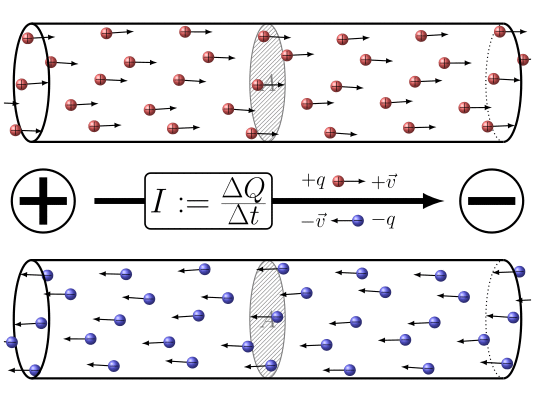
ID:(7860, 0)
Charge flow
Equation 
Si las cargas se desplazan se puede definir un flujo de cargas
| $ I =\displaystyle\frac{ dQ }{ dt }$ |
ID:(10401, 0)
Potential energy difference
Equation 
Si los extremos del conductor están a los potenciales
| $ \Delta\varphi = \varphi_2 - \varphi_1 $ |
ID:(3845, 0)
Field in the conductor
Equation 
Si la diferencia de potencial
| $ \varphi =\varphi_0 - \displaystyle\int_C \vec{E}\cdot d\vec{s}$ |
donde
| $ E =\displaystyle\frac{ \Delta\varphi }{ L }$ |
ID:(3838, 0)
Simple model for a conductor
Equation 
Si suponemos que los electrones se pueden mover en un conductor, la aplicación de un campo eléctrico
| $ F = q E $ |
Dada esta fuerza y la masa de los electrones
| $ \vec{F} = m_i \vec{a} $ |
estimar la aceleración que estos experimentan con
| $ a =\displaystyle\frac{ e E }{ m_e }$ |
ID:(3843, 0)
Resistance in the conductor
Equation 
Los átomos del conductor representan obstáculos contra los que estos impactaran. Por este motivo, la aceleración generada por un campo
| $ a =\displaystyle\frac{ e E }{ m_e }$ |
donde
Si el tiempo entre dos choques es
| $ v_{max} =\displaystyle\frac{ e E }{ m } \tau $ |
ID:(3836, 0)
Current
Equation 
If the concentration of charges is
$S,\bar{v},dt,c$
\\n\\nSi la concentración de cargas es
$S,\bar{v},dt,c$
\\n\\nSi la carga es la del electrón
$dQ,=,e,S,\bar{v},dt,c$
por lo que el flujo de cargas o corriente con charge $C$, current $A$ and time $s$
| $ I =\displaystyle\frac{ dQ }{ dt }$ |
será con charge $C$, current $A$ and time $s$
| $ I = e S c \bar{v} $ |
ID:(10400, 0)
Current per conductor (classic model)
Equation 
Si asumimos que la velocidad media es la mitad de la velocidad máxima es con ,
| $ \bar{v} =\displaystyle\frac{1}{2} v_{max} $ |
El flujo total de cargas será con average speed of charges $m/s$, charge concentration $1/m^3$, current $A$, electron Charge $C$ and section of Conductors $m^2$
| $ I = e S c \bar{v} $ |
Con la expresión para la velocidad máxima con electric eield $V/m$, electron Charge $C$, mass of the electron $kg$, maximum Speed $m/s$ and time between collisions $s$
| $ v_{max} =\displaystyle\frac{ e E }{ m } \tau $ |
se obtiene la expresión para la corriente con electric eield $V/m$, electron Charge $C$, mass of the electron $kg$, maximum Speed $m/s$ and time between collisions $s$
| $ I =\displaystyle\frac{ e ^2 E }{2 m_e } \tau c S $ |
ID:(3837, 0)
Microscopic Ohm's law
Equation 
Como la corriente en el conductor es con charge concentration $1/m^3$, current $A$, electric eield $V/m$, electron Charge $C$, mass of the electron $kg$, section of Conductors $m^2$ and time between collisions $s$
| $ I =\displaystyle\frac{ e ^2 E }{2 m_e } \tau c S $ |
El campo eléctrico en el conductor es con conductor length $m$, electric field of an infinite wire $V/m$ and potential difference $V$
| $ E =\displaystyle\frac{ \Delta\varphi }{ L }$ |
\\n\\ncon
$I=\displaystyle\frac{e^2\tau c}{2m_e}\displaystyle\frac{S}{L}\Delta\varphi$
Esta expresión se puede despejar en función del potencial tomando la forma microscópica de la ley de Ohm con conductor length $m$, electric field of an infinite wire $V/m$ and potential difference $V$:
| $ \varphi =\displaystyle\frac{2 m_e }{ e ^2 \tau c }\displaystyle\frac{ L }{ S } I $ |
ID:(3839, 0)
Specific resistivity
Equation 
La ecuación de Ohm microscópica es con charge concentration $1/m^3$, conductor length $m$, current $A$, electron Charge $C$, mass of the electron $kg$, potential difference $V$, section of Conductors $m^2$ and time between collisions $s$
| $ \varphi =\displaystyle\frac{2 m_e }{ e ^2 \tau c }\displaystyle\frac{ L }{ S } I $ |
El factor
| $ \rho_e =\displaystyle\frac{2 m_e }{ e ^2 \tau c }$ |
ID:(3840, 0)
Resistance
Equation 
La ecuación de Ohm microscópica es con charge concentration $1/m^3$, conductor length $m$, current $A$, electron Charge $C$, mass of the electron $kg$, potential difference $V$, section of Conductors $m^2$ and time between collisions $s$
| $ \varphi =\displaystyle\frac{2 m_e }{ e ^2 \tau c }\displaystyle\frac{ L }{ S } I $ |
El factor
| $ \rho_e =\displaystyle\frac{2 m_e }{ e ^2 \tau c }$ |
y es parte, con la parte geométrica
| $ R = \rho_e \displaystyle\frac{ L }{ S }$ |
ID:(3841, 0)
Ohm's law
Equation 
La ecuación de Ohm microscópica es con charge concentration $1/m^3$, conductor length $m$, current $A$, electron Charge $C$, mass of the electron $kg$, potential difference $V$, section of Conductors $m^2$ and time between collisions $s$
| $ \varphi =\displaystyle\frac{2 m_e }{ e ^2 \tau c }\displaystyle\frac{ L }{ S } I $ |
La definición de la resistividad con charge concentration $1/m^3$, electron Charge $C$, mass of the electron $kg$, resistivity $Ohm m$ and time between collisions $s$ es
| $ \rho_e =\displaystyle\frac{2 m_e }{ e ^2 \tau c }$ |
y la resistencia con conductor length $m$, resistance $Ohm$, resistivity $Ohm m$ and section of Conductors $m^2$
| $ R = \rho_e \displaystyle\frac{ L }{ S }$ |
se obtiene finalmente la ley de Ohm con conductor length $m$, resistance $Ohm$, resistivity $Ohm m$ and section of Conductors $m^2$
| $ \Delta\varphi_R = R I_R $ |
ID:(3214, 0)
Resistance and heat
Image 
The heat makes the atoms oscillate with a greater amplitude, making it difficult for the electrons to advance:
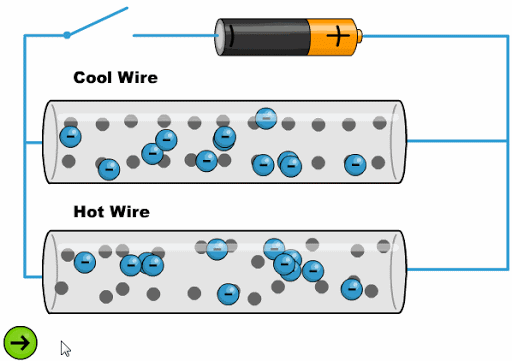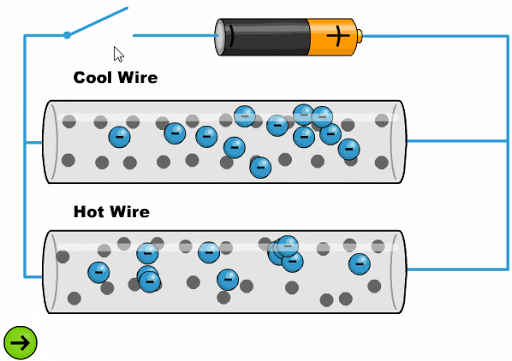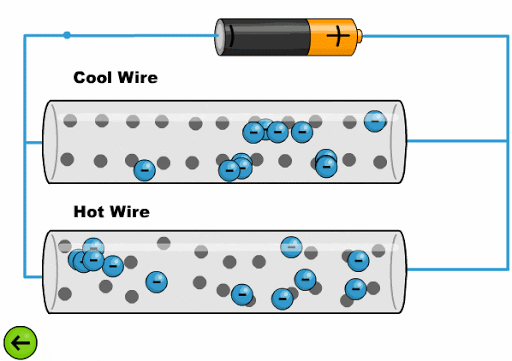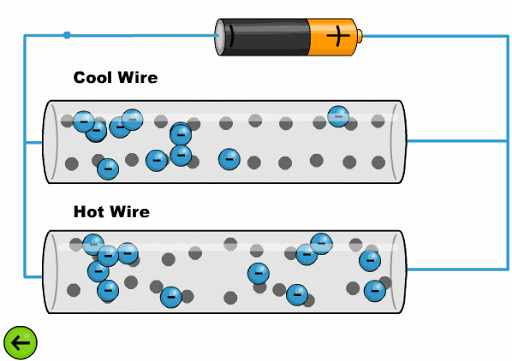
ID:(11761, 0)
Current energy
Equation 
Como el potencial eléctrico
$dW = \Delta\varphi dQ$
Si recordamos que la corriente se define con charge $C$, current $A$ and time $s$ mediante
| $ I =\displaystyle\frac{ dQ }{ dt }$ |
se tiene que la energía es
$dW = \Delta\varphi dQ = \Delta\varphi I dt$
Por ello el incremento del trabajo en el tiempo es con charge $C$, current $A$ and time $s$ igual a
| $ dW = V I dt$ |
ID:(12255, 0)
The power of an element
Equation 
Si el trabajo realizado por un elemento eléctrico es con current $A$, infinitesimal Variation of Time $s$, infinitesimal variation of work $J$ and potential difference $V$ igual a
| $ dW = V I dt$ |
y la potencia se define con como
| $ P =\displaystyle\frac{ d W }{ d t }$ |
se tiene que la potencia del elemento es con igual a
| $ P = \Delta\varphi I $ |
ID:(12258, 0)
0
Video
Video: Ohm's law
