Storyboard

Capacitance are short-distance metal plates that can be charged by connecting to a potential difference. In this way there is a plate with negative charges (electrons) and the other positive (lack of electrons so that the positive charges of the conductor structure predominate) that are held in place by the attraction between charges of different signs. Once both poles are connected by means of a conductor, current flows until it is completely discharged, the load being equalized on both plates.

>Model

ID:(816, 0)

Image

>Top

In the case of two plates with opposite charges there is a field of greater intensity between them. However, there is a minor field that can be described with field lines that emerge from one of the plates and return by giving an external turn to the opposite plate:

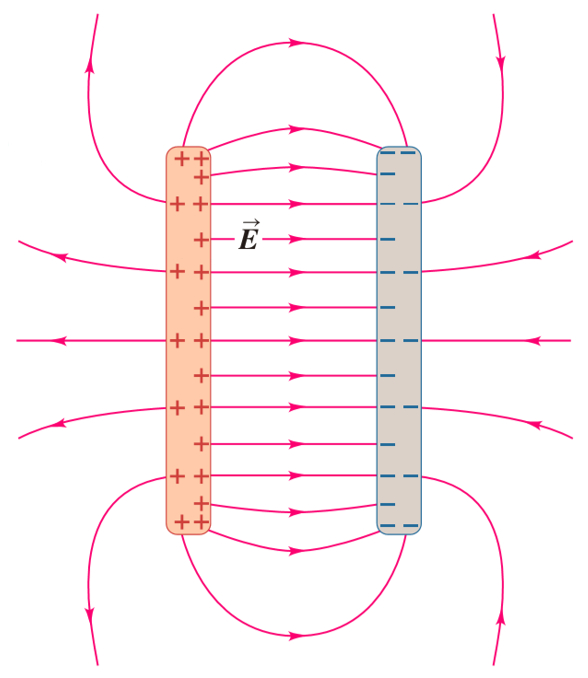
ID:(11454, 0)

Equation

>Top, >Model

Si se define una superficie que pasa entre las placas y rodea la carga Q se puede aplicar la ley de Gauss para calcular el campo que se forma entre las placas. Si se asume que el campo solo existe entre las dos placas y estas tienen una superficie S se obtiene que

$E_dS=\displaystyle\frac{Q}{\epsilon\epsilon_0}$

con \epsilon_0 la constante de campo y \epsilon el número dieléctrico.

Como por otro lado el campo es igual a la diferencia de potencial \Delta\varphi partido por la distancia entre las placas d se obtiene

$\Delta\varphi = \displaystyle\frac{\sigma}{\epsilon\epsilon_0}d=E_dd=\displaystyle\frac{Q}{\epsilon\epsilon_0}\displaystyle\frac{d}{S}$

se obtiene con la definición

$\Delta\varphi=\displaystyle\frac{Q}{C}$

que la capacidad de dos placas se puede calcular con

ID:(3865, 0)

Equation

>Top, >Model

La relación entre la carga Q en cada placa y el potencial aplicado con

ID:(3864, 0)