User:
Composition of Soils
Storyboard 
The main components of soil include both coarse and fine sand, silt, and clay. The proportion of these three components varies depending on the type of soil. Due to significant differences in particle size among these components, spaces known as pores are formed. These pores can accommodate or facilitate the flow of water, which in turn affects the moisture content of the unsaturated part of the soil.
ID:(363, 0)
Soil model
Image
Soils have a basic structure composed of different layers that result from their initial formation and how they develop, influenced by factors such as erosion and vegetation growth. While the thickness of these layers can vary significantly, they exhibit clear patterns in terms of their organic matter content:
Soil profile (commons.wikimedia.org - translated)
O: Organic Surface Layer
This layer originates from the accumulation of organic matter, primarily consisting of decomposing plant material and humus. The amount of organic material can reach up to 100%, depending on the type of vegetation and its stage of development.
A: Surface Soil
The A horizon is formed through a combination of mineral erosion, the addition of organic matter from the upper horizon, and the activities of soil organisms. Most of this layer is composed of soil's inherent mineral components, with an organic matter content that can reach up to 10%.
B: Subsoil
The B horizon accumulates minerals and leached materials that have moved downward from the A horizon. This accumulation may also result from the arrival of minerals transported from other areas and chemical processes occurring within the horizon. In this layer, the majority of components are soil minerals, with an organic matter content that can reach up to 5%.
C: Substratum
The C horizon is primarily formed by geological processes and contributes to the overall terrain configuration. It provides materials that interact with the upper layers. In this layer, most components consist of soil minerals, with an organic matter content that can reach up to 1%.
The thickness of the first two layers can vary from a few centimeters to several tens of centimeters, while the latter layers can span from tens of centimeters to meters. It is important to note that the thickness values mentioned in the Wikipedia image are provided solely as illustrative examples and may vary widely in reality.
ID:(15066, 0)
Using sieves to separate components
Concept
Soil is composed of varying proportions of sand, silt, and clay. Therefore, to model soil behavior accurately, it is necessary to represent its structure as a mixture of these components.
The simplest way to determine the composition of a particular sample is by calculating the mass of each component in relation to the total mass. To do this, physically separating each sample into its individual components is required, taking advantage of the differences in particle size. After drying and grinding the soil, a sieving process is performed using multiple sieves of different sizes, chosen based on the required precision:
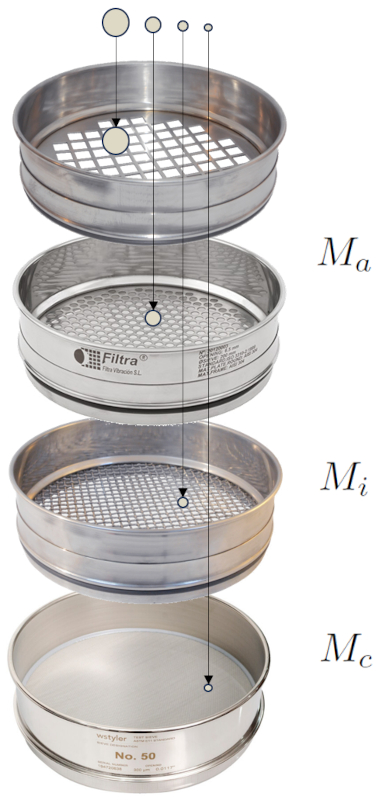
The image illustrates a scheme that separates three components, which could correspond to measurements of sand, silt, and clay. However, it is possible to use multiple sieves to differentiate, for example, coarse sand, medium sand, and fine sand, as needed.
The main masses are as follows:
• the dry mass of sand in the sample ($M_a$): Mass of all sand grains.
• the dry mass of silt in the sample ($M_i$): Mass of all silt grains.
• the dry mass of clay in the sample ($M_c$): Mass of all clay grains.
ID:(2067, 0)
Distribution of sand, silt and clay
Concept
A ternary diagram is a visual tool used to represent the relative proportions of three components, such as silt, sand, and clay. In this diagram, each corner is dedicated to one of these components, and various points within the triangular space signify unique combinations of these constituents.
To interpret a ternary diagram for silt, sand, and clay:
1. Identify the components' locations:
- Sand typically occupies the bottom-left corner.
- Silt is found at the bottom-right corner.
- Clay is situated at the top corner.
2. Analyze the mixtures:
- Points located inside the triangle denote diverse mixtures of these three components.
3. Understand lines and zones:
- Lines traversing the triangle signify consistent ratios of the three components.
- Different regions within the triangle correspond to distinct soil classifications.
In particular, for the specified zones, the following ranges can be estimated:
| Type | $g_a$ | $g_i$ | $g_c$ |
| Clay | 0-45 | 0-40 | 55-100 |
| Loam | 23-53 | 28-50 | 40-60 |
| Sand | 85-100 | 0-15 | 0-10 |
| Silt | 0-20 | 87-100 | 0-12 |
| Silty clay | 70-90 | 0-35 | 0-15 |
| Sandy clay | 45-80 | 0-28 | 20-35 |
| Clay loam | 45-65 | 0-20 | 35-55 |
| Silty clay loam | 0-50 | 50-87 | 0-27 |
| Sandy clay loam | 0-20 | 40-72 | 28-40 |
| Silt loam | 0-20 | 40-60 | 40-60 |
| Sandy loam | 20-45 | 15-53 | 28-40 |
| Loamy sand | 45-85 | 0-50 | 0-20 |
where any point has to satisfy the condition
| $ g_a + g_i + g_c = 1$ |
ID:(2070, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ g_a + g_i + g_c = 1$
g_a + g_i + g_c = 1
$ g_a =\displaystyle\frac{ M_a }{ M_s }$
g_a = M_a / M_s
$ g_c =\displaystyle\frac{ M_c }{ M_s }$
g_c = M_c / M_s
$ g_i =\displaystyle\frac{ M_i }{ M_s }$
g_i = M_i / M_s
$ M_s = M_a + M_l + M_c $
M_s = M_a + M_l + M_c
$ N_a = \displaystyle\frac{ M_a }{ m_a }$
N_a = M_a / m_a
$ N_c = \displaystyle\frac{ M_c }{ m_c }$
N_c = M_c / m_c
$ N_i = \displaystyle\frac{ M_i }{ m_i }$
N_i = M_i / m_i
$ \rho_p = \rho_a g_a + \rho_i g_i + \rho_c g_c $
rho_p = rho_a * g_a + rho_i * g_i + rho_c * g_c
$ \rho_s = \displaystyle\frac{ M_s }{ V_s }$
rho_s = M_s / V_s
$ V_a =\displaystyle\frac{ M_a }{ \rho_a }$
V_a = M_a / rho_a
$ V_a = N_a v_a $
V_a = N_a * v_a
$ V_c =\displaystyle\frac{ M_c }{ \rho_c }$
V_c = M_c / rho_c
$ V_c = N_c v_c $
V_c = N_c * v_c
$ V_i =\displaystyle\frac{ M_i }{ \rho_i }$
V_i = M_i / rho_i
$ V_i = N_i v_i $
V_i = N_i * v_i
$ V_s = V_a + V_l + V_c $
V_s = V_a + V_l + V_c
$\displaystyle\frac{1}{ \rho_s }=\displaystyle\frac{ g_a }{ \rho_a }+\displaystyle\frac{ g_i }{ \rho_i }+\displaystyle\frac{ g_c }{ \rho_c }$
1/ rho_s = g_a / rho_a + g_i / rho_i + g_c / rho_c
ID:(15216, 0)
Total dry mass of the sample
Equation
During the analysis of a sample, the first step involves removing the contained water to prevent its influence. In this way, we obtain the total Dry Mass of Sample ($M_s$), which corresponds to the sum of the dry mass of sand in the sample ($M_a$), the dry mass of silt in the sample ($M_i$), and the dry mass of clay in the sample ($M_c$):
| $ M_s = M_a + M_l + M_c $ |
It's important to emphasize that this mass must be completely dry, as moisture can distort the weight of each component. Additionally, any components such as rocks and organic material other than sand, silt, or clay must be removed from the sample.
ID:(4729, 0)
Sand fraction in the sample
Equation
To describe the soil model, we must first understand its composition. To do this, we introduce the variable representing the mass fraction of sand in the sample ($g_a$). This fraction is calculated from the dry mass of sand in the sample ($M_a$) and the total Dry Mass of Sample ($M_s$), using the following relationship:
| $ M_s = M_a + M_l + M_c $ |
This relationship is expressed as follows:
| $ g_a =\displaystyle\frac{ M_a }{ M_s }$ |
ID:(4716, 0)
Silt fraction in the sample
Equation
To describe the soil model, we must first understand its composition. To do this, we introduce the variable representing the mass fraction of silt in the sample ($g_i$). This fraction is calculated from the dry mass of silt in the sample ($M_i$) and the total Dry Mass of Sample ($M_s$), using the following relationship:
| $ M_s = M_a + M_l + M_c $ |
This relationship is expressed as follows:
| $ g_i =\displaystyle\frac{ M_i }{ M_s }$ |
ID:(15064, 0)
Clay fraction in the sample
Equation
To describe the soil model, we must first understand its composition. To do this, we introduce the variable representing the mass fraction of clay in the sample ($g_c$). This fraction is calculated from the dry mass of clay in the sample ($M_c$) and the total Dry Mass of Sample ($M_s$), using the following relationship:
| $ M_s = M_a + M_l + M_c $ |
This relationship is expressed as follows:
| $ g_c =\displaystyle\frac{ M_c }{ M_s }$ |
ID:(15065, 0)
Normalization condition
Equation
Since the mass fraction of sand in the sample ($g_a$), the mass fraction of silt in the sample ($g_i$), and the mass fraction of clay in the sample ($g_c$) are calculated based on their proportion in relation to the respective masses of the dry mass of sand in the sample ($M_a$), the dry mass of silt in the sample ($M_i$), and the dry mass of clay in the sample ($M_c$) in the sample, which sum up to the total Mass ($M_t$) for normalization:
| $ M_s = M_a + M_l + M_c $ |
We obtain a normalization condition:
| $ g_a + g_i + g_c = 1$ |
When we add the mass fraction of sand in the sample ($g_a$) in the sample:
| $ g_a =\displaystyle\frac{ M_a }{ M_s }$ |
along with the mass fraction of silt in the sample ($g_i$) in the sample:
| $ g_i =\displaystyle\frac{ M_i }{ M_s }$ |
and the mass fraction of clay in the sample ($g_c$) in the sample:
| $ g_c =\displaystyle\frac{ M_c }{ M_s }$ |
we obtain:
$g_a + g_i + g_c = \displaystyle\frac{M_a}{M_t} + \displaystyle\frac{M_i}{M_t} + \displaystyle\frac{M_c}{M_t} = \displaystyle\frac{M_a + M_i + M_c}{M_t}$
Given that $M_t$ represents the total mass, as per the condition:
| $ M_s = M_a + M_l + M_c $ |
this results in:
| $ g_a + g_i + g_c = 1$ |
ID:(15072, 0)
Number of grains of sand in the sample
Equation
To model the soil, it is important to introduce the variable corresponding to the number of sand grains in the sample ($N_a$), which can be calculated by dividing the dry mass of sand in the sample ($M_a$) by the mass of a grain of sand ($m_a$):
| $ N_a = \displaystyle\frac{ M_a }{ m_a }$ |
ID:(1539, 0)
Number of silt grains in the sample
Equation
To model the soil, it is crucial to introduce the variable corresponding to the number of silt grains in the sample ($N_i$), which can be calculated by dividing the dry mass of silt in the sample ($M_i$) by the mass of a grain of silt ($m_i$):
| $ N_i = \displaystyle\frac{ M_i }{ m_i }$ |
ID:(15067, 0)
Number of clay grains in the sample
Equation
To model the soil, it is crucial to introduce the variable corresponding to the number of clay grains in the sample ($N_c$), which can be calculated by dividing the dry mass of clay in the sample ($M_c$) by the mass of a clay plate ($m_c$):
| $ N_c = \displaystyle\frac{ M_c }{ m_c }$ |
ID:(15068, 0)
Volume of the sand component of the sample
Equation
To model the soil's behavior, it is necessary to introduce the variable corresponding to the solid volume of sand ($V_a$). This variable can be calculated from the number of sand grains in the sample ($N_a$) and the volume grain of sand ($v_a$) using the following equation:
| $ V_a = N_a v_a $ |
If we model the grain as a sphere with a radius of $r_a$, we can calculate its volume using the formula:
| $ v_a =\displaystyle\frac{4 \pi }{3} r_a ^3$ |
With the density of the sand denoted as $\rho_a$, we can determine the mass of a single sand grain using the equation:
| $ m_a = \rho_a v_a $ |
By dividing the mass of the sample's sand component $M_a$ by the mass of a single grain, we can find the number of grains:
| $ N_a = \displaystyle\frac{ M_a }{ m_a }$ |
This allows us to calculate the volume of the sand component:
| $ V_a = N_a v_a $ |
ID:(10366, 0)
Volume of the silt component of the sample
Equation
To model the soil's behavior, it is necessary to introduce the variable corresponding to the solid volume of silt ($V_i$). This variable can be calculated from the number of silt grains in the sample ($N_i$) and the volume of a grain of silt ($v_i$) using the following equation:
| $ V_i = N_i v_i $ |
If we model the grain as a sphere with a radius of $r_i$, we can calculate its volume using the formula:
| $ v_i = a_i ^3$ |
With the density of silt denoted as $\rho_i$, we can determine the mass of a single silt grain using the equation:
| $ m_i = \rho_i v_i $ |
By dividing the mass of the silt sample $M_i$ by the mass of a single grain, we can obtain the number of grains:
| $ V_c = N_c v_c $ |
This allows us to calculate the volume of the silt component:
| $ V_i = N_i v_i $ |
ID:(10365, 0)
Volume of the clay component of the sample
Equation
To model the soil's behavior, it is necessary to introduce the variable corresponding to the solid volume of clay ($V_c$). This variable can be calculated from the number of clay grains in the sample ($N_c$) and the volume of a grain of clay ($v_c$) using the following equation:
| $ V_c = N_c v_c $ |
If we model the grain as a thin plate with length and width $l_c$ and height $w_c$, we can calculate its volume using the formula:
| $ v_c = w_c l_c ^2$ |
With the density of clay denoted as $\rho_c$, we can determine the mass of a single clay grain using the equation:
| $ m_c = \rho_c v_c $ |
By dividing the mass of the clay sample $M_c$ by the mass of a single grain, we can obtain the number of grains:
| $ N_c = \displaystyle\frac{ M_c }{ m_c }$ |
This allows us to calculate the volume of the clay component:
| $ V_c = N_c v_c $ |
ID:(15069, 0)
Direct calculation of sand volume of the sample
Equation
Using the definition of density, it is possible to calculate the solid volume of sand ($V_a$) directly from the dry mass of sand in the sample ($M_a$) and the density of a grain of sand ($\rho_a$) using the following formula:
| $ V_a =\displaystyle\frac{ M_a }{ \rho_a }$ |
The direct calculation has the advantage that it does not depend on the shape of the grains:
If we assume that density is homogeneous, we can calculate the total volume independently of the number and shape of the grains.
ID:(3168, 0)
Direct calculation of silt volume of the sample
Equation
Using the definition of density, it is possible to calculate the solid volume of silt ($V_i$) directly from the dry mass of silt in the sample ($M_i$) and the density of a grain of silt ($\rho_i$) using the following formula:
| $ V_i =\displaystyle\frac{ M_i }{ \rho_i }$ |
This direct calculation has the advantage that it does not depend on the shape of the grains:
If we assume that density is homogeneous, we can calculate the total volume independently of the number and shape of the grains.
ID:(15070, 0)
Direct calculation of clay volume of the sample
Equation
Using the definition of density, it is possible to calculate the solid volume of clay ($V_c$) directly from the dry mass of clay in the sample ($M_c$) and the density of a grain of clay ($\rho_c$) using the following formula:
| $ V_c =\displaystyle\frac{ M_c }{ \rho_c }$ |
This direct calculation has the advantage that it does not depend on the shape of the grains:
If we assume that density is homogeneous, we can calculate the total volume independently of the number and shape of the grains.
ID:(15071, 0)
Solid volume of components
Equation
Once we know electron Energy ($E$), susceptible ($S_t$), and infected ($I_t$), we can determine the solid volume of a component ($V_s$) by summing the various components, as described in the following equation:
| $ V_s = V_a + V_l + V_c $ |
ID:(4734, 0)
Solid density
Equation
Since we already know the total Dry Mass of Sample ($M_s$) and the solid volume ($V_s$) from the sample, we can introduce the solid Density ($\rho_s$) and calculate it using the following equation:
| $ \rho_s = \displaystyle\frac{ M_s }{ V_s }$ |
ID:(15073, 0)
Calculation of solid density from fractions
Equation
The solid Density ($\rho_s$) can be calculated from the density of a grain of sand ($\rho_a$), the density of a grain of silt ($\rho_i$), and the density of a grain of clay ($\rho_c$), as well as from the factors describing its composition: the mass fraction of sand in the sample ($g_a$), the mass fraction of silt in the sample ($g_i$), and the mass fraction of clay in the sample ($g_c$). This leads us to the following relationship:
| $\displaystyle\frac{1}{ \rho_s }=\displaystyle\frac{ g_a }{ \rho_a }+\displaystyle\frac{ g_i }{ \rho_i }+\displaystyle\frac{ g_c }{ \rho_c }$ |
If we express the inverse of the solid Density ($\rho_s$) defined in the total Dry Mass of Sample ($M_s$) and the solid volume ($V_s$) using the equation:
| $ \rho_s = \displaystyle\frac{ M_s }{ V_s }$ |
And considering that the solid volume is the sum of the solid volume of sand ($V_a$), the solid volume of silt ($V_i$), and the solid volume of clay ($V_c$):
| $ V_s = V_a + V_l + V_c $ |
We obtain:
$\displaystyle\frac{1}{\rho_s}=\displaystyle\frac{V_s}{M_s}=\displaystyle\frac{V_a+V_i+V_c}{M_s}$
Substituting the volumes with the relationships for the density of a grain of sand ($\rho_a$)
| $ V_a =\displaystyle\frac{ M_a }{ \rho_a }$ |
for the density of a grain of silt ($\rho_i$)
| $ V_i =\displaystyle\frac{ M_i }{ \rho_i }$ |
and the density of a grain of clay ($\rho_c$)
| $ V_c =\displaystyle\frac{ M_c }{ \rho_c }$ |
We get:
$\displaystyle\frac{1}{\rho_s}=\displaystyle\frac{1}{\rho_a}\displaystyle\frac{M_a}{M_s}+\displaystyle\frac{1}{\rho_i}\displaystyle\frac{M_i}{M_s}+\displaystyle\frac{1}{\rho_c}\displaystyle\frac{M_c}{M_s}$
With the mass fraction of sand in the sample ($g_a$)
| $ g_a =\displaystyle\frac{ M_a }{ M_s }$ |
for the mass fraction of silt in the sample ($g_i$)
| $ g_i =\displaystyle\frac{ M_i }{ M_s }$ |
and the mass fraction of clay in the sample ($g_c$)
| $ g_c =\displaystyle\frac{ M_c }{ M_s }$ |
We obtain:
| $\displaystyle\frac{1}{ \rho_s }=\displaystyle\frac{ g_a }{ \rho_a }+\displaystyle\frac{ g_i }{ \rho_i }+\displaystyle\frac{ g_c }{ \rho_c }$ |
ID:(15074, 0)
Particle density
Equation
The particle density ($\rho_p$) can be calculated as the average density where the weighting factors are the components. Therefore, with the mass fraction of sand in the sample ($g_a$), the mass fraction of silt in the sample ($g_i$), the mass fraction of clay in the sample ($g_c$), and the density of a grain of sand ($\rho_a$), the density of a grain of silt ($\rho_i$), the density of a grain of clay ($\rho_c$), it can be defined as follows:
| $ \rho_p = \rho_a g_a + \rho_i g_i + \rho_c g_c $ |
ID:(15127, 0)