Druyd:Knowledge

Generalized Force

Storyboard

The generalized forces (intensive variables) and their corresponding conjugate variables (extensive variables) represent how various microscopic parameters can be calculated from microscopic distributions.

>Model

ID:(438, 0)

Generalized Force

Equation

>Top, >Model

If we expand the energy around a variable $x_i$ :

$dE = -\displaystyle\frac{\partial E}{\partial x_i}dx_i$

we recognize that the derivative of energy with respect to this variable acts as a force that tends to resist changes in the variable. For this reason, the derivative of force with respect to the variable $x_i$ , with

$X_i=-\displaystyle\frac{\partial E}{\partial x_i}$

is called the generalized force. The generalized force is an intensive variable (it does not depend on the system's size), while the associated variable is an extensive variable (it depends on the system's size).

An example of an extensive variable is volume. When we consider a larger system, its volume increases. However, pressure is intensive, meaning it does not increase when considering a larger system.

ID:(3445, 0)

Generalized force calculation

Equation

>Top, >Model

As the generalized force e with energía del sistema $J$ , fuerza generalizada $-$ and variable extensiva $-$ s

$X_i=-\displaystyle\frac{\partial E}{\partial x_i}$

can be rewritten as

$\displaystyle\frac{1}{T}=\displaystyle\frac{\partial S}{\partial E}$

using the following equation:

$X_i =\displaystyle\frac{\partial E}{\partial x_i}=\displaystyle\frac{\partial E}{\partial S}\displaystyle\frac{\partial S}{\partial x_i}=T\displaystyle\frac{\partial}{\partial x_i} (k\ln\Omega)$

which results in

$k_B T \equiv\displaystyle\frac{1}{ \beta }$

with

$X_i =\displaystyle\frac{1}{ \beta }\displaystyle\frac{\partial}{\partial x_i }\ln \Omega$

ID:(11544, 0)

Examples of intensive and extensive variables

Image

>Top

An article that summarizes most of the thermodynamic relationships very well is Use of Legendre Transforms in Chemical Thermodynamics, Robert A. Alberty, Pure Appl. Chem., Vol. 73, No. 8, pp. 13491380, 2001 containing the following table of pairs of extensive and intensive variables:

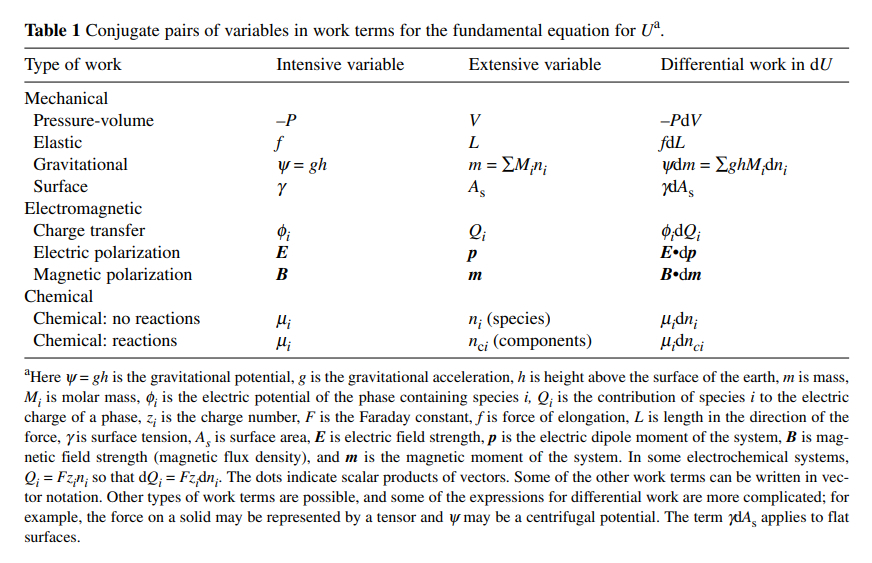
ID:(11545, 0)

Pressure as generalized force

Equation

>Top, >Model

An example of an extensive variable and generalized force is the volume $V$ with pressure $p$ . In this case, the relationship for the generalized force is given with as follows:

$\bar{p}=\displaystyle\frac{1}{\beta}\displaystyle\frac{\partial\ln\Omega}{\partial V}$

ID:(3446, 0)

General Equation of Gases

Equation

>Top, >Model

Si empleamos el numero de estados para el caso de un gas ideal tendremos que el numero de estados es con

$\Omega = \Omega_0 \left(\displaystyle\frac{2 m }{ h ^2}\right)^{3 N /2} V ^ N E ^{3N/2}$

\\n\\ncon N el numero de partículas, V el volumen, E la energía, m la masa de la partícula y h la constante de Planck.\\n\\nSi calculamos el logaritmo del numero de estados y luego diferenciamos se obtiene\\n\\n

$\displaystyle\frac{\partial \ln\Omega}{\partial V}=\displaystyle\frac{N}{V}$

por lo que se obtiene con :

$\bar{p} =\displaystyle\frac{ N }{ V } k_B T$

ID:(3447, 0)

0

Video

Video: Generalized Force