Gaussian distribution
Storyboard 
In the limit of similar probabilities the binomial distribution is reduced in the continuous limit to the Gaussean distribution.
ID:(1556, 0)
Distribución binomial
Equation 
Con la probabilidad de que se de un numero definido de pasos a la derecha e izquierda esta dada por
| W_N(n_1,n_2)=\displaystyle\frac{N!}{n_1!n_2!}p^{n_1}q^{n_2} |
con el número total de pasos es
| N=n_1+n_2 |
y solo existe la probabilidad de ir a la derecha o a la izquierda, con se tiene para las probabilidades que
| p+q=1 |
por lo que con se tiene la distribución binomial
| W_N(n) =\displaystyle\frac{ N !}{ n !( N - n )!} p ^ n (1- p )^{ N - n } |
ID:(8961, 0)
Approach for N!
Equation 
With the Stirling approximation
equation=8966
and the change of variables
equation=8996
you get that
equation
ID:(8998, 0)
Approximation for n!
Equation 
With the Stirling approximation
equation=8966
and the change of variables
equation=11431
you get that
equation
ID:(9003, 0)
Approximation for (N-n)!
Equation 
With the Stirling approximation
equation=8966
and the change of variables
equation=8997
the expression is
equation
ID:(8999, 0)
Factor N!/N!(N-n)! For N\gg 1, n\gg 1 and N>n
Equation 
In the case of medium probabilities (
| N!\sim\sqrt{2\pi N}\left(\displaystyle\frac{N}{e}\right)^N |
| n!\sim\sqrt{2\pi n}\left(\displaystyle\frac{n}{e}\right)^n |
and
| (N-n)!\sim\sqrt{2\pi(N-n)}\left(\displaystyle\frac{N-n}{e}\right)^{N-n} |
is obtained
| \displaystyle\frac{N!}{n!(N-n)!}\sim\displaystyle\frac{1}{\sqrt{2\pi N}}\left(\displaystyle\frac{n}{N}\right)^{-n-1/2}\left(\displaystyle\frac{N-n}{N}\right)^{-N+n-1/2} |
ID:(507, 0)
Limit of large Numbers and middle Probabilities
Equation 
The expression
| W_N(n) =\displaystyle\frac{ N !}{ n !( N - n )!} p ^ n (1- p )^{ N - n } |
is reduced by
| \displaystyle\frac{N!}{n!(N-n)!}\sim\displaystyle\frac{1}{\sqrt{2\pi N}}\left(\displaystyle\frac{n}{N}\right)^{-n-1/2}\left(\displaystyle\frac{N-n}{N}\right)^{-N+n-1/2} |
to representation
| W_N(n)\sim\displaystyle\frac{1}{\sqrt{2\pi N}}\left(\displaystyle\frac{n}{N}\right)^{-n-1/2}\left(\displaystyle\frac{N-n}{N}\right)^{-N+n-1/2}p^n(1-p)^{N-n} |
ID:(506, 0)
Average position
Equation 
If total
| \mu=aNp |
ID:(9008, 0)
Change variables by offset x=(n-Np)a
Equation 
To obtain the Gaussian distribution it is necessary to develop the distribution around its deviation from its mean position that can be given by
| x=(n-Np)a |
ID:(8973, 0)
Factor n/N depending on the path x
Equation 
As the way is
| x=(n-Np)a |
factor
| \displaystyle\frac{n}{N}=p\left(1+\displaystyle\frac{x}{aNp}\right) |
ID:(9004, 0)
Factor N-n/N depending on the path x
Equation 
As the way is
| x=(n-Np)a |
factor
| \displaystyle\frac{N-n}{N}=(1-p)\left(1-\displaystyle\frac{x}{aN(1-p)}\right) |
ID:(9005, 0)
Binomial distribution as a function of deviation
Equation 
If large numbers and probabilities around 1/2 are entered in the binomial distribution for the case
| W_N(n)\sim\displaystyle\frac{1}{\sqrt{2\pi N}}\left(\displaystyle\frac{n}{N}\right)^{-n-1/2}\left(\displaystyle\frac{N-n}{N}\right)^{-N+n-1/2}p^n(1-p)^{N-n} |
the expressions
| \displaystyle\frac{n}{N}=p\left(1+\displaystyle\frac{x}{aNp}\right) |
and
| \displaystyle\frac{N-n}{N}=(1-p)\left(1-\displaystyle\frac{x}{aN(1-p)}\right) |
a distribution of the form is obtained
| W_N(n)\sim\displaystyle\frac{1}{\sqrt{2\pi N}p(1-p)}\left(1+\displaystyle\frac{x}{aNp}\right)^{-n-1/2}\left(1-\displaystyle\frac{x}{aN(1-p)}\right)^{-N+n-1/2} |
ID:(8974, 0)
Variable change u=x/aNp
Equation 
To develop the
| u=\displaystyle\frac{x}{aNp} |
ID:(9021, 0)
Factor 1+x/aNp for N\gg 1 and p\sim 1/2
Equation 
With the approximation
| 1+u\sim e^{u-\frac{1}{2}u^2} |
it has to
| \left(1+\displaystyle\frac{x}{aNp}\right)\sim e^{x/aNp-x^2/2a^2N^2p^2} |
ID:(9006, 0)
Variable change u=x/aN(1-p)
Equation 
To develop the factor
| u=\displaystyle\frac{x}{aN(1-p)} |
ID:(9022, 0)
Factor 1-x /aN(1-p) for N\gg 1 and p\sim 1/2
Equation 
With the approximation
| 1+u\sim e^{u-\frac{1}{2}u^2} |
it has to
| \left(1-\displaystyle\frac{x}{aN(1-p)}\right)\sim e^{-x/aN(1-p)-x^2/2a^2N^2(1-p)^2} |
ID:(9007, 0)
Probability for large N and middle p
Equation 
It can be shown that for a large number
| P(x)=\displaystyle\frac{1}{\sqrt{2\pi N^2p(1-p)}}e^{-(x-aNp)^2/2N^2p(1-p)} |
In this case, the probability
ID:(3367, 0)
Generalization Limit Big Numbers
Equation 
$\begin{matrix}
P(x) & = & \displaystyle\frac{1}{\sqrt{2\pi\sigma^2}}e^{-(x-\mu)^2/2\sigma^2}\\
\sigma^2 & = & Np(1-p)\\
\end{matrix}
$
ID:(3368, 0)
Gaussian distribution standard deviation
Equation 
The standard deviation of the binomial distribution at the limit
| \sigma^2 = N ^2 p (1- p ) |
ID:(8963, 0)
Example comparison with Gaussian distribution
Image 
If we study the binomial distribution for large numbers
| P(x)=\displaystyle\frac{1}{\sqrt{2\pi\sigma^2}}e^{-(x-\mu)^2/2\sigma^2} |
which is represented below:
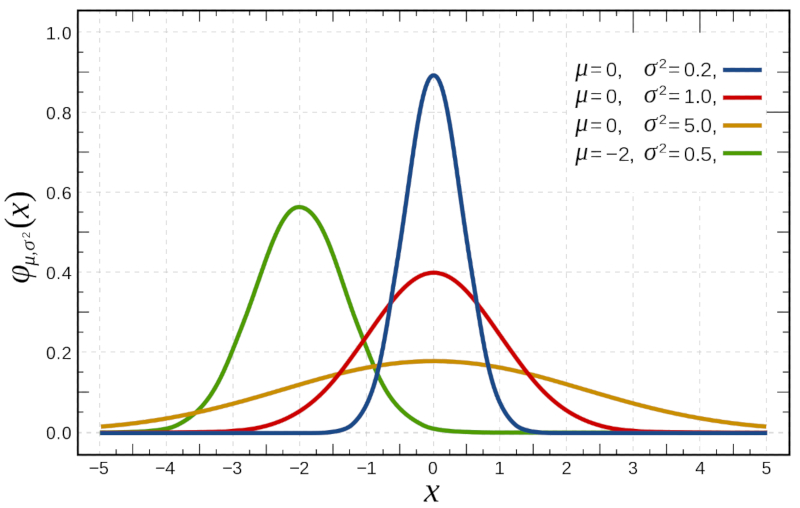
ID:(7793, 0)
0
Video
Video: Gaussian distribution
