User:


Poisson distributions
Storyboard

In the case where the probability is very small, the binomial distribution is reduced to a Poisson distribution.

>Model

ID:(1555, 0)


Distribución binomial
Equation

>Top, >Model


Con la probabilidad de que se de un numero definido de pasos a la derecha e izquierda esta dada por

$W_N(n_1,n_2)=\displaystyle\frac{N!}{n_1!n_2!}p^{n_1}q^{n_2}$



con el número total de pasos es

$N=n_1+n_2$



y solo existe la probabilidad de ir a la derecha o a la izquierda, con se tiene para las probabilidades que

$p+q=1$



por lo que con se tiene la distribución binomial

$ W_N(n) =\displaystyle\frac{ N !}{ n !( N - n )!} p ^ n (1- p )^{ N - n }$

ID:(8961, 0)


Application of the Sterling Approach
Equation

>Top, >Model


Therefore expressions such as N!/(Nn)! for N large (N\gg 1) and n small (N\gg n) can be approximated with

$u!\sim\sqrt{2\pi u}\left(\displaystyle\frac{u}{e}\right)^u$



with what you get with N\gg n

\displaystyle\frac{N!}{(N-n)!}\sim\displaystyle\frac{\sqrt{2\pi N}}{\sqrt{2\pi (N-n)}}\displaystyle\frac{N^N}{(N-n)^{N-n}}\displaystyle\frac{e^{N-n}}{e^N}\sim N^n

that is

$N^n\sim\displaystyle\frac{N!}{(N-n)!}$

ID:(4738, 0)


Desviación Estandard Poison
Equation

>Top, >Model


ID:(8964, 0)


Estimate $N! p^n/(N-n)!$ if $p\sim 0$ and $N\gg n$
Equation

>Top, >Model


With the approximation

$N^n\sim\displaystyle\frac{N!}{(N-n)!}$



and employing

$\lambda=Np$



it can be shown that

$\displaystyle\frac{N!}{(N-n)!}p^n\sim \lambda^n$

ID:(8969, 0)


Estimate of $(1-p)^{N-n}$ if $p\sim 0$ and $N\gg n$
Equation

>Top, >Model


How the exponential is defined as

$e^z\sim\left(1+\displaystyle\frac{z}{u}\right)^u$



and by entering

$\lambda=Np$



you can replace z=-\lambda=-Np and u=N-n with N\gg n what results

$e^{-\lambda}\sim (1-p)^{N-n}$

ID:(8968, 0)


Probability for large $N$ and small $p$
Equation

>Top, >Model


Since the probability of taking n steps in one direction is

$ W_N(n) =\displaystyle\frac{ N !}{ n !( N - n )!} p ^ n (1- p )^{ N - n }$



for a large number N and the probability is very small p \ll 1 can be approximated

$\displaystyle\frac{N!}{(N-n)!}p^n\sim \lambda^n$



and

$e^{-\lambda}\sim (1-p)^{N-n}$



the binomial distribution is reduced to a Poisson distribution:

$ P_{\lambda}(n) =\displaystyle\frac{ \lambda ^ n }{ n! }e^{- \lambda }$

ID:(3369, 0)


Example comparison with Poisson distribution
Image

>Top


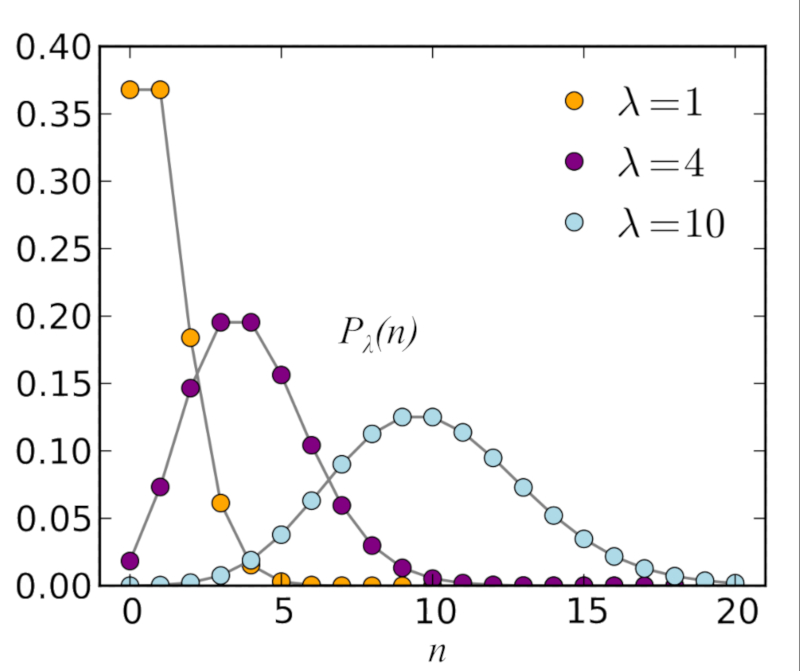
If we study the binomial distribution for large numbers N and very small probability p \ ll 1 it can be approximated using a Poisson distribution. The comparison can be done with the following simulator:

ID:(7794, 0)


0
Video

Video: Poisson distributions