User:
Electric field
Storyboard 
As loads generate forces, a load distribution will act on a load that positions one at any point in space. In other words there is a 'field' that is a force at any point in space. This force depends on the charge we expose, so it makes sense to define a force per charge so that it is independent of the particle's charge that we seek to study its behavior. Therefore it is possible to define what we call an electric field that is the total sum of all Coulomb forces of the distributed charges divided by the charge of the particle from which the behavior is being studied.
ID:(814, 0)
Definition of vector electric field
Concept
To measure Coulomb's force, a test charge must be introduced into the system. If this test charge is the test charge ($q$), the force per unit charge exerted by the system's charges on the test charge can be estimated. The magnitude of the force the force ($\vec{F}$) per unit charge the test charge ($q$) is called the electric field the electric field ($\vec{E}$) and is measured in Newtons (N) per Coulomb (C). The electric field is measured under the assumption that the test charge does not significantly disturb the system; in other words, it is assumed to be very small. The definition of the field can be written as:
| $ \vec{E} =\lim_{q\rightarrow 0}\displaystyle\frac{ \vec{F} }{ q }$ |
ID:(15784, 0)
Definition of electric field
Concept
In the case where the geometry allows for one-dimensional analysis, the force with constant mass ($F$) per the test charge ($q$) can be defined by introducing the electric eield ($E$), which is expressed as:
| $ E =\lim_{q\rightarrow 0}\displaystyle\frac{ F }{ q }$ |
ID:(15786, 0)
Electric field of a point charge
Concept
The magnitude of the force with constant mass ($F$) generated between two charges, represented by the test charge ($q$) and the charge ($Q$), which are at a distance of the distance ($r$), is calculated using the electric field constant ($\epsilon_0$) and the dielectric constant ($\epsilon$) as follows:
| $ F =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\displaystyle\frac{ q Q }{ r ^2}$ |
Using the definition of the electric field as
| $ E =\lim_{q\rightarrow 0}\displaystyle\frac{ F }{ q }$ |
we obtain
| $ E =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\displaystyle\frac{ Q }{ r ^2}$ |
ID:(790, 0)
Electric field of charge distribution
Concept
The force ($\vec{F}$) on the test charge ($q$) at the position ($\vec{r}$) will depend on the number of charges ($N$), indexed by $i$ and represented by the charge of the ion i ($Q_i$) located at the position of a charge i ($\vec{u}_i$). With the parameters the dielectric constant ($\epsilon$) and the electric field constant ($\epsilon_0$), this can be written as:
| $ \vec{F} =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\sum_i^N\displaystyle\frac{ q Q_i }{| \vec{r} - \vec{u}_i |^3}( \vec{r} - \vec{u}_i )$ |
With the definition of the electric field ($\vec{E}$) given by
| $ \vec{E} =\lim_{q\rightarrow 0}\displaystyle\frac{ \vec{F} }{ q }$ |
it follows that the electric field of a charge distribution is
| $ \vec{E} =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\sum_ i ^ N \displaystyle\frac{ Q_i }{| \vec{r} - \vec{u}_i |^3}( \vec{r} - \vec{u}_i )$ |
La ecuación se puede representar gráficamente de la siguiente manera:
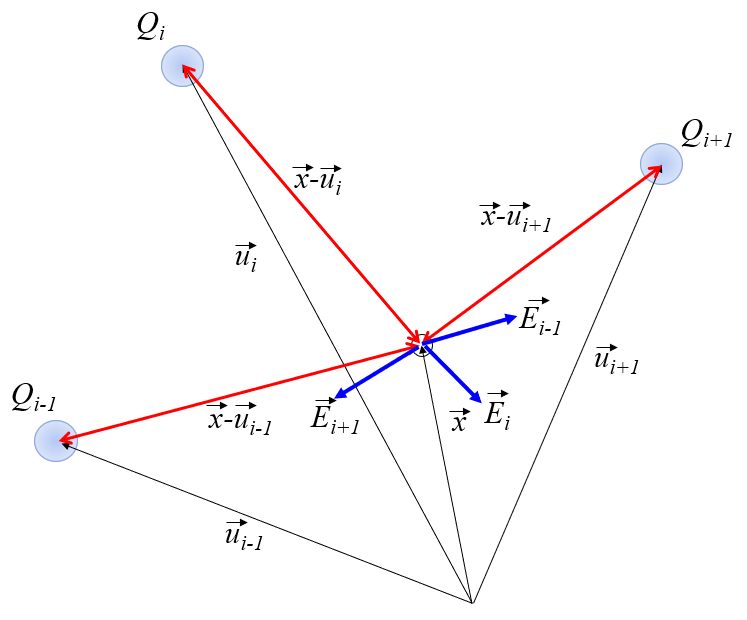
ID:(11378, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ \vec{E} =\lim_{q\rightarrow 0}\displaystyle\frac{ \vec{F} }{ q }$
&E = &F / q
$ \vec{E} =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\sum_ i ^ N \displaystyle\frac{ Q_i }{| \vec{r} - \vec{u}_i |^3}( \vec{r} - \vec{u}_i )$
&E =@SUM( Q_i / MAG( &r - &u_i )^3)( &r - &u_i )/(4* pi * epsilon_0 * epsilon ), i , 1 , N )
$ \vec{F} = q \vec{E} $
&F = q * &E
$ E =\lim_{q\rightarrow 0}\displaystyle\frac{ F }{ q }$
E = F / q
$ E =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\displaystyle\frac{ Q }{ r ^2}$
E = Q /(4* pi * epsilon * epsilon_0 * r ^2)
$ F = q E $
F = q * E
ID:(15782, 0)
Definition of vector electric field
Equation
The force ($\vec{F}$) for the test charge ($q$) is defined as the electric field ($\vec{E}$), which is expressed as:
| $ \vec{E} =\lim_{q\rightarrow 0}\displaystyle\frac{ \vec{F} }{ q }$ |
ID:(3724, 0)
Definition of electric field
Equation
The force with constant mass ($F$) for the test charge ($q$) is defined as the electric eield ($E$), which is expressed as:
| $ E =\lim_{q\rightarrow 0}\displaystyle\frac{ F }{ q }$ |
ID:(15785, 0)
Force on a charge
Equation
Once the electric eield ($E$) is known, the force with constant mass ($F$), which acts on the charge ($q$), can be calculated using:
| $ F = q E $ |
None
ID:(3872, 0)
Vector force on a charge
Equation
Once the electric field ($\vec{E}$) is known, the force ($\vec{F}$), which acts on the charge ($q$), can be calculated using:
| $ \vec{F} = q \vec{E} $ |
ID:(15811, 0)
Electric field of a point charge
Equation
The magnitude of the electric eield ($E$) generated by the charge ($Q$), which are at a distance of the distance ($r$), is calculated using the electric field constant ($\epsilon_0$) and the dielectric constant ($\epsilon$) as follows:
| $ E =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\displaystyle\frac{ Q }{ r ^2}$ |
The magnitude of the force with constant mass ($F$) generated between two charges, represented by the test charge ($q$) and the charge ($Q$), which are at a distance of the distance ($r$), is calculated using the electric field constant ($\epsilon_0$) and the dielectric constant ($\epsilon$) as follows:
| $ F =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\displaystyle\frac{ q Q }{ r ^2}$ |
Using the definition of the electric field as
| $ E =\lim_{q\rightarrow 0}\displaystyle\frac{ F }{ q }$ |
we obtain
| $ E =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\displaystyle\frac{ Q }{ r ^2}$ |
ID:(11379, 0)
Electric field distribution of charges
Equation
The electric field ($\vec{E}$) at the position ($\vec{r}$) will depend on the number of charges ($N$), accounted for with the index $i$ represented by the charge of the ion i ($Q_i$) located at the position of a charge i ($\vec{u}_i$). With the parameters the dielectric constant ($\epsilon$) and the electric field constant ($\epsilon_0$), this can be written as:
| $ \vec{E} =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\sum_ i ^ N \displaystyle\frac{ Q_i }{| \vec{r} - \vec{u}_i |^3}( \vec{r} - \vec{u}_i )$ |
The force ($\vec{F}$) on the test charge ($q$) at the position ($\vec{r}$) will depend on the number of charges ($N$), indexed by $i$ and represented by the charge of the ion i ($Q_i$) located at the position of a charge i ($\vec{u}_i$). With the parameters the dielectric constant ($\epsilon$) and the electric field constant ($\epsilon_0$), this can be written as:
| $ \vec{F} =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\sum_i^N\displaystyle\frac{ q Q_i }{| \vec{r} - \vec{u}_i |^3}( \vec{r} - \vec{u}_i )$ |
With the definition of the electric field ($\vec{E}$) given by
| $ \vec{E} =\lim_{q\rightarrow 0}\displaystyle\frac{ \vec{F} }{ q }$ |
it follows that the electric field of a charge distribution is
| $ \vec{E} =\displaystyle\frac{1}{4 \pi \epsilon_0 \epsilon }\sum_ i ^ N \displaystyle\frac{ Q_i }{| \vec{r} - \vec{u}_i |^3}( \vec{r} - \vec{u}_i )$ |
ID:(3726, 0)