Thermodynamic potentials
Description 
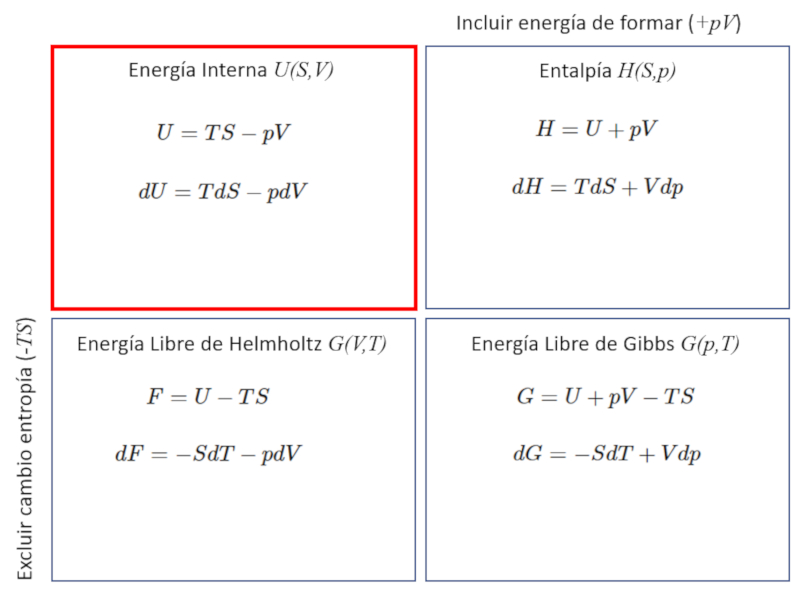
The thermodynamic potentials correspond to different variations of the internal energy $U$, which can include the energy required to form the system equivalent to the work $pV$, as well as the energy that cannot be used to perform work, which is $TS$.
ID:(12348, 0)
Internal energy
Concept 
If the absolute temperature ($T$) and the pressure ($p$) are kept constant, the variation of the internal energy ($dU$), which depends on the entropy variation ($dS$) and the volume Variation ($dV$), is expressed as:
| $ dU = T dS - p dV $ |
Integrating this results in the following expression in terms of the internal energy ($U$), the entropy ($S$), and the volume ($V$):
| $ U = T S - p V $ |
![]() [1] "Über die quantitative und qualitative Bestimmung der Kräfte" (On the Quantitative and Qualitative Determination of Forces), Julius Robert von Mayer, Annalen der Chemie und Pharmacie, 1842
[1] "Über die quantitative und qualitative Bestimmung der Kräfte" (On the Quantitative and Qualitative Determination of Forces), Julius Robert von Mayer, Annalen der Chemie und Pharmacie, 1842
![]() [2] "Über die Erhaltung der Kraft" (On the Conservation of Force), Hermann von Helmholtz, 1847
[2] "Über die Erhaltung der Kraft" (On the Conservation of Force), Hermann von Helmholtz, 1847
ID:(214, 0)
Internal Energy: differential ratio
Equation 
The dependency of the internal energy differential ($dU$) on the pressure ($p$) and the volume Variation ($dV$), in addition to the absolute temperature ($T$) and the entropy variation ($dS$), is given by:
As the internal energy differential ($dU$) depends on the differential inexact Heat ($\delta Q$), the pressure ($p$), and the volume Variation ($dV$) according to the equation:
| $ dU = \delta Q - p dV $ |
and the expression for the second law of thermodynamics with the absolute temperature ($T$) and the entropy variation ($dS$) as:
| $ \delta Q = T dS $ |
we can conclude that:
| $ dU = T dS - p dV $ |
.
ID:(3471, 0)
Internal Energy
Equation 
The internal energy ($U$) is with the absolute temperature ($T$), the pressure ($p$), the entropy ($S$) and the volume ($V$) equal to:
If the absolute temperature ($T$) and the pressure ($p$) are kept constant, the variation of the internal energy ($dU$), which depends on the entropy variation ($dS$) and the volume Variation ($dV$), is expressed as:
| $ dU = T dS - p dV $ |
Integrating this results in the following expression in terms of the internal energy ($U$), the entropy ($S$), and the volume ($V$):
| $ U = T S - p V $ |
ID:(3472, 0)
Enthalpy
Concept 
The enthalpy ($H$) refers to the energy contained within a system, including any energy required to create it. It is composed of the internal energy ($U$) and the work necessary to form the system, which is represented as $pV$ where the pressure ($p$) and the volume ($V$) are involved.
It is a function of the entropy ($S$) and the pressure ($p$), allowing it to be expressed as $H = H(S,p)$ and it follows the following mathematical relation:
| $ H = U + p V $ |
An article that can be considered as the origin of the concept, although it does not include the definition of the name, is:
![]() [1] "Memoir on the Motive Power of Heat, Especially as Regards Steam, and on the Mechanical Equivalent of Heat," written by Benoît Paul Émile Clapeyron (1834).
[1] "Memoir on the Motive Power of Heat, Especially as Regards Steam, and on the Mechanical Equivalent of Heat," written by Benoît Paul Émile Clapeyron (1834).
ID:(215, 0)
Enthalpy
Equation 
The enthalpy ($H$) is defined as the sum of the internal energy ($U$) and the formation energy. The latter corresponds to the work done in the formation, which is equal to $pV$ with the pressure ($p$) and the volume ($V$). Therefore, we have:
ID:(3536, 0)
Differential Enthalpy Relationship
Equation 
The dependency of the differential enthalpy ($dH$) on the absolute temperature ($T$) and the entropy variation ($dS$), in addition to the volume ($V$) and the pressure Variation ($dp$), is given by:
If we differentiate the definition of the enthalpy ($H$), which depends on the internal energy ($U$), the pressure ($p$), and the volume ($V$), given by:
| $ H = U + p V $ |
we obtain:
$dH = dU + Vdp + pdV$
using the differential enthalpy ($dH$), the internal energy differential ($dU$), the pressure Variation ($dp$), and the volume Variation ($dV$).
By differentiating the internal energy ($U$) with respect to the absolute temperature ($T$) and the entropy ($S$),
| $ U = T S - p V $ |
we get:
| $ dU = T dS - p dV $ |
with the internal energy differential ($dU$) and the entropy variation ($dS$).
Therefore, it finally results in:
| $ dH = T dS + V dp $ |
ID:(3473, 0)
Enthalpy Ratio
Equation 
The enthalpy ($H$) is reduced with the absolute temperature ($T$) and the entropy ($S$) to:
The enthalpy ($H$) is defined using the internal energy ($U$), the pressure ($p$), and the volume ($V$) as:
| $ H = U + p V $ |
Considering the internal energy ($U$) as a function of the entropy ($S$) and the speed of the Oval Window ($v_2$) as:
| $ U = T S - p V $ |
it simplifies to:
| $ H = T S $ |
ID:(3476, 0)
Helmholtz free energy
Concept 
The helmholtz free fnergy ($F$) [1] refers to the energy contained within a system, but excludes the energy that cannot be used to perform work. In this sense, it represents the energy available to do work as long as it does not include the energy required to form the system. It is composed, therefore, of the internal energy ($U$), from which the thermal energy $ST$, where the entropy ($S$) and the absolute temperature ($T$) are involved, is subtracted.
This function depends on the absolute temperature ($T$) and the volume ($V$), allowing it to be expressed as $F = F(V,T)$, and it satisfies the following mathematical relation:
| $ F = U - T S $ |
![]() [1] "Über die Thermodynamik chemischer Vorgänge" (On the thermodynamics of chemical processes.), Hermann von Helmholtz, Dritter Beitrag. Offprint from: ibid., 31 May, (1883)
[1] "Über die Thermodynamik chemischer Vorgänge" (On the thermodynamics of chemical processes.), Hermann von Helmholtz, Dritter Beitrag. Offprint from: ibid., 31 May, (1883)
ID:(216, 0)
Helmholtz free Energy
Equation 
The helmholtz free fnergy ($F$) is defined as the difference between the internal energy ($U$) and the energy that cannot be utilized to perform work. The latter corresponds to $ST$ with the entropy ($S$) and the absolute temperature ($T$). Therefore, we obtain:
ID:(14047, 0)
Differential relation Helmholtz Free Energy
Equation 
The dependency of the differential Helmholtz Free Energy ($dF$) on the entropy ($S$) and the temperature variation ($dT$), in addition to the pressure ($p$) and the volume Variation ($dV$), is given by:
The helmholtz free fnergy ($F$) is defined using the internal energy ($U$), the absolute temperature ($T$), and the entropy ($S$) as:
| $ F = U - T S $ |
When we differentiate this equation, we obtain with the differential Helmholtz Free Energy ($dF$), the variation of the internal energy ($dU$), the entropy variation ($dS$), and the temperature variation ($dT$):
$dF = dU - TdS - SdT$
With the differential of internal energy and the variables the pressure ($p$) and the volume Variation ($dV$),
| $ dU = T dS - p dV $ |
we finally obtain:
| $ dF =- S dT - p dV $ |
ID:(3474, 0)
Helmholtz Free Energy Relation
Equation 
The helmholtz free fnergy ($F$) is reduced with the pressure ($p$) and the volume ($V$) to:
Expressing the helmholtz free fnergy ($F$) in terms of the internal energy ($U$), the absolute temperature ($T$), and the entropy ($S$), we have the following equation:
| $ F = U - T S $ |
By substituting the internal energy ($U$), which is a function of the pressure ($p$) and the volume ($V$), we obtain:
| $ U = T S - p V $ |
This leads to the following expression:
| $ F = - p V $ |
ID:(3477, 0)
Gibbs free energy
Concept 
The gibbs free energy ($G$) refers to the energy contained within a system, including the energy required for its formation, but excludes the energy that cannot be used to do work. In this sense, it represents the energy available to do work in a process that includes the energy required for its formation. It is composed, therefore, of the enthalpy ($H$), from which the thermal energy $ST$, where the entropy ($S$) and the absolute temperature ($T$) are involved, is subtracted.
This function depends on the absolute temperature ($T$) and the pressure ($p$), allowing it to be expressed as $G = G(T,p)$ and it satisfies the following mathematical relation:
| $ G = H - T S $ |
![]() [1] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 108-248 (October 1875 May 1876)
[1] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 108-248 (October 1875 May 1876)
![]() [2] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 343-524 (May 1877 July 1878)
[2] "On the Equilibrium of Heterogeneous Substances," J. Willard Gibbs, Transactions of the Connecticut Academy of Arts and Sciences, 3: 343-524 (May 1877 July 1878)
ID:(217, 0)
Gibbs and Helmholtz free energy
Equation 
The gibbs free energy ($G$) [1,2] represents the total energy, encompassing both the internal energy and the formation energy of the system. It is defined as the enthalpy ($H$), excluding the portion that cannot be used to perform work, which is represented by $TS$ with the absolute temperature ($T$) and the entropy ($S$). This relationship is expressed as follows:
ID:(3542, 0)
Gibbs free energy as differential
Equation 
The dependency of the variation of Gibbs Free Energy ($dG$) on the entropy ($S$) and the temperature variation ($dT$), in addition to the volume ($V$) and the pressure Variation ($dp$), is given by:
The gibbs free energy ($G$) as a function of the enthalpy ($H$), the entropy ($S$), and the absolute temperature ($T$) is expressed as:
| $ G = H - T S $ |
The value of the differential of the Gibbs free energy ($dG$) is determined using the differential enthalpy ($dH$), the temperature variation ($dT$), and the entropy variation ($dS$) through the equation:
$dG=dH-SdT-TdS$
Since the differential enthalpy ($dH$) is related to the volume ($V$) and the pressure Variation ($dp$) as follows:
| $ dH = T dS + V dp $ |
It follows that the differential enthalpy ($dH$), the entropy variation ($dS$), and the pressure Variation ($dp$) are interconnected in the following manner:
| $ dG =- S dT + V dp $ |
ID:(3541, 0)
Gibbs Free Energy Ratio
Equation 
The gibbs free energy ($G$) reduces to:
The gibbs free energy ($G$) with the internal energy ($U$), the entropy ($S$), the absolute temperature ($T$), the pressure ($p$), and the volume ($V$) is represented as:
| $ G = U - S T + p V $ |
And with the substitution of the internal energy ($U$),
| $ U = T S - p V $ |
We obtain:
| $ G = 0$ |
ID:(3478, 0)
