User:
Solutions
Storyboard 
When a material (solute) is dissolved in a liquid (solvent), the physical properties of the solvent change.
In the presence of a semipermeable membrane that allows the solvent to pass through but retains the solute, osmotic pressure is generated. This phenomenon results in a reduction of the effective pressure in the solvent.
Additionally, the dissolution affects the liquid's phase transition temperatures. Specifically, it lowers the freezing point and raises the boiling point, altering its thermal behavior.
ID:(1675, 0)
Solutions Phase Diagram
Image
In a phase diagram of a solution, the boundaries between phases shift in such a way that, at the same pressure, the melting point decreases while the boiling point increases:
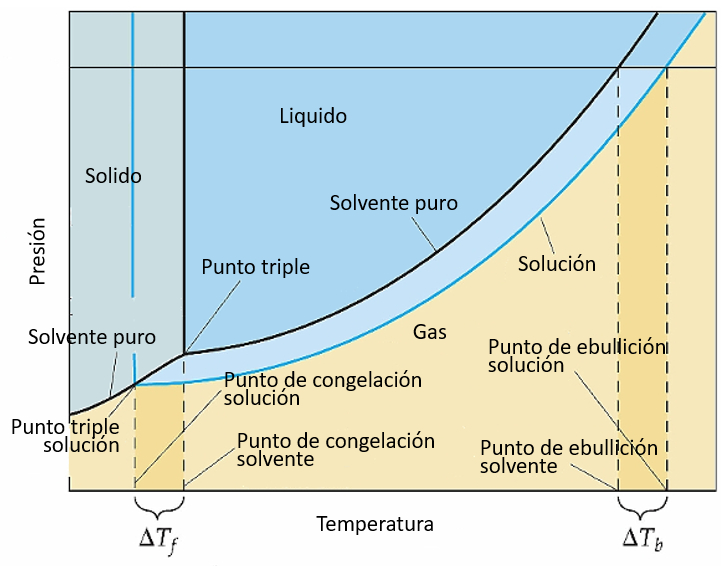
ID:(1980, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ l_S = T_f ( s_L - s_S )$
l_S = T_f *( s_L - s_S )
$ l_V = T_b ( s_V - s_L )$
l_V = T_b *( s_V - s_L )
$ \mu_L N_A = s_L ( T_{fs} - T_f ) - \displaystyle\frac{ N_s }{ N } R T_{fs} $
mu_L * N_A = s_L *( T - T_L ) - N_s * R * T / N
$ \mu_L N_A = s_L ( T_{bs} - T_b ) - \displaystyle\frac{ N_s }{ N } R T_{bs} $
mu_L * N_A = s_L *( T - T_L ) - N_s * R * T / N
$ \mu_S N_A = s_S ( T_{fs} - T_f )$
mu_S * N_A = s_S *( T - T_S )
$ \mu_V N_A = s_V ( T_{bs} - T_b )$
mu_V * N_A = s_V *( T - T_V )
$ n = \displaystyle\frac{ M }{ M_m }$
n = M / M_m
$ n_s = \displaystyle\frac{ M_s }{ M_{ms} }$
n = M / M_m
$ n \equiv\displaystyle\frac{ N }{ N_A }$
n = N / N_A
$ n_s \equiv\displaystyle\frac{ N_s }{ N_A }$
n = N / N_A
$ T_{bs} = T_b +\displaystyle\frac{ N_s }{ N }\displaystyle\frac{ R T_b ^2}{ l_V }$
T_bs = T_b +N_s * R * T_b ^2/( N * l_V )
$ T_{fs} = T_f - \displaystyle\frac{ N_s }{ N }\displaystyle\frac{ R T_f ^2}{ l_S }$
T_fs = T_f - R * N_s * T_f ^2/( l_S * N )
ID:(15348, 0)
The chemical potential for a gas
Equation
The chemical vapor potential ($\mu_V$), together with the avogadro's number ($N_A$), is equal to the molar entropy of vapor ($s_V$) multiplied by the difference between the absolute temperature ($T$) and the steam reference temperature ($T_V$), expressed as:
| $ \mu_V N_A = s_V ( T_{bs} - T_b )$ |
| $ \mu_V N_A = s_V ( T - T_V )$ |
ID:(12815, 0)
The chemical potential for a solid
Equation
The chemical potential of the solid ($\mu_S$), together with the avogadro's number ($N_A$), is equivalent to the molar entropy of the solid ($s_S$) multiplied by the difference between the absolute temperature ($T$) and the solid reference temperature ($T_S$), represented as:
| $ \mu_S N_A = s_S ( T_{fs} - T_f )$ |
| $ \mu_S N_A = s_S ( T - T_S )$ |
ID:(12816, 0)
The chemical potential for a solution (1)
Equation
The chemical potential of the liquid ($\mu_L$), together with the avogadro's number ($N_A$), is equal to the molar entropy of the liquid ($s_L$) multiplied by the difference between the absolute temperature ($T$) and the liquid reference temperature ($T_L$), in addition to the effect of osmotic pressure, which depends on the number of ions ($N_s$), the number of particles ($N$), the absolute temperature ($T$), and the universal gas constant ($R$), represented as:
| $ \mu_L N_A = s_L ( T_{fs} - T_f ) - \displaystyle\frac{ N_s }{ N } R T_{fs} $ |
| $ \mu_L N_A = s_L ( T - T_L ) - \displaystyle\frac{ N_s }{ N } R T $ |
ID:(12817, 1)
The chemical potential for a solution (2)
Equation
The chemical potential of the liquid ($\mu_L$), together with the avogadro's number ($N_A$), is equal to the molar entropy of the liquid ($s_L$) multiplied by the difference between the absolute temperature ($T$) and the liquid reference temperature ($T_L$), in addition to the effect of osmotic pressure, which depends on the number of ions ($N_s$), the number of particles ($N$), the absolute temperature ($T$), and the universal gas constant ($R$), represented as:
| $ \mu_L N_A = s_L ( T_{bs} - T_b ) - \displaystyle\frac{ N_s }{ N } R T_{bs} $ |
| $ \mu_L N_A = s_L ( T - T_L ) - \displaystyle\frac{ N_s }{ N } R T $ |
ID:(12817, 2)
Latent heat of freezing
Equation
If the temperatura de fusión ($T_f$) represents the boiling temperature, the molar entropy of the liquid ($s_L$) corresponds to the molar entropy of the liquid, and the molar entropy of the solid ($s_S$) to that of the solid, then the enthalpy of evaporation the calor latente molar del cambio de fase solido liquido ($l_S$) is calculated using the following formula:
| $ l_S = T_f ( s_L - s_S )$ |
ID:(9051, 0)
Calor latente de ebullición
Equation
If the boiling temperature ($T_b$) is the boiling temperature, the molar entropy of vapor ($s_V$) represents the molar entropy of the vapor, and the molar entropy of the liquid ($s_L$) represents that of the liquid, then the enthalpy of evaporation the calor latente molar del cambio de fase liquido vapor ($l_V$) is calculated using the following expression:
| $ l_V = T_b ( s_V - s_L )$ |
ID:(9050, 0)
Boiling Point Elevation by Solution
Equation
Another effect that varies in solutions is the boiling point. When a solvent boils at a temperature $T$ and pressure $p$ in its pure state, the chemical potential of the liquid phase must equal the chemical potential of the vapor phase.
However, due to the reduction in vapor pressure caused by the presence of a solute, an increase in temperature is required to achieve this equilibrium. As a result, the boiling point of a solution is higher than that of the pure solvent.
Therefore, the boiling temperature with solute ($T_{bs}$), together with the boiling temperature ($T_b$), the number of ions ($N_s$), the number of particles ($N$), the calor latente molar del cambio de fase liquido vapor ($l_V$), and the universal gas constant ($R$), is equal to:
| $ T_{bs} = T_b +\displaystyle\frac{ N_s }{ N }\displaystyle\frac{ R T_b ^2}{ l_V }$ |
Si se considera como temperatura de referencia
| $ \mu_L N_A = s_L ( T - T_L ) - \displaystyle\frac{ N_s }{ N } R T $ |
y el del vapor
| $ \mu_V N_A = s_V ( T_{bs} - T_b )$ |
se tiene que la temperatura de ebullición de la solución
$s_L (T_s-T_b)-\displaystyle\frac{N_s}{N}RT_s= s_V (T_s-T_b)$
Con
| $ l_V = T_b ( s_V - s_L )$ |
se tiene que con
| $ T_{bs} = T_b +\displaystyle\frac{ N_s }{ N }\displaystyle\frac{ R T_b ^2}{ l_V }$ |
ID:(12819, 0)
Freezing Point Reduction by Solution
Equation
Another effect that varies in solutions is the freezing point. When a solvent freezes at a temperature $T$ and pressure $p$ in its pure state, the chemical potential of the solid phase must equal that of the liquid phase.
Due to the reduction in vapor pressure caused by the presence of a solute, the temperature must be lowered to achieve this equilibrium. As a result, the freezing point of the solvent decreases in a solution.
Thus, the freezing temperature with solute ($T_{fs}$), together with the freezing temperature ($T_f$), the number of ions ($N_s$), the number of particles ($N$), the calor latente molar del cambio de fase solido liquido ($l_S$), and the universal gas constant ($R$), is equal to:
| $ T_{fs} = T_f - \displaystyle\frac{ N_s }{ N }\displaystyle\frac{ R T_f ^2}{ l_S }$ |
Si se considera como temperatura de referencia
| $ \mu_L N_A = s_L ( T - T_L ) - \displaystyle\frac{ N_s }{ N } R T $ |
y el del solido
| $ \mu_S N_A = s_S ( T_{fs} - T_f )$ |
se tiene que la temperatura de ebullición de la solución
$s_S (T_s-T_b)= s_L (T_s-T_b)-\displaystyle\frac{N_s}{N}RT_s$
Con
| $ l_S = T_f ( s_L - s_S )$ |
se tiene que con
| $ T_{fs} = T_f - \displaystyle\frac{ N_s }{ N }\displaystyle\frac{ R T_f ^2}{ l_S }$ |
ID:(12818, 0)
Number of moles (1)
Equation
The number of moles ($n$) corresponds to the number of particles ($N$) divided by the avogadro's number ($N_A$):
| $ n \equiv\displaystyle\frac{ N }{ N_A }$ |
ID:(3748, 1)
Number of moles with molar mass (1)
Equation
The number of moles ($n$) is determined by dividing the mass ($M$) of a substance by its the molar Mass ($M_m$), which corresponds to the weight of one mole of the substance.
Therefore, the following relationship can be established:
| $ n = \displaystyle\frac{ M }{ M_m }$ |
The number of moles ($n$) corresponds to the number of particles ($N$) divided by the avogadro's number ($N_A$):
| $ n \equiv\displaystyle\frac{ N }{ N_A }$ |
If we multiply both the numerator and the denominator by the particle mass ($m$), we obtain:
$n=\displaystyle\frac{N}{N_A}=\displaystyle\frac{Nm}{N_Am}=\displaystyle\frac{M}{M_m}$
So it is:
| $ n = \displaystyle\frac{ M }{ M_m }$ |
The molar mass is expressed in grams per mole (g/mol).
ID:(4854, 1)
Number of moles (2)
Equation
The number of moles ($n$) corresponds to the number of particles ($N$) divided by the avogadro's number ($N_A$):
| $ n_s \equiv\displaystyle\frac{ N_s }{ N_A }$ |
| $ n \equiv\displaystyle\frac{ N }{ N_A }$ |
ID:(3748, 2)
Number of moles with molar mass (2)
Equation
The number of moles ($n$) is determined by dividing the mass ($M$) of a substance by its the molar Mass ($M_m$), which corresponds to the weight of one mole of the substance.
Therefore, the following relationship can be established:
| $ n_s = \displaystyle\frac{ M_s }{ M_{ms} }$ |
| $ n = \displaystyle\frac{ M }{ M_m }$ |
The number of moles ($n$) corresponds to the number of particles ($N$) divided by the avogadro's number ($N_A$):
| $ n \equiv\displaystyle\frac{ N }{ N_A }$ |
If we multiply both the numerator and the denominator by the particle mass ($m$), we obtain:
$n=\displaystyle\frac{N}{N_A}=\displaystyle\frac{Nm}{N_Am}=\displaystyle\frac{M}{M_m}$
So it is:
| $ n = \displaystyle\frac{ M }{ M_m }$ |
The molar mass is expressed in grams per mole (g/mol).
ID:(4854, 2)