Benützer:
Flacher Spiegel
Storyboard 
Bei einem flachen Spiegel wird das Licht in einem Winkel reflektiert, der mit dem Winkel identisch ist, den es beeinflusst.
Im Allgemeinen verfügt das Licht nicht über die Informationen, die mit der Annahme reflektiert wurden, dass das Licht 'hinter' dem Spiegel entsteht.
ID:(1263, 0)
La ley de reflexión
Bild
Aplicando el principio de Huygens se muestra que un haz que incide sobre una superficie se refleja bajo un angulo igual al de incidencia:
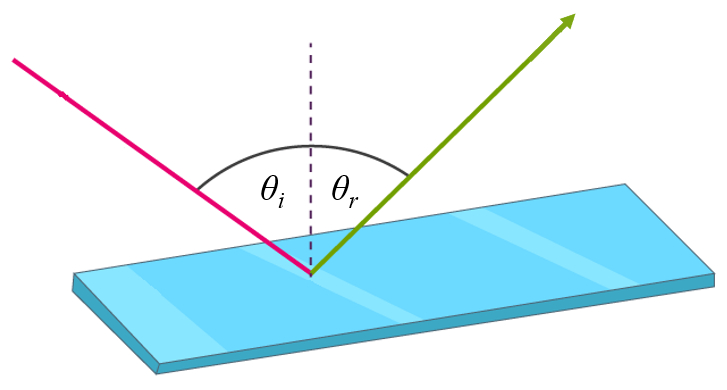
ID:(12758, 0)
Ein Spiegel
Bild
Ein Spiegel sieht aus wie ein Fenster zu einem anderen Raum. Der Effekt wird durch das reflektierte Licht erzeugt, von dem das Auge annimmt, dass es nicht reflektiert wurde, sondern von einem Objekt hinter dem Spiegel kommt.
ID:(9777, 0)
Relación entre ángulos de incidencia y reflexión
Bild
Del análisis mediante el principio de Huygens se concluye que los ángulos de incidencia y reflexión son iguales:
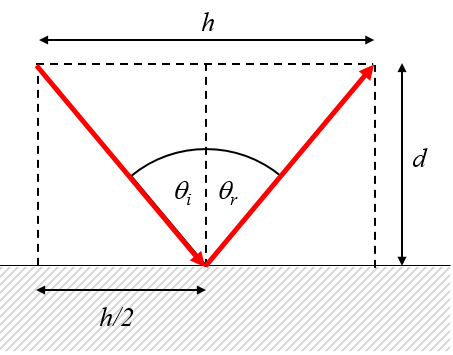
ID:(12665, 0)
Relación entre ángulos de incidencia y reflexión
Bild
En caso de dos espejos con una esquina
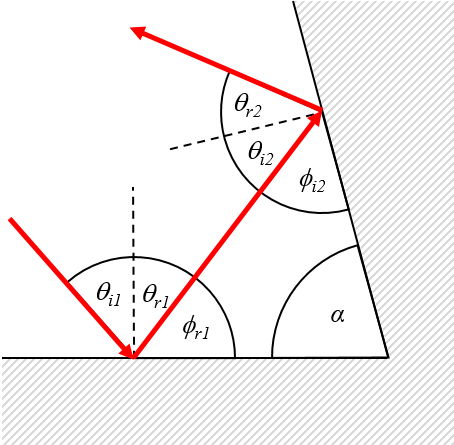
se pueden calcular los ángulos con la relación de reflexión
| $ \theta_i = \theta_r $ |
el calculo del complemento del angulo incidente
| $ \theta_{rc} =\displaystyle\frac{ \pi }{2} - \theta_r $ |
el calculo del complemento del angulo de reflección
| $ \theta_{ic} =\displaystyle\frac{ \pi }{2} - \theta_i $ |
y la relación entre los ángulos de un triangulo
| $ \pi = \alpha + \beta + \gamma $ |
ID:(12666, 0)
Modell
Top
Parameter
Variablen
Berechnungen
zu 
, dann die Variable auswählen: 
zu 
Berechnungen
Berechnungen
Variable 
Gegeben Berechnen 
Ziel : Gleichung Zu verwenden 
Gleichungen
$ \pi = \alpha + \beta + \gamma $
pi = alpha + beta + gamma
$ \tan \theta_i =\displaystyle\frac{ h }{2 d }$
theta_i = tan( h /(2* d ))
$ \theta_i = \theta_r $
theta_i = theta_r
$ \theta_{ic} =\displaystyle\frac{ \pi }{2} - \theta_i $
theta_ic = pi /2- theta_i
$ \theta_{rc} =\displaystyle\frac{ \pi }{2} - \theta_r $
theta_rc = pi /2- theta_r
ID:(16061, 0)
Richtung des reflektierten Licht
Gleichung
Para la luz reflejada el angulo del haz respecto de la normal
| $ \theta_i = \theta_r $ |
ID:(3262, 0)
Komplementärer Einfallswinkel
Gleichung
Para el calculo de los ángulos en el caso de que los haces se reflejan en un espejo es útil poder calcular el angulo complementario al de incidencia. Por ello se tiene que
| $ \theta_{ic} =\displaystyle\frac{ \pi }{2} - \theta_i $ |
Como la suma de los ángulos internos en un triangulo es
| $ \pi = \alpha + \beta + \gamma $ |
se tiene que en un rectángulo, en el que uno de los ángulos es
| $ \theta_{ic} =\displaystyle\frac{ \pi }{2} - \theta_i $ |
ID:(10928, 0)
Angulo complementario del angulo de reflexión
Gleichung
Para el calculo de los ángulos en el caso de que los haces se reflejan en un espejo es útil poder calcular el angulo complementario al de reflexión. Por ello se tiene que
| $ \theta_{rc} =\displaystyle\frac{ \pi }{2} - \theta_r $ |
Como la suma de los ángulos internos en un triangulo es
| $ \pi = \alpha + \beta + \gamma $ |
se tiene que en un rectángulo, en el que uno de los ángulos es
| $ \theta_{rc} =\displaystyle\frac{ \pi }{2} - \theta_r $ |
ID:(10925, 0)
Winkelsumme eines Dreiecks
Gleichung
| $ \pi = \alpha + \beta + \gamma $ |
ID:(10926, 0)
Angulo de incidencia
Gleichung
El angulo de incidencia
| $ \tan \theta_i =\displaystyle\frac{ h }{2 d }$ |
ID:(9779, 0)
0
Video
Video: Espejo Plano