User:
Moment of inertia
Storyboard 
The moment of inertia is the rotating factor that is equivalent to the mass in the translation.
The moment of inertia can be determined empirically by rotating a body around an axis or calculating how the mass is distributed around the axis.
ID:(600, 0)
Moment of inertia of a particle off axis
Equation
A simple application of the Steiner's theorem is a point mass $m$ at a distance $L$ from an axis. Since a point mass has no dimensions, it has no moment of inertia with respect to its center of mass. However, since the center of mass is at a distance of $L$ from the axis according to Steiner's theorem,
| $ I = I_{CM} + m d ^2$ |
its moment of inertia will be
 $ I = m L ^2$ |
.
ID:(9880, 0)
Moment of inertia calculation method
Equation
The total moment of inertia $I_t$ of an object is calculated by summing the moments of inertia of its components that are comparable to the moment of inertia of an individual particle,
| $ I = m r ^2$ |
resulting in a moment of inertia as
| $I_t=\sum_kI_k$ |
.
ID:(4438, 0)
Calculo del momento de inercia de un cuerpo
Equation
Si el eje no varia y la distribución de la masa no varia el momento de inercia es constante se tiene que es
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
Para calcular el momento de inercia de un cuerpo se debe considerar este desglosado en pequeños volúmenes que se suman para obtener el momento de inercia total
| $I_t=\sum_kI_k$ |
Como los momentos de inercia de masas
| $ I = m r ^2$ |
\\n\\npor lo que si se define la masa como la densidad
$I=\displaystyle\sum_k I_k =\displaystyle\sum_k m_k r_k^2 = \displaystyle\sum_k \rho r_k^2 dV \rightarrow \displaystyle\int_V \rho r^2 dV$
por lo que
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
ID:(10583, 0)
Bar that rotates around an axis $\perp$
Image
A bar with mass $m$ and length $l$ rotating around its center, which coincides with the center of mass:
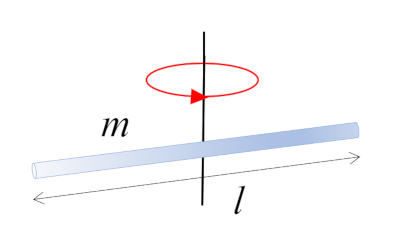
ID:(10962, 0)
Moment of inertia of bar length $l$ axis $\perp$
Equation
The moment of inertia of a rod rotating around a perpendicular ($\perp$) axis passing through the center is obtained by dividing the body into small volumes and summing them:
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
resulting in
| $ I_{CM} =\displaystyle\frac{1}{12} m l ^2$ |
.
ID:(4432, 0)
Cylinder rotating around axis $\parallel$
Image
A rotation of a cylinder with mass $m$ and radius $r$ around the axis of the cylinder, where the center of mass (CM) is located at mid-height:
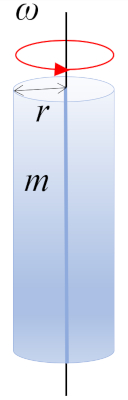
ID:(10964, 0)
Cylinder moment of inertia, axis $\parallel$
Equation
The moment of inertia of a cylinder rotating around an axis parallel ($\parallel$) to its central axis is obtained by segmenting the body into small volumes and summing them:
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
resulting in
| $ I_{CM} =\displaystyle\frac{1}{2} m r_c ^2$ |
.
ID:(4434, 0)
Cylinder that rotates about axis $\perp$
Image
In this scenario, a cylinder with mass $m$, radius $r$, and height $h$ is rotating around an axis perpendicular to its own axis. This axis passes through the midpoint of the cylinder's length, where the center of mass (CM) is located:
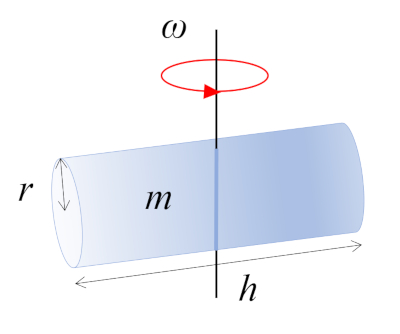
ID:(10965, 0)
Cylinder moment of inertia, axis $\perp$
Equation
The moment of inertia of a cylinder rotating around a perpendicular ($\perp$) axis passing through the center is obtained by segmenting the body into small volumes and summing them:
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
resulting in
| $ I_{CM} =\displaystyle\frac{1}{12} m ( h ^2+3 r_c ^2)$ |
.
ID:(4435, 0)
Regular parallelepiped moment of inertia
Image
A straight rectangular parallelepiped with mass $m$ and sides $a$ and $b$, perpendicular to the axis of rotation, is rotating around its center of mass, which is located at the geometric center of the body:
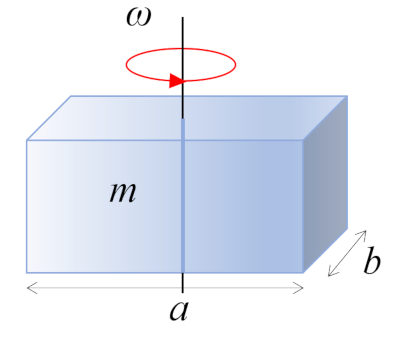
ID:(10973, 0)
Moment of inertia of a right parallelepiped
Equation
The moment of inertia of a parallelepiped rotating around an axis passing through its center is obtained by partitioning the body into small volumes and summing them up:
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
resulting in
| $ I_{CM} =\displaystyle\frac{1}{12} m ( a ^2+ b ^2)$ |
.
ID:(4433, 0)
Straight parallelepiped
Image
In the case of a right rectangular parallelepiped with mass $m$ and side $a$, the center of mass is located at the geometric center:
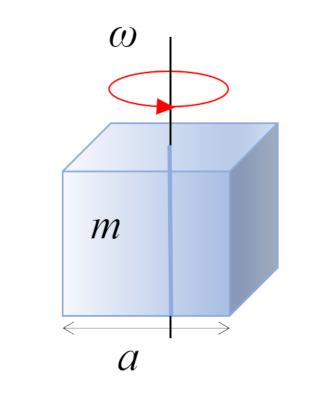
ID:(10963, 0)
Momento de inercia de cubo recto
Equation
El momento de inercia de un cubo que rota en torno a un eje que pasa por el centro se obtiene segmentando el cuerpo en pequeños volúmenes sumando:
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
resultando
| $ I_{CM} =\displaystyle\frac{1}{6} m a ^2$ |
ID:(10972, 0)
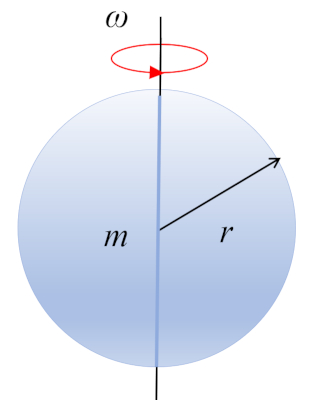
Moment of inertia of a sphere
Equation
The moment of inertia of a sphere rotating around an axis passing through its center is obtained by segmenting the body into small volumes and summing:
| $ I =\displaystyle\int_V r ^2 \rho dV $ |
resulting in
| $ I_{CM} =\displaystyle\frac{2}{5} m r_e ^2$ |
.
ID:(4436, 0)
0
Video
Video: Moment of inertia