User:
First Law of Thermodynamics
Storyboard 
The first law of thermodynamics states that energy is conserved and consists of two components: one associated with work and the other with heat. Unlike work, this latter component cannot be completely converted into work, which limits the possible energy conversions.
ID:(1398, 0)
Mechanisms
Iframe
The first law of thermodynamics, or the law of energy conservation, states that energy cannot be created or destroyed in an isolated system; it can only be transferred or transformed. This principle asserts that the total energy of an isolated system remains constant. In practical terms, this means the change in internal energy of a system is equal to the heat added to the system minus the work done by the system. Internal energy encompasses the total energy within a system, including kinetic and potential energy at the molecular level. Heat is the energy transferred between systems due to a temperature difference, while work is the energy transferred when a force is applied over a distance, often related to volume changes in gases. The first law is fundamental in analyzing the efficiency of heat engines, the performance of refrigeration and heating systems, and understanding metabolic processes in biological systems. It provides a basis for understanding energy transfers and transformations in various physical processes, ensuring that the energy within an isolated system is always conserved.
Mechanisms
ID:(15250, 0)
Energy conservation
Concept
The first law of thermodynamics states that energy is always conserved.
While in mechanics a similar conservation is stated, restricted to non-dissipative systems (for example, excluding friction), thermodynamics generalizes it by considering not only mechanical work but also heat generated or absorbed by the system.
In this sense, the conservation of energy postulated in thermodynamics has no restrictions and is applicable to all systems, as long as all possible energy exchanges and conversions are taken into account.
ID:(37, 0)
First law of thermodynamics
Concept
The first law of thermodynamics was developed through various works [1,2], establishing that energy is conserved. This means that the internal energy differential ($dU$) is always equal to the differential inexact Heat ($\delta Q$) supplied to the system (positive) minus the differential inexact labour ($\delta W$) done by the system (negative).
Therefore, we have:
| $ dU = \delta Q - \delta W $ |
While the exact differential does not depend on how the variation is executed, the inexact differential does. When we refer to a differential without specifying that it is inexact, it is assumed to be exact.
[1] "Über die quantitative und qualitative Bestimmung der Kräfte" (On the Quantitative and Qualitative Determination of Forces), Julius Robert von Mayer, Annalen der Chemie und Pharmacie, 1842
[2] "Über die Erhaltung der Kraft" (On the Conservation of Force), Hermann von Helmholtz, 1847
ID:(15700, 0)
Pressure and work
Description
Let's consider a gas in a cylinder where a piston can move. If the piston is displaced, the volume can be reduced by compressing the gas. To perform this compression, energy is required, which is equal to the force exerted by the gas multiplied by the distance traveled by the piston. This energy can also be represented in terms of pressure since pressure is defined by the force and the area of the piston.
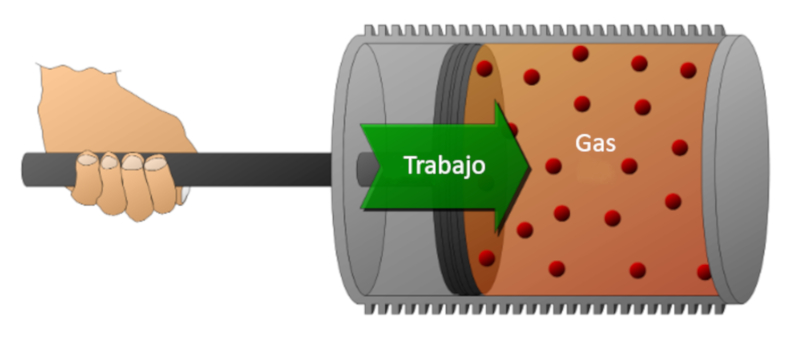
Work can be done on the system (compression) or by the system on the external environment (expansion).
Since the mechanical force ($F$) divided by the section ($S$) is equal to the pressure ($p$):
| $ p \equiv\displaystyle\frac{ F }{ S }$ |
and the volume Variation ($dV$) with the distance traveled ($dx$) is equal to:
| $ \Delta V = S \Delta s $ |
The equation for the differential inexact labour ($\delta W$) can be expressed as:
| $ \delta W = F dx $ |
So it can be written as:
| $ \delta W = p dV $ |
ID:(11126, 0)
First law of Thermodynamics and Pressure
Concept
Since the internal energy differential ($dU$) relates to the differential inexact Heat ($\delta Q$) and the differential inexact labour ($\delta W$) as shown below:
| $ dU = \delta Q - \delta W $ |
And it is known that the differential inexact labour ($\delta W$) is related to the pressure ($p$) and the volume Variation ($dV$) as follows:
| $ \delta W = p dV $ |
Therefore, we can conclude that:
| $ dU = \delta Q - p dV $ |
ID:(15701, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ dU = \delta Q - \delta W $
dU = dQ - dW
$ dU = \delta Q - p dV $
dU = dQ - p * dV
$ dV = S dx $
DV = S * Ds
$ \delta W = F dx $
dW = F * dx
$ \delta W = p dV $
dW = p * dV
$ p \equiv\displaystyle\frac{ F }{ S }$
p = F / S
ID:(15309, 0)
First law of thermodynamics
Equation
The internal energy differential ($dU$) is always equal to the amount of the differential inexact Heat ($\delta Q$) supplied to the system (positive) minus the amount of the differential inexact labour ($\delta W$) performed by the system (negative):
| $ dU = \delta Q - \delta W $ |
ID:(9632, 0)
Work
Equation
The relationship between work and our actions is linked to the dependence of the differential inexact labour ($\delta W$) on the distance traveled. If we consider a mechanical force ($F$) moving an object along a distance traveled ($dx$), the required energy can be expressed as:
| $ \delta W = F dx $ |
The notation $\delta W$ is used to indicate the variation in work, in contrast to $dW$, which reminds us that its value depends on the process of varying the length $dx$. An example of this would be if the displacement occurred in a gas and a change occurred in it, in which case:
$\delta W < Fdx$
ID:(3202, 0)
Definition of pressure
Equation
The water column pressure ($p$) is calculated from the column force ($F$) and the column Section ($S$) as follows:
| $ p \equiv\displaystyle\frac{ F }{ S }$ |
ID:(4342, 0)
Volume element
Equation
If we have a tube with a the section Tube ($S$) moving a distance the tube element ($\Delta s$) along its axis, having displaced the volume element ($\Delta V$), then it is equal to:
| $ dV = S dx $ |
| $ \Delta V = S \Delta s $ |
ID:(3469, 0)
Work and pressure
Equation
The differential inexact labour ($\delta W$) is equal to the pressure ($p$) multiplied by the volume Variation ($dV$):
| $ \delta W = p dV $ |
Since the mechanical force ($F$) divided by the section ($S$) is equal to the pressure ($p$):
| $ p \equiv\displaystyle\frac{ F }{ S }$ |
and the volume Variation ($dV$) with the distance traveled ($dx$) is equal to:
| $ \Delta V = S \Delta s $ |
The equation for the differential inexact labour ($\delta W$) can be expressed as:
| $ \delta W = F dx $ |
So it can be written as:
| $ \delta W = p dV $ |
ID:(3468, 0)
First law of thermodynamics and pressure
Equation
With the first law of thermodynamics, it can be expressed in terms of the internal energy differential ($dU$), the differential inexact Heat ($\delta Q$), the pressure ($p$), and the volume Variation ($dV$) as:
| $ dU = \delta Q - p dV $ |
Since the internal energy differential ($dU$) relates to the differential inexact Heat ($\delta Q$) and the differential inexact labour ($\delta W$) as shown below:
| $ dU = \delta Q - \delta W $ |
And it is known that the differential inexact labour ($\delta W$) is related to the pressure ($p$) and the volume Variation ($dV$) as follows:
| $ \delta W = p dV $ |
Therefore, we can conclude that:
| $ dU = \delta Q - p dV $ |
ID:(3470, 0)