User:
Mechanisms
Iframe 
In thermodynamics, work is the energy transferred to or from a system by means of a force acting over a distance. This can occur in various forms such as mechanical work, where a gas in a piston-cylinder arrangement expands and moves the piston, or electrical work, involving the transfer of energy through electrical forces. The concept of work is crucial for understanding energy exchanges and processes. It is mathematically represented, often involving pressure and volume changes in the system. According to the first law of thermodynamics, the change in internal energy of a system is equal to the heat added to the system minus the work done by the system on its surroundings. Work is integral to various processes, including isothermal, adiabatic, isobaric, and isochoric processes, and is essential in applications like heat engines and refrigerators, where it plays a key role in converting heat into work or using work to transfer heat.
Mechanisms
ID:(15247, 0)
Working converter heat
Description
The conversion of work into energy is studied by generating heat through friction. To do this, a metal band is wrapped around a cylinder containing water and a thermometer. By turning the crank, heat is generated through friction, leading to the heating of the water. If the applied force, the number of rotations, and the radius of the cylinder are measured, the distance traveled can be estimated, allowing for the estimation of energy as the product of force and distance.
ID:(1884, 0)
Pressure and work
Description
Let's consider a gas in a cylinder where a piston can move. If the piston is displaced, the volume can be reduced by compressing the gas. To perform this compression, energy is required, which is equal to the force exerted by the gas multiplied by the distance traveled by the piston. This energy can also be represented in terms of pressure since pressure is defined by the force and the area of the piston.
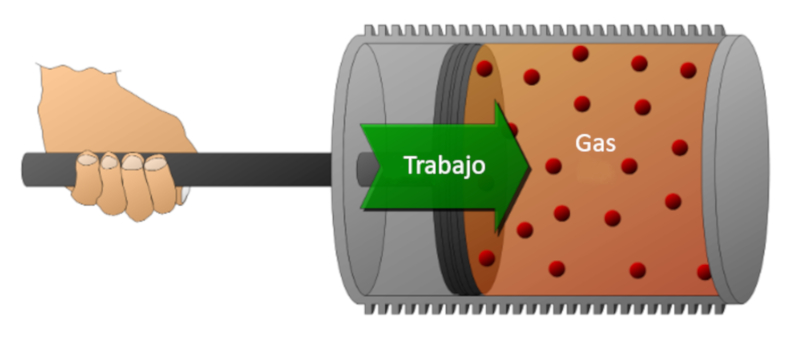
Work can be done on the system (compression) or by the system on the external environment (expansion).
Since the mechanical force ($F$) divided by the section ($S$) is equal to the pressure ($p$):
| $ p \equiv\displaystyle\frac{ F }{ S }$ |
and the volume Variation ($dV$) with the distance traveled ($dx$) is equal to:
| $ \Delta V = S \Delta s $ |
The equation for the differential inexact labour ($\delta W$) can be expressed as:
| $ \delta W = F dx $ |
So it can be written as:
| $ \delta W = p dV $ |
ID:(11126, 0)
Pressure and work on a gas
Description
As the piston moves within the cylinder, it acts like a tennis racket, imparting kinetic energy to the molecules and increasing their speed. As these molecules gain greater velocity, they transfer a higher momentum to the walls, resulting in an increased pressure.
ID:(11127, 0)
Pressure and work in a liquid/solid
Description
In the case of a liquid or solid, an external force displaces the particles of the material, which affects the potential energy of the bonds between them. This can be imagined as small springs being compressed, thereby increasing their potential energy.
ID:(11128, 0)
Model
Top
Parameters
Variables
Calculations
to 
, then, select the variable: 
to 
Calculations
Calculations
Variable 
Given Calculate 
Target : Equation To be used 
Equations
$ \delta W = F dx $
dW = F * dx
$ \delta W = p dV $
dW = p * dV
$ W = \displaystyle\int_{V_1}^{V_2}p\,dV$
W = @INT( p , V , V_1 , V_2 )
ID:(15306, 0)
Work
Equation
The relationship between work and our actions is linked to the dependence of the differential inexact labour ($\delta W$) on the distance traveled. If we consider a mechanical force ($F$) moving an object along a distance traveled ($dx$), the required energy can be expressed as:
| $ \delta W = F dx $ |
The notation $\delta W$ is used to indicate the variation in work, in contrast to $dW$, which reminds us that its value depends on the process of varying the length $dx$. An example of this would be if the displacement occurred in a gas and a change occurred in it, in which case:
$\delta W < Fdx$
ID:(3202, 0)
Work and pressure
Equation
The differential inexact labour ($\delta W$) is equal to the pressure ($p$) multiplied by the volume Variation ($dV$):
| $ \delta W = p dV $ |
Since the mechanical force ($F$) divided by the section ($S$) is equal to the pressure ($p$):
| $ p \equiv\displaystyle\frac{ F }{ S }$ |
and the volume Variation ($dV$) with the distance traveled ($dx$) is equal to:
| $ \Delta V = S \Delta s $ |
The equation for the differential inexact labour ($\delta W$) can be expressed as:
| $ \delta W = F dx $ |
So it can be written as:
| $ \delta W = p dV $ |
ID:(3468, 0)
0
Video
Video: Work