Charles Law
Storyboard 
Charles's law states that the ratio of the volume ($V$) to the absolute temperature ($T$) of a gas remains constant, provided that the pressure and the number of moles remain unchanged.
This implies that the volume ($V$) varies proportionally with the absolute temperature ($T$).
ID:(1473, 0)
Mechanisms
Iframe 
Charles's law states that the volume of a gas is directly proportional to its temperature when the pressure is held constant. This means that as the temperature of a gas increases, its volume increases as well, provided the pressure remains the same. Conversely, if the temperature decreases, the volume decreases. This relationship is fundamental in understanding the behavior of gases and is often observed when heating or cooling a gas in a flexible container, such as a balloon, where the change in temperature results in a noticeable change in volume.
Mechanisms
ID:(15255, 0)
Volume and temperature variation
Concept 
The generation of the pressure ($p$) occurs when gas particles collide with the surface of the gas container. Each collision transmits a momentum equal to twice the particle mass ($m$) times the average speed of a particle ($\bar{v}$). Additionally, it is important to consider the particle flow towards the surface, which depends on the particle concentration ($c_n$) but also on the average speed of a particle ($\bar{v}$) with which they move. Therefore,
$p \propto c_n v \cdot m v = c_n m v^2$
The particle flow and momentum transmission are depicted in the following graph:

Given that the particle mass ($m$) times the average speed of a particle ($\bar{v}$) squared is proportional to energy, and this to the absolute temperature ($T$),
$mv^2 \propto E \propto T$
and, since the number of particles ($N$) is constant, the particle concentration ($c_n$) is inversely proportional to the volume ($V$):
$c_n \propto \displaystyle\frac{1}{V}$
Since the pressure ($p$) is constant, we have:
$p \propto \displaystyle\frac{T}{V}$
implying:
$V \propto T$
ID:(15689, 0)
Meaning of Absolute Temperature
Description 
Temperature corresponds to the thermal energy (motion) contained within an object.
Since energy is always positive, temperature scales like Celsius and Fahrenheit, which can assume negative values, may seem counterintuitive. However, the relationship between energy and temperature leads to the conclusion that there must exist an absolute zero temperature, where the energy of a system of particles is zero.
Furthermore, in 1802, Gay-Lussac observed that in gases, there is a proportional relationship between volume and temperature. This proportionality implies that a gas would reach zero volume at a temperature of -273.15 degrees Celsius (according to modern measurements), which is known as absolute zero temperature:
This implies the existence of such a scale, which has been termed the absolute temperature scale measured in Kelvin degrees. Other scales, such as temperatures $t$ in Celsius or Fahrenheit degrees, can be converted to
Celsius:
$T = 273.15 + t$
Fahrenheit:
$T = 255.37 + \displaystyle\frac{5}{9} t$
ID:(111, 0)
Equal temperature differences Celsius and Kelvin
Concept 
The temperature difference in degrees Celsius ($\Delta t$) combined with the temperature in degrees Celsius in state 1 ($t_1$) and the temperature in degrees Celsius in state 2 ($t_2$) results in:
$\Delta t = t_2 - t_1$
If we express both temperatures in Kelvin degrees, we have that the temperature in state 1 ($T_1$) is equal to:
$T_1 = t_1 + 273.15 , \text{K}$
and the temperature in state 2 ($T_2$) is equal to:
$T_2 = t_2 + 273.15 , \text{K}$
Therefore, the difference in temperatures in Kelvin, denoted as the temperature difference ($\Delta T$), is calculated as:
$\Delta T=T_2-T_1=t_2-t_1=\Delta t$
This implies:
| $ \Delta T = \Delta t $ |
Which means that the difference between temperatures in Celsius and Kelvin degrees remains the same.
ID:(15694, 0)
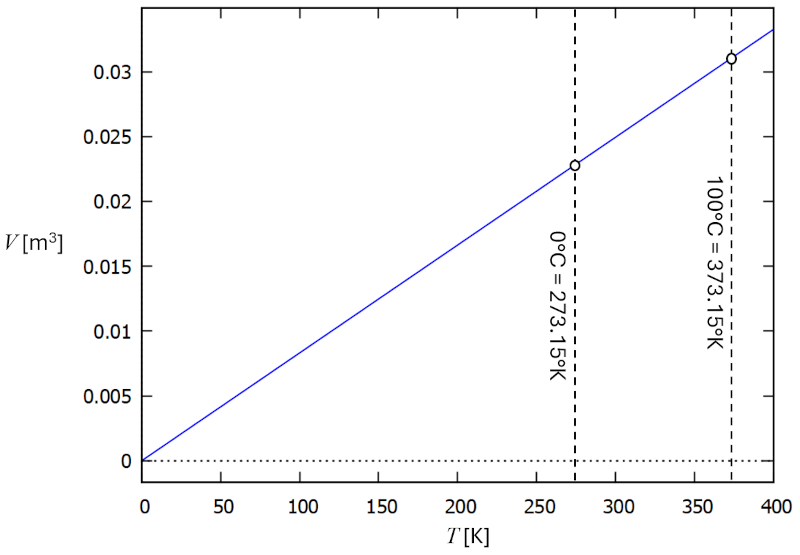
Temperature vs volume relationship
Description 
In a gas, if the pressure ($p$) and the number of particles ($N$) are held constant, it is observed that the volume ($V$) and the absolute temperature ($T$) vary in proportion. When the volume ($V$) decreases, the absolute temperature ($T$) also decreases, and vice versa,
$V \propto T$
as shown in the following graph:

Charles's law [1] states that, with the pressure ($p$) and the number of particles ($N$) held constant, the volume ($V$) and the absolute temperature ($T$) are directly proportional.
This can be expressed with the charles law constant ($C_c$) as follows:
| $\displaystyle\frac{ V }{ T } = C_c$ |
![]() [1] "Sur la dilatación de los gases y vapores" (On the Expansion of Gases and Vapors), Jacques Charles, French Academy of Sciences (1787)
[1] "Sur la dilatación de los gases y vapores" (On the Expansion of Gases and Vapors), Jacques Charles, French Academy of Sciences (1787)
ID:(9529, 0)
Jacques Charles
Description 
Jacques Charles, born on November 12, 1746, and died on April 7, 1823, was a French physicist, inventor, and balloonist. He is best known for his pioneering work in the field of aeronautics and for his discoveries related to the behavior of gases. In 1783, he launched the world's first manned hydrogen-filled balloon flight, along with the chemist Nicolas-Louis Robert. This successful flight marked a significant milestone in the history of aviation. Charles also made important contributions to the understanding of gas laws, including what is now known as Charles's Law, which describes the relationship between the volume and temperature of a gas at constant pressure.
Note: Charles's law was made public by Joseph Louis Gay-Lussac, who attributed it to Jacques Charles, citing an unpublished article.

ID:(1656, 0)
Change of state of an ideal gas according to Charles's law
Concept 
Charles's law states that, with the pressure ($p$) constant, the ratio of the volume ($V$) to the absolute temperature ($T$) equals the charles law constant ($C_c$):
| $\displaystyle\frac{ V }{ T } = C_c$ |
This implies that if a gas transitions from an initial state (the volume in state i ($V_i$) and the temperature in initial state ($T_i$)) to a final state (the volume in state f ($V_f$) and the temperature in final state ($T_f$)), while keeping the pressure ($p$) constant, it must always comply with Charles's law:
$\displaystyle\frac{V_i}{T_i} = C_c = \displaystyle\frac{V_f}{T_f}$
Therefore, we have:
| $\displaystyle\frac{ V_i }{ T_i }=\displaystyle\frac{ V_f }{ T_f }$ |
ID:(15692, 0)
Equivalent to Charles's law for densities
Concept 
In an isobaric process where the content remains constant, if the volume in state i ($V_i$), the temperature in final state ($T_f$), the temperature in initial state ($T_i$), and the temperature in final state ($T_f$) are related by:
| $\displaystyle\frac{ V_i }{ T_i }=\displaystyle\frac{ V_f }{ T_f }$ |
Then, we can introduce the density ($\rho$), which, along with the mass ($M$) and the volume ($V$), satisfies:
| $ \rho \equiv\displaystyle\frac{ M }{ V }$ |
This leads us to the density in state i ($\rho_i$) and the density in state f ($\rho_f$) as:
| $ \rho_i T_i = \rho_f T_f $ |
ID:(15693, 0)
Model
Top 
Parameters
Variables
Calculations
Calculations
Calculations
Equations
$ \rho_i \equiv\displaystyle\frac{ M }{ T_i }$
rho = M / V
$ \rho_f \equiv\displaystyle\frac{ M }{ T_f }$
rho = M / V
$ \rho_i T_i = \rho_f T_f $
rho_1 * T_1 = rho_2 * T_2
$\displaystyle\frac{ V_i }{ T_i } = C_c$
V / T = C_c
$\displaystyle\frac{ V_f }{ T_f } = C_c$
V / T = C_c
$\displaystyle\frac{ V_i }{ T_i }=\displaystyle\frac{ V_f }{ T_f }$
V_i / T_i = V_f / T_f
ID:(15314, 0)
Charles Law (1)
Equation 
Charles's law establishes a relationship between the volume ($V$) and the absolute temperature ($T$), indicating that their ratio is equal to the charles law constant ($C_c$), as follows:
ID:(583, 1)
Charles Law (2)
Equation 
Charles's law establishes a relationship between the volume ($V$) and the absolute temperature ($T$), indicating that their ratio is equal to the charles law constant ($C_c$), as follows:
ID:(583, 2)
Change of state of an ideal gas according to Charles's law
Equation 
If a gas transitions from an initial state (i) to a final state (f) with the pressure ($p$) constant, then for the volume in state i ($V_i$), the volume in state f ($V_f$), the temperature in initial state ($T_i$), and the temperature in final state ($T_f$):
Charles's law states that, with the pressure ($p$) constant, the ratio of the volume ($V$) to the absolute temperature ($T$) equals the charles law constant ($C_c$):
| $\displaystyle\frac{ V }{ T } = C_c$ |
This implies that if a gas transitions from an initial state (the volume in state i ($V_i$) and the temperature in initial state ($T_i$)) to a final state (the volume in state f ($V_f$) and the temperature in final state ($T_f$)), while keeping the pressure ($p$) constant, it must always comply with Charles's law:
$\displaystyle\frac{V_i}{T_i} = C_c = \displaystyle\frac{V_f}{T_f}$
Therefore, we have:
| $\displaystyle\frac{ V_i }{ T_i }=\displaystyle\frac{ V_f }{ T_f }$ |
ID:(3492, 0)
Mass and Density (1)
Equation 
The density ($\rho$) is defined as the ratio between the mass ($M$) and the volume ($V$), expressed as:
This property is specific to the material in question.
ID:(3704, 1)
Mass and Density (2)
Equation 
The density ($\rho$) is defined as the ratio between the mass ($M$) and the volume ($V$), expressed as:
This property is specific to the material in question.
ID:(3704, 2)
Equivalent to Charles's law for densities
Equation 
For the initial states (the density in state i ($\rho_i$), the volume in state i ($V_i$)) and final states (the density in state f ($\rho_f$), the volume in state f ($V_f$)), the law of Charles is obtained as follows:
In an isobaric process where the content remains constant, if the volume in state i ($V_i$), the temperature in final state ($T_f$), the temperature in initial state ($T_i$), and the temperature in final state ($T_f$) are related by:
| $\displaystyle\frac{ V_i }{ T_i }=\displaystyle\frac{ V_f }{ T_f }$ |
Then, we can introduce the density ($\rho$), which, along with the mass ($M$) and the volume ($V$), satisfies:
| $ \rho \equiv\displaystyle\frac{ M }{ V }$ |
This leads us to the density in state i ($\rho_i$) and the density in state f ($\rho_f$) as:
| $ \rho_i T_i = \rho_f T_f $ |
ID:(8835, 0)
