User:
Darcy's Law
Storyboard
Hagen-Poiseuille's law for total flow can be redefined in terms of a pressure difference, flow rate, and a factor that can be characterized as hydraulic resistance, thus leading to what is known as Darcy's law.
ID:(877, 0)
Laminar flow through a tube
Concept
When a tube filled with liquid with a viscosity of viscosity ($\eta$) is exposed to the pressure in the initial position ($p_i$) at the position at the beginning of the tube ($L_i$) and the pressure in end position (e) ($p_e$) at the position at the end of the tube ($L_e$), it generates a pressure difference ($\Delta p$) along the tube length ($\Delta L$), resulting in the profile of the speed on a cylinder radio ($v$):
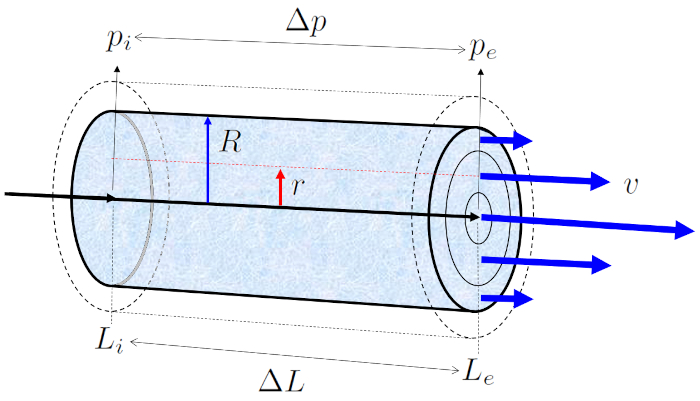
In flows with low values of the number of Reynold ($Re$), where viscosity is more significant than the inertia of the liquid, the flow develops in a laminar manner, meaning without the presence of turbulence.
ID:(2218, 0)
Hagen Poiseuille Equation
Equation
If we examine the profile of the speed on a cylinder radio ($v$) for a fluid within a cylindrical channel of radius cylinder radio ($R$), in which the speed on a cylinder radio ($v$) varies as a function of ($$), we can integrate it across the entire cross-section of the channel:
$J_V= \pi \displaystyle\int_0^Rdr r v(r)$
This leads to the Hagen-Poiseuille law with parameters the volume flow ($J_V$), the viscosity ($\eta$), the pressure difference ($\Delta p$), and the tube length ($\Delta L$):
 $ J_V =-\displaystyle\frac{ \pi R ^4}{8 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
If we consider the profile of speed on a cylinder radio ($v$) for a fluid in a cylindrical channel, where the speed on a cylinder radio ($v$) varies with respect to radius of position in a tube ($r$) according to the following expression:
| $ v = v_{max} \left(1-\displaystyle\frac{ r ^2}{ R ^2}\right)$ |
involving the cylinder radio ($R$) and the maximum flow rate ($v_{max}$). We can calculate the maximum flow rate ($v_{max}$) using the viscosity ($\eta$), the pressure difference ($\Delta p$), and the tube length ($\Delta L$) as follows:
| $ v_{max} =-\displaystyle\frac{ R ^2}{4 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
If we integrate the velocity across the cross-section of the channel, we obtain the volume flow ($J_V$), defined as the integral of $\pi r v(r)$ with respect to radius of position in a tube ($r$) from $0$ to cylinder radio ($R$). This integral can be simplified as follows:
$J_V=-\displaystyle\int_0^Rdr \pi r v(r)=-\displaystyle\frac{R^2}{4\eta}\displaystyle\frac{\Delta p}{\Delta L}\displaystyle\int_0^Rdr \pi r \left(1-\displaystyle\frac{r^2}{R^2}\right)$
The integration yields the resulting Hagen-Poiseuille law:
| $ J_V =-\displaystyle\frac{ \pi R ^4}{8 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
The original papers that gave rise to this law with a combined name were:
• Gotthilf Hagen: "Ueber die Gesetze, welche des der Strom des Wassers in röhrenförmigen Gefässen bestimmen" (On the laws governing the flow of water in cylindrical vessels), Annalen der Physik und Chemie 46:423442 (1839).
• Jean-Louis-Marie Poiseuille: "Recherches expérimentales sur le mouvement des liquides dans les tubes de très-petits diamètres" (Experimental research on the movement of liquids in tubes of very small diameters), Comptes Rendus de l'Académie des Sciences 9:433544 (1840).
ID:(3178, 0)
Hydraulic Conductance of a Pipe
Equation
If we examine the Hagen-Poiseuille law, which allows us to calculate the volume flow ($J_V$) from the cylinder radio ($R$), the viscosity ($\eta$), the tube length ($\Delta L$), and the pressure difference ($\Delta p$):
| $ J_V =-\displaystyle\frac{ \pi R ^4}{8 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
we can identify parameters related to geometry (the tube length ($\Delta L$) and the cylinder radio ($R$)) and the type of liquid (the viscosity ($\eta$)), which can be collectively referred to as a hydraulic conductance ($G_h$):
| $ G_h =\displaystyle\frac{ \pi R ^4}{8 \eta | \Delta L | }$ |
ID:(15102, 0)
Darcy's law and hydraulic conductance
Equation
With the introduction of the hydraulic conductance ($G_h$), we can rewrite the Hagen-Poiseuille equation with the pressure difference ($\Delta p$) and the volume flow ($J_V$) using the following equation:
| $ J_V = G_h \Delta p $ |
If we examine the Hagen-Poiseuille law, which allows us to calculate the volume flow ($J_V$) from the cylinder radio ($R$), the viscosity ($\eta$), the tube length ($\Delta L$), and the pressure difference ($\Delta p$):
| $ J_V =-\displaystyle\frac{ \pi R ^4}{8 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
we can introduce the hydraulic conductance ($G_h$), defined in terms of the tube length ($\Delta L$), the cylinder radio ($R$), and the viscosity ($\eta$), as follows:
| $ G_h =\displaystyle\frac{ \pi R ^4}{8 \eta | \Delta L | }$ |
to arrive at:
| $ J_V = G_h \Delta p $ |
ID:(14471, 0)
Hydraulic conductance
Equation
In the context of electrical resistance, there exists its inverse, known as electrical conductance. Similarly, what would be the hydraulic conductance ($G_h$) can be defined in terms of the hydraulic resistance ($R_h$) through the expression:
| $ R_h = \displaystyle\frac{1}{G_h }$ |
ID:(15092, 0)
Hydraulic resistance of a tube
Equation
Since the hydraulic resistance ($R_h$) is equal to the inverse of the hydraulic conductance ($G_h$), it can be calculated from the expression of the latter. In this way, we can identify parameters related to geometry (the tube length ($\Delta L$) and the cylinder radio ($R$)) and the type of liquid (the viscosity ($\eta$)), which can be collectively referred to as a hydraulic resistance ($R_h$):
| $ R_h =\displaystyle\frac{8 \eta | \Delta L | }{ \pi R ^4}$ |
Since the hydraulic resistance ($R_h$) is equal to the hydraulic conductance ($G_h$) as per the following equation:
| $ R_h = \displaystyle\frac{1}{G_h }$ |
and since the hydraulic conductance ($G_h$) is expressed in terms of the viscosity ($\eta$), the cylinder radio ($R$), and the tube length ($\Delta L$) as follows:
| $ G_h =\displaystyle\frac{ \pi R ^4}{8 \eta | \Delta L | }$ |
we can conclude that:
| $ R_h =\displaystyle\frac{8 \eta | \Delta L | }{ \pi R ^4}$ |
ID:(3629, 0)
Darcy's law and hydraulic resistance
Equation
Since the volume flow ($J_V$) can be calculated from the hydraulic conductance ($G_h$) and the pressure difference ($\Delta p$) using the following equation:
| $ J_V = G_h \Delta p $ |
it can be expressed in terms of the pressure difference ($\Delta p$). Considering that the inverse of the hydraulic resistance ($R_h$) is the hydraulic conductance ($G_h$), we arrive at the following expression:
| $ \Delta p = R_h J_V $ |
In the case of a single cylinder the hydraulic resistance ($R_h$), which depends on the viscosity ($\eta$), the tube length ($\Delta L$), and the cylinder radio ($R$), it is calculated using the following equation:
| $ R_h =\displaystyle\frac{8 \eta | \Delta L | }{ \pi R ^4}$ |
On the other hand, Hagen-Poiseuille's law allows us to calculate the volume flow ($J_V$) generated by the pressure difference ($\Delta p$) according to the equation:
| $ J_V =-\displaystyle\frac{ \pi R ^4}{8 \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
Combining both equations, we obtain Darcy's law:
| $ \Delta p = R_h J_V $ |
which Henry Darcy formulated to model the general behavior of more complex porous media through which a liquid flows.
The genius of this way of rewriting the Hagen-Poiseuille law is that it demonstrates the analogy between the flow of electric current and the flow of liquid. In this sense, Hagen-Poiseuille's law corresponds to Ohm's law. This opens up the possibility of applying the concepts of electrical networks to systems of pipes through which a liquid flows.
This law, also known as the Darcy-Weisbach Law, was first published in Darcy's work:
• "Les fontaines publiques de la ville de Dijon" ("The Public Fountains of the City of Dijon"), Henry Darcy, Victor Dalmont Editeur, Paris (1856).
ID:(3179, 0)
Volume Flow and its Speed
Equation
Flow is measured in the volume that passes through a section per time, which can finally be expressed as the section times an average flow velocity
| $ j_s = \displaystyle\frac{ J_V }{ S }$ |
Since the flow is defined as the volume
| $ J_V =\displaystyle\frac{ \Delta V }{ \Delta t }$ |
and the volume is equal to the
| $ dV = S ds $ |
As the path traveled
| $ j_s =\displaystyle\frac{ \Delta s }{ \Delta t }$ |
you get that the flow is
| $ j_s = \displaystyle\frac{ J_V }{ S }$ |
Hay que tener presente que en este modelamiento:
La densidad de flujo cumple el rol de una velocidad media sobre toda la sección del flujo.
ID:(4349, 0)
Surface of a disk
Equation
The area the section ($S$) of a disk with a diameter of ($$) is calculated as follows:
| $ S = \pi r ^2$ |
ID:(3804, 0)
Hydraulic permeability
Equation
When analyzing the hydraulic conductance ($G_h$), it can be observed that in the numerator, the cross-sectional area of the tube is represented as $\pi R^2$, where the cylinder radio ($R$) corresponds to a property of the liquid, the viscosity ($\eta$) is related to the fluid's viscosity, and the tube length ($\Delta L$) pertains to the pressure gradient generated.
| $ G_h =\displaystyle\frac{ \pi R ^4}{8 \eta | \Delta L | }$ |
The remaining factor is referred to as the hydrodynamic permeability ($k$), known as
| $ k = \displaystyle\frac{ R ^2}{8}$ |
.
ID:(108, 0)
Flow density between columns
Equation
In the case of a tube through which a liquid with the liquid density ($\rho_w$) flows due to the pressure difference ($\Delta p$) generated by ($$) under the influence of gravity represented by the gravitational Acceleration ($g$), and calculated using:
| $ \Delta p = \rho_w g \Delta h $ |
this can be employed in the Hagen-Poiseuille equation, along with the definition of the flux density ($j_s$) in terms of the volume flow ($J_V$), which in turn depends on the cylinder radio ($R$) and the tube length ($\Delta L$):
| $ j_s = -\displaystyle\frac{ k }{ \eta }\displaystyle\frac{ \Delta p }{ \Delta L }$ |
ID:(14470, 0)
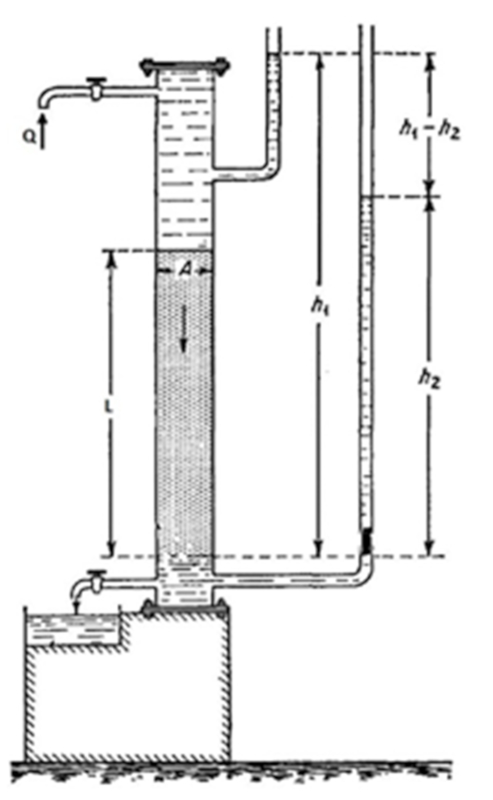
Measurement of Darcy\'s Law
Image
The Darcy\'s experiment involves a cylinder filled with a material under study, which is then filled with the desired liquid. There is a valve at the bottom to regulate the liquid outflow. Both the upper and lower parts have liquid columns associated with them to determine the existing pressures. By measuring the pressures, the amount of flowing liquid, and the elapsed time, the hydraulic resistance can be determined.
ID:(11104, 0)
0
Video
Video: Darcy's Law