User:
Tasa de plantación de semillas
Equation 
El plantar de semillas se puede modelar como un flujo de partículas en que la tasa
 $ R_s =\displaystyle\frac{ Q \rho }{ w v }$ |
ID:(12839, 0)
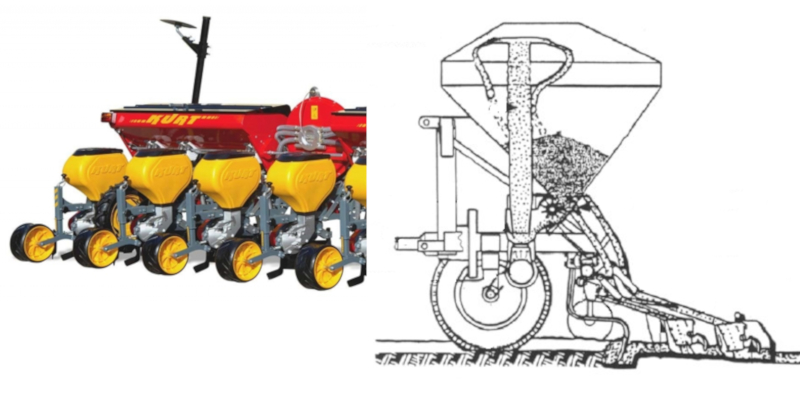
Plantador centrifugo
Image
La semillas pueden ser también distribuidas con un mecanismo centrifugo para su lanzamiento:
ID:(12899, 0)
Tangential speed
Equation
Si se divide el camino expresado como arco de un circulo se tendrá que con es
| $ \Delta s=r \Delta\theta $ |
por el tiempo transcurrido
| $ \bar{v} \equiv\displaystyle\frac{ \Delta s }{ \Delta t }$ |
y como la velocidad angular con es
| $ \bar{\omega} \equiv\displaystyle\frac{ \Delta\theta }{ \Delta t }$ |
se tiene con la relación
| $ v_t = r \omega $ |
ID:(10968, 0)
Road with constant acceleration
Equation
En el caso de que se asuma que la aceleración inicial es constante y tiempo inicial nulo la ecuación de la posición
| $ s = s_0 + v_0 ( t - t_0 )+\displaystyle\frac{1}{2} a_0 ( t - t_0 )^2$ |
se reduce a
| $ s = s_0 + v_0 t +\displaystyle\frac{1}{2} a_0 t ^2$ |
En el caso de que se asuma que el tiempo inicial es nulo\\n\\n
$t_0=0$
la ecuación de la posición
| $ s = s_0 + v_0 ( t - t_0 )+\displaystyle\frac{1}{2} a_0 ( t - t_0 )^2$ |
se reduce a
| $ s = s_0 + v_0 t +\displaystyle\frac{1}{2} a_0 t ^2$ |
ID:(4360, 0)
Horizontal distance traveled
Equation
The object cycles through a time ($t$) to a initial horizontal speed ($v_{0x}$) A position on the x-axis ($x$) equal to
| $ x = v_{0x} t $ |
The position ($s$) traveled with the constant velocity ($v_0$) with the starting position ($s_0$), the time ($t$), and the start Time ($t_0$) is
| $ s = s_0 + v_0 ( t - t_0 )$ |
Therefore, if the motion starts at the origin ($s_0=0$) at the beginning of time ($t_0=0$), the motion is described by $x=s$ and $v_0=v_{0x}$.
| $ x = v_{0x} t $ |
ID:(10930, 0)
Datos de sembrado
Image
Los parámetros claves para la plantación se dan como ejemplo para algunas especies
ID:(12841, 0)