User:
Oscillations
Storyboard 
There are different types of oscillators, the most discussed being closed by a spring and the pendulum. Both are relevant to study how we walk.
On the one hand there is the behavior similar to a spring that the muscles are capable of showing. On the other hand, when moving, there are systems such as arms that perform compensatory work oscillating with the same frequency of our steps.
In the case of the pendulum there are two types, the mathematician who considers the oscillation of a point mass and the physicist who considers the shape of the object as such.
ID:(51, 0)
Conservación en el caso de un Resorte
Equation
En el caso de un resorte la energía total
| $ K_t =\displaystyle\frac{1}{2} m_i v ^2$ |
donde
| $ V =\displaystyle\frac{1}{2} k x ^2$ |
\\n\\ndonde
$E_k=\displaystyle\frac{1}{2}m_iv^2+\displaystyle\frac{1}{2}kx^2$
Si uno reescribe esta expresión como
 $\displaystyle\frac{ v ^2}{\displaystyle\frac{2 E }{ m_i }}+\displaystyle\frac{ x ^2}{\displaystyle\frac{2 E }{ k }}=1$ |
\\n\\nse puede dar cuenta que corresponde a una elipse en el espacio velocidad
$a=\sqrt{\displaystyle\frac{2E_k}{k}}=x_0$
, y\\n\\n
$b=\sqrt{\displaystyle\frac{2E_k}{m_i}}=v_0$
.
Los semiejes corresponde a la vez a la velocidad
ID:(7101, 0)
Representación de la Elipse
Description
En el espacio de fase la oscilación se puede representar por una elipse
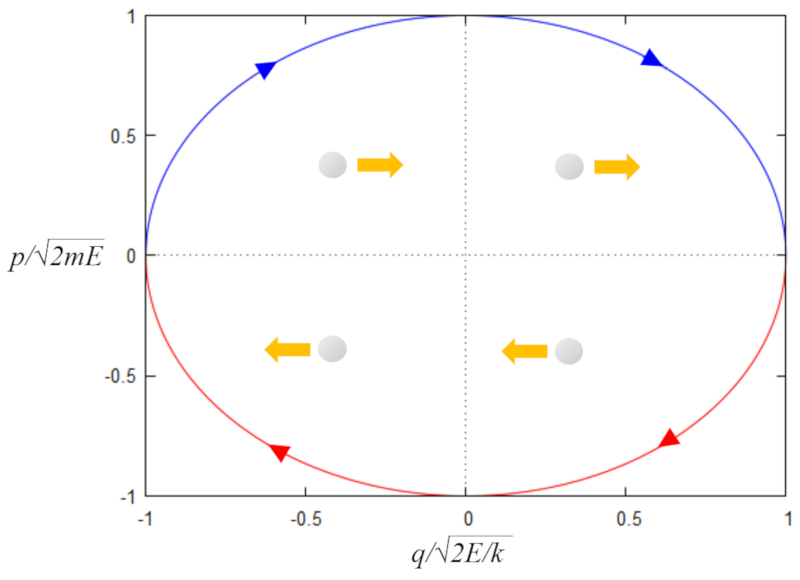
\\n \\nque en forma matemática se escribe como\\n\\n
$\displaystyle\frac{x^2}{a^2}+\displaystyle\frac{y^2}{b^2}=1$
\\n\\nde semiejes
$x=a\cos u$
\\n\\ny\\n\\n
$y=b\sin u$
ID:(7105, 0)
Representación de la Amplitud
Equation
En el caso de la amplitud, que corresponde a nuestra coordenada
$a=\sqrt{\displaystyle\frac{2E_k}{k}}$
\\n\\ny el tiempo se escala como\\n\\n
$u=\displaystyle\frac{2\pi t}{T}$
por lo que la amplitud será con igual a
| $ x =\sqrt{\displaystyle\frac{2 E }{ k }}\cos \displaystyle\frac{2 \pi t }{ T }$ |
ID:(7102, 0)
Representación de la Velocidad
Equation
En el caso de la velocidad, que corresponde a nuestra coordenada
$b=\sqrt{\displaystyle\frac{2E}{m}}$
\\n\\ny el tiempo se escala como\\n\\n
$u=\displaystyle\frac{2\pi t}{T}$
por lo que la velocidad será igual a
| $ v =-\sqrt{\displaystyle\frac{2 E }{ m_i }}\sin \displaystyle\frac{2 \pi t }{ T }$ |
ID:(7104, 0)
Periodo de la Oscilación
Equation
Como la oscilación cumple las leyes físicas se puede hacer uso del hecho que el area debajo de la curva velocidad vs tiempo el camino recorrido para determinar el perido. Como la velocidad es\\n\\n
$\displaystyle\int_0^{T/2}v(t)dt=\sqrt{\displaystyle\frac{2E}{m}}\displaystyle\int_0^{T/2}\cos \displaystyle\frac{2\pi t}{T}dt=\sqrt{\displaystyle\frac{2E}{m}}\displaystyle\frac{T}{\pi}$
\\n\\ny el camino entre un mínimo a un máximo de una elongación, lo que ocurre entre el tiempo
$x_{max}-x_{min}=2\sqrt{\displaystyle\frac{2E}{k}}$
se tiene que
| $ T =2 \pi \sqrt{\displaystyle\frac{ m_i }{ k }}$ |
ID:(7106, 0)
Frequency
Equation
The frequency ($\nu$) corresponds to the number of times an oscillation occurs within one second. The period ($T$) represents the time it takes for one oscillation to occur. Therefore, the number of oscillations per second is:
| $ \nu =\displaystyle\frac{1}{ T }$ |
Frequency is indicated in Hertz (Hz).
ID:(4427, 0)
Relación frecuencia angular - frecuencia
Equation
Como la frecuencia angular es con igual a
| $ \omega = \displaystyle\frac{2 \pi }{ T }$ |
y la frecuencia con frequency $Hz$ and period $s$ igual a
| $ \nu =\displaystyle\frac{1}{ T }$ |
se tiene que con frequency $Hz$ and period $s$ igual a
| $ \omega = 2 \pi \nu $ |
ID:(12338, 0)