Electric charges
Storyboard 
Electromagnetism focuses on the property of particles known as electric charge. This charge can be positive or negative and is generally an integer multiple of a fundamental unit of charge, which corresponds to the charge of an electron.
ID:(822, 0)
Electrostatic property
Image 
If we rub a comb and then approach it to pieces of paper we will see that the comb attracts these:

This is due to the fact that when rubbing we get loads from the comb to the object with which we rub it, being positively polarized or absorbing loads of the object with which it is rubbed and being negatively charged.
The loading of the comb leads to the paper loads separating, moving away those that are equal and approaching those that are different. This is because
>Same charges are repelled.
Y
>Opposite charges are attract.
When the polarized papers remain and the opposite charges are attracted, the papers are adhered to the comb.
ID:(1903, 0)
Bar after separation from the conductor
Concept 
If the charged body is separated, the repulsion between like charges causes them to distribute themselves over the surface of the conductor:

For this to occur, it is necessary for charges to be able to move within the body. Stephen Gray [1] identified this type of material, noting that they are conductors of electric charges. Conversely, he identified other materials in which this movement does not occur, describing them as insulators.
![]() [1] "Documented experiments - not a formal paper," Stephen Gray, Philosophical Transactions of the Royal Society, 1729-1730
[1] "Documented experiments - not a formal paper," Stephen Gray, Philosophical Transactions of the Royal Society, 1729-1730
ID:(1902, 0)
Polarization of a conductor
Image 
When approaching a loaded body to another in which the loads can move (conductor) a polarization originates:

Opposite charges are attracted while those equal to those of the approaching body are repelled.
ID:(1696, 0)
Bar in contact with conductor
Concept 
When a charged body is brought near a conductor, the conductor becomes polarized, causing opposite charges to move closer to the charged body. Upon contact, the charges transfer, neutralizing some of the charges on the charged body and leaving a surplus of charges on the conductor:

This phenomenon is also associated with the fact that like charges (positive or negative) repel each other, while opposite charges attract. This principle was introduced by Charles-Augustin de Coulomb [1] in his study of the forces between electric charges.
![]() [1] "Premier Mémoire sur lÉlectricité et le Magnétisme" (First memory on electricity and magnetism), Charles-Augustin de Coulomb, Académie Royale des Sciences in Paris, 1785.
[1] "Premier Mémoire sur lÉlectricité et le Magnétisme" (First memory on electricity and magnetism), Charles-Augustin de Coulomb, Académie Royale des Sciences in Paris, 1785.
ID:(1901, 0)
Charges are preserved
Concept 
A body can be neutral or polarized by separating positive charges from negative ones. However, this separation is such that the same number of positive charges are created as negative. This is a reflection of:
The sum of the positive and negative charges in the universe is preserved.
This means that the load itself is not preserved. In other words, a positive charge can 'disappear' as long as a negative charge 'disappears'. Similarly, a positive charge can only be 'created' to the extent that at the same time a negative charge is 'created'.
ID:(10389, 0)
Existence of Charges
Concept 
Experimenting with objects we can discover that there is a property that we can call load and that it has the following properties:
• they can be 'created from nothing' to the extent that they are 'created' the same number of positive as negative, which corresponds to a load conservation
• in some media, which we call conductors , these loads can be displaced
• the charges generate forces that can be repellent (equal charges) or attractants (opposite charges)
An additional property is that charges can only take discrete values, the 'unit' value being the charge of the electron.
ID:(10388, 0)
Change conservation example
Image 
A classic example of charge conservation occurs when a photon in a gamma ray generates a particle pair consisting of an electron and a positron. The original photon carries no charge, while the electron has a charge of -1.6e-19 Coulombs (negative) and the positron, which is the antimatter counterpart of the electron, carries a charge of +1.6e-19 Coulombs (positive).
This phenomenon could be observed in the old cloud chambers used by physicists to study particles:
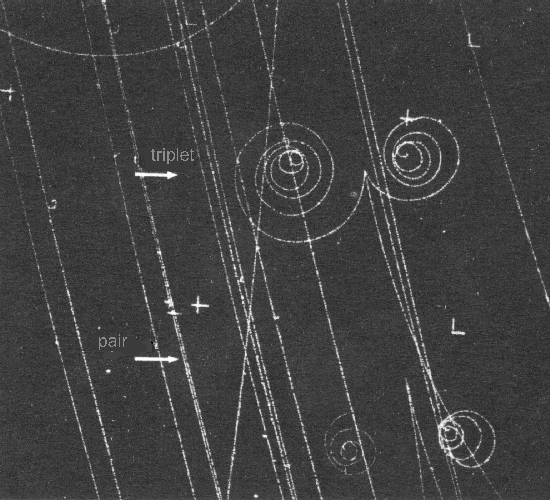
In these chambers, the particles induce condensation in the saturated water vapor, leaving visible trails. Since the chamber is placed in a magnetic field, the electrical charges are deflected, creating spiral-like images. With opposite charges, one particle spirals in one direction while the other spirals in the opposite direction.
ID:(11362, 0)
Number of electrons
Concept 
The electron was discovered by J.J. Thomson [1,2], who also determined its charge, $e$, to be $-1.6\times 10^{-19}C$. Therefore, the number of electrons ($n_e$) can be determined from the charge of all electrons ($Q_e$) divided by the electron Charge ($e$), resulting in:
| $ n_e =\displaystyle\frac{ Q_e }{ e }$ |
![]() [1] "Cathode Rays," J.J. Thomson, Philosophical Magazine, 1897
[1] "Cathode Rays," J.J. Thomson, Philosophical Magazine, 1897
![]() [2] "On the Charge of Electricity Carried by the Ions Produced by Röntgen Rays," J.J. Thomson, Philosophical Magazine, 1897
[2] "On the Charge of Electricity Carried by the Ions Produced by Röntgen Rays," J.J. Thomson, Philosophical Magazine, 1897
ID:(15777, 0)
Number of ions
Concept 
Svante Arrhenius, in his doctoral thesis [1], postulated that there are particles with multiple charges of the electron, known as ions. Therefore, their number depends on the total charge and the multiple of the electron's charge. Thus, the number of k-type ions ($n_{i,k}$) is calculated from the charge of the ion i ($Q_i$) divided by the charge of each ion, which is the electron Charge ($e$), multiplied by the valency ($z$):
| $ n_{i,k} =\displaystyle\frac{ Q_{i,k} }{ z_k e }$ |
![]() [1] "Recherches sur la conductibilité galvanique des électrolytes" (Research on the Galvanic Conductivity of Electrolytes), Svante Arrhenius, Doctoral Thesis, 1884
[1] "Recherches sur la conductibilité galvanique des électrolytes" (Research on the Galvanic Conductivity of Electrolytes), Svante Arrhenius, Doctoral Thesis, 1884
ID:(15776, 0)
Total charge
Concept 
The charge ($Q$) total can be calculated by summing the number of electrons ($n_e$) multiplied by the electron Charge ($e$) and the sum of the number of k-type ions ($n_{i,k}$) multiplied by the valency ($z$), with this number then multiplied by the electron Charge ($e$):
| $ Q = n_e e + \displaystyle\sum_k n_{i,k} z_k e $ |
As opposite charges cancel each other out, it is not possible to determine the total number of charges, nor is it possible to know how many of these are positive or negative.
ID:(15778, 0)
Model
Top 
Parameters
Variables
Calculations
Calculations
Calculations
Equations
$ n_e =\displaystyle\frac{ Q_e }{ e }$
n_e = Q_e / e
$ n_{i,k} =\displaystyle\frac{ Q_{i,k} }{ z_k e }$
n_ik = Q_ik /( z_k * e )
$ Q = n_e e + \displaystyle\sum_k n_{i,k} z_k e $
Q = n_e * e + @SUM( n_ik * z_k , k )* e
ID:(15322, 0)
Number of electrons
Equation 
The number of electrons ($n_e$) can be determined from the charge of all electrons ($Q_e$) divided by the electron Charge ($e$), resulting in:
ID:(3211, 0)
Number of ions
Equation 
The number of k-type ions ($n_{i,k}$) is calculated from the charge of the ion i ($Q_i$) divided by the charge of each ion, which is the electron Charge ($e$), multiplied by the valency ($z$):
ID:(15774, 0)
Total charge
Equation 
The charge ($Q$) total can be calculated by adding the number of electrons ($n_e$) multiplied by the electron Charge ($e$) and the sum of the number of k-type ions ($n_{i,k}$) multiplied by the valency ($z$), if this number is multiplied by the electron Charge ($e$):
ID:(15775, 0)
