Storyboard

>Model

ID:(775, 0)

Image

>Top

The heat conducted by a S section and long L depends on the temperature difference

\Delta T=T_2-T_1

and the conductivity \lambda:

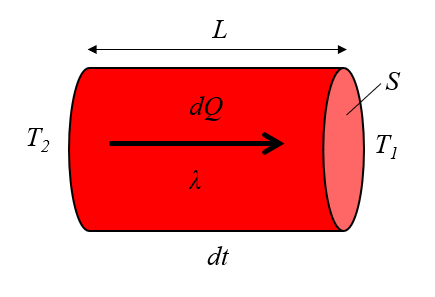
ID:(7718, 0)

Equation

>Top, >Model

In this manner, a relationship is established that allows us to calculate the variation of heat ($dQ$) based on the time variation ($dt$), the section ($S$), the conductor length ($L$), the temperature difference in the conductor ($\Delta T_0$), and the thermal conductivity ($\lambda$):

$\displaystyle\frac{ dQ }{ dt } = \displaystyle\frac{ S }{ L } \lambda \Delta T_0 $

Conditions

Variables

Relation

ID:(7712, 0)

Description

>Top

El motor de la conducción de calor es la diferencia de temperatura que existe entre los dos extremos de un cuerpo. Si ambas temperaturas son iguales no existiría flujo de calor.

En el caso de calefacción la diferencia de temperatura entre la del agua del calefactor y la temperatura ambiente interior genera el flujo de calor para calentar la habitación. Para calefacción crital el tubo se encuentra inmerso en un cemento que tiene típicamente una constante de conducción $\lambda$ del orden de $1.5,J/m^2sK$. Adicionalmente puede existir un revestimiento con baldosas, con un $\lambda$ de $1,J/m^2sK$ o parqué con un $\lambda$ de $0.17,J/m^2sK$.

> Diferencia de temperatura necesaria

>

> Si se requiere que atraviesen $40,W/m^2$ y se tiene que el coeficiente de conducción es del orden de $1,J/m^2sK$, siendo el grosor de la pared de $1,cm$ se tiene que se requiere de una diferencia de temperatura de

>

>$\Delta T=\displaystyle\frac{L}{\lambda}\displaystyle\frac{\dot{Q}}{S}=\displaystyle\frac{0.01,m}{1,J/m^2sK}\displaystyle\frac{40,W/m^2}{1\m^2}=0.4,K$

>

>Si el grosor de la pared fuera diez veces mayor o la conductividad un décimo se requiere de una diferencia de cuatro grados.

Cabe hacer notar que la diferencia de temperatura aquí calculado es aquel entre las dos superficies del medio. Como la temperatura aumenta/disminuye dentro del medio liquido/gaseoso en contacto, la diferencia total entre ambos medios es mayor a la entre ambas caras del conductor.

ID:(7731, 0)

Image

>Top

Heat is transferred between the S section of a medium at temperature T_1 and the contact section of a second medium at temperature T_2 is a function of the transfer constant \alpha:

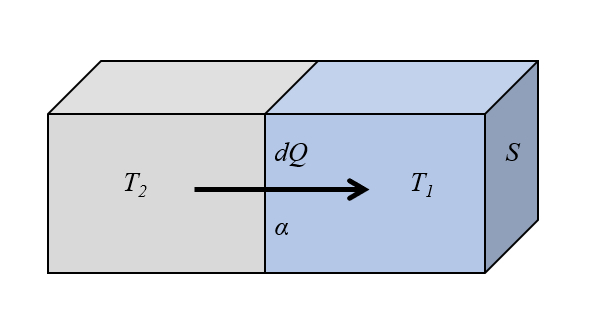
ID:(7723, 0)

Equation

>Top, >Model

If a medium is moving with a constant of the transmission coefficient dependent on the speed ($\alpha_{wv}$), and the mean speed ($v_m$) is equal to

$ \alpha_{wv} = \alpha_{w0} \left(1+\sqrt{\displaystyle\frac{ v_m }{ v_{w0} }}\right)$

Conditions

Variables

$v_{l0}$

Factor velocidad del coefiente de transmisión

0.0278

$m/s$

$v_m$

Mean speed

$m/s$

$\alpha_l$

Transmission coefficient dependent on the speed

$W/m^2K$

$\alpha_{l0}$

Transmission coefficient Independent of the speed

$W/m^2K$

Relation

where the transmission coefficient Independent of the speed ($\alpha_{w0}$) represents the case where the medium is not moving, and the factor velocidad del coefiente de transmisión ($v_{w0}$) is the reference velocity.

The thermal transfer constant of the material for the case of a stationary liquid is equal to $340 J/m^2sK$, while the reference velocity is $0.0278 m/s$.

ID:(7714, 0)

Equation

>Top, >Model

La transferencia del calor en un medio a otro lleva a que una cantidad de calor dQ se desplaza a través de un cuerpo de sección S en un tiempo dt bajo una diferencia de temperatura \Delta T es:

$\displaystyle\frac{ dQ }{ dt }= \alpha S \Delta T $

Conditions

Variables

$dt$

Infinitesimal Variation of Time

$s$

$S$

Section

$m^2$

$\Delta T$

Temperature difference between mediums

$K$

$\alpha$

Transmission coefficient

$W/m^2K$

$\delta Q$

Variation of heat

$J$

Relation

donde \alpha es la constante de transferencia térmica del material.

La constante de transferencia térmica del material es del orden de 350 - 10000 J/m2sK. Este valor depende de la velocidad si uno de los medios es liquido y se desplaza.

ID:(7713, 0)

Equation

>Top, >Model

In the event that a medium moves with a constant value of ($$) and the transmission coefficient in gases, dependent on speed ($\alpha_{gv}$) is equal to

$ \alpha_{gv} = \alpha_{g0} \left(1+\displaystyle\frac{ v_m }{ v_{g0} }\right)$

Conditions

Variables

$v_m$

Mean speed

$m/s$

$v_{g0}$

Transmission coefficient gas velocity factor

$m/s$

$\alpha_g$

Transmission coefficient in gases, dependent on speed

$W/m^2K$

$\alpha_{g0}$

Transmission coefficient in gases, independent of speed

$W/m^2K$

Relation

where the transmission coefficient in gases, independent of speed ($\alpha_{g0}$) represents the scenario where the medium does not move, and the transmission coefficient gas velocity factor ($v_{g0}$) is the reference velocity.

The thermal transfer constant for the material in the case of a stationary gas is equal to $5.6 J/m^2sK$, while the reference velocity is $1.41 m/s$.

ID:(7715, 0)

Image

>Top

The total flow through a material consists of three components: the transfer from the exterior to the medium, conduction through the medium, and the transfer from the medium to the interior, as depicted in the following illustration:

ID:(7721, 0)

Equation

>Top, >Model

In this way, we establish a relationship that allows us to calculate the variation of heat ($dQ$) as a function of the time variation ($dt$), the section ($S$), the total transport coefficient (multiple medium, two interfaces) ($k$), and the temperature difference ($\Delta T$):

$\displaystyle\frac{ dQ }{ dt } = k S \Delta T $

Conditions

Variables

$S$

Section

$m^2$

$\Delta T$

Temperature difference

$K$

$dt$

Time variation

$s$

$k$

Total transport coefficient (multiple medium, two interfaces)

$J/m s K$

$dQ$

Variation of heat

$J$

Relation

Development

With the temperature difference at internal interface ($\Delta T_i$), the temperature difference in the conductor ($\Delta T_0$), the temperature difference at external interface ($\Delta T_e$), and the temperature difference ($\Delta T$), we obtain

$ \Delta T = \Delta T_i + \Delta T_0 + \Delta T_e $

which can be rewritten with the variation of heat ($dQ$), the time variation ($dt$), the section ($S$)

$\displaystyle\frac{ dQ }{ dt } = S \alpha_i \Delta T_i $

$\displaystyle\frac{ dQ }{ dt } = S \alpha_e \Delta T_e $

and with the thermal conductivity ($\lambda$) and the conductor length ($L$)

$\displaystyle\frac{ dQ }{ dt } = \displaystyle\frac{ S }{ L } \lambda \Delta T_0 $

and

$\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_i }+\displaystyle\frac{1}{ \alpha_e }+\displaystyle\frac{ L }{ \lambda }$

as

$\Delta T = \Delta T_i + \Delta T_0 + \Delta T_e = \displaystyle\frac{1}{S} \frac{dQ}{dt} \left(\displaystyle\frac{1}{\alpha_i} + \displaystyle\frac{1}{\alpha_e} + \displaystyle\frac{L}{\lambda}\right) = \displaystyle\frac{1}{Sk} \displaystyle\frac{dQ}{dt}$

resulting in

$\displaystyle\frac{ dQ }{ dt } = k S \Delta T $

ID:(7716, 0)

Equation

>Top, >Model

The transport constant k through a conduction constant means \lambda of a thickness L with transfer coefficients on both edges \alpha_1 and \alpha_2 is the same

$\displaystyle\frac{1}{ k }=\displaystyle\frac{1}{ \alpha_1 }+\displaystyle\frac{1}{ \alpha_2 }+\displaystyle\frac{ L }{ \lambda }$

Conditions

Variables

Relation

ID:(7717, 0)

Equation

>Top, >Model

The temperatures within the conductor medium are represented as:

$ T_{1s} = T_1 +\displaystyle\frac{ k }{ \alpha_1 }( T_2 - T_1 )$

Conditions

Variables

$k$

Constant transportation

$W/m^2K$

$T_2$

Indoor temperature

$K$

$T_{1s}$

Inner surface temperature

$K$

$T_1$

Outdoor temperature

$K$

$\alpha_1$

Transmission coefficient Interface 1

$W/m^2K$

Relation

ID:(3487, 0)

Equation

>Top, >Model

The temperatures within the conductor medium are represented as:

$ T_{2s} = T_2 -\displaystyle\frac{ k }{ \alpha_2 }( T_2 - T_1 ) $

Conditions

Variables

$k$

Constant transportation

$W/m^2K$

$T_2$

Indoor temperature

$K$

$T_1$

Outdoor temperature

$K$

$T_{2s}$

Outer surface temperature

$K$

$\alpha_2$

Transmission coefficient Interface 2

$W/m^2K$

Relation

ID:(3488, 0)

Equation

>Top, >Model

The transport constant $k$ through media with thermal conductivity $\lambda_i$ and thickness $L_i$, respectively, and with transfer coefficients at both boundaries $\alpha_1$ and $\alpha_2$, is determined as follows:

$\displaystyle\frac{1}{k}=\displaystyle\frac{1}{\alpha_1}+\displaystyle\frac{1}{\alpha_2}+\sum_i\displaystyle\frac{L_i}{\lambda_i}$

Conditions

Variables

$L_i$

Element length i

$m$

$\lambda_i$

Thermal conductivity element i

$J/m s K$

$k$

Total transport coefficient (multiple medium, two interfaces)

$J/m s K$

$\alpha_1$

Transmission coefficient Interface 1

$W/m^2K$

$\alpha_2$

Transmission coefficient Interface 2

$W/m^2K$

Relation

ID:(7730, 0)

Image

>Top

Typically, the temperature variation within a conductor follows a linear pattern. However, in the case of gaseous and/or liquid media in contact with the conductor, there is a gradual temperature variation from the center of the medium to the surface, as depicted in the following image:

ID:(7722, 0)

Description

>Top

En el caso de la calefacción crital uno de los sistemas es agua y el otro aire. En el caso del agua los flujos $J$ típicos son del orden de 100 l/h. Si el tubo tiene un radio $R$ de 8 mm esto significa que la velocidad del agua $v$ se puede calcular de

$J=Sv=\pi R^2v$

donde $S$ es la sección del tubo. Por ello en el caso de la calefacción crital la velocidad típica es del orden de 0.13 m/s. Con ello el coeficiente de transmisión del agua-suelo es del orden de 1075 J/m2sK.

En el caso del aire la velocidad es del orden de cero y con ello el coeficiente de transmisión del orden de 5.4 J/m2sK.

Por ultimo el cemento presenta un coeficiente de 2.3 J/msK. Si la distancia entre tubo y superficie es del orden de 1 cm se tiene que el coeficiente de transporte $k$ es del orden de 5.4 J/m2sK.

ID:(7725, 0)

Html

>Top

The heat transport simulator through a wall allows estimating the key parameters and plotting the temperature profile along the system.

There are three ways to use the simulator:

• Calculate the heat transport constant $k$ and the achieved power density $\dot{Q}$ given temperatures, thermal conductivities $\lambda_i$, layer widths $L_i$, and transfer constant $\alpha$. In this case, select the line for the heat transport constant and power density to recalculate and overwrite these values.

• Calculate the width of one of the layers based on its thermal conductivity, along with other geometric and material parameters of the system, and either the heat transport constant $k$ or the power density $\dot{Q}$. In this case, choose the line corresponding to the layer you want to calculate and leave the thermal conductivity field empty.

• Calculate the thermal conductivity of one of the layers based on its width, along with other geometric and material parameters of the system, and either the heat transport constant $k$ or the power density $\dot{Q}$. In this case, choose the line corresponding to the layer you want to calculate and leave the width field empty.

If the external system corresponds to a different material, such as soil instead of air, leave the transfer coefficient field empty.

ID:(7736, 0)